When 101+102+103+.......+101000+101001 is divided by 6, the remainder obtained is ?
4
6
2
3
The correct Answer is:c
To solve the problem of finding the remainder when 101+102+103+…+101000+101001 is divided by 6, we can follow these steps:
Step 1: Understand the expression
The expression we need to evaluate is:
S=101+102+103+…+101001
Step 2: Find the remainder of 10n when divided by 6
First, we need to find the remainder of 10n when divided by 6 for any integer n.
Calculating 10mod6:
10÷6=1(remainder 4)
Thus,
10≡4mod6
Now, we can find 10nmod6:
101≡4mod6
102≡42=16≡4mod6
103≡43=64≡4mod6
Continuing this pattern, we see that:
10n≡4mod6for all n≥1
Step 3: Count the number of terms
The series 101+102+103+…+101001 has a total of:
1001 terms
Step 4: Calculate the sum modulo 6
Since each term 10n contributes a remainder of 4 when divided by 6, the total contribution of all terms is:
S≡4+4+4+…+4(1001 times)
This can be expressed as:
S≡1001×4mod6
Step 5: Calculate 1001×4
Calculating 1001×4:
1001×4=4004
Step 6: Find the remainder of 4004 when divided by 6
Now we need to find 4004mod6:
4004÷6=667(remainder 2)
Thus,
4004≡2mod6
Final Answer
The remainder when 101+102+103+…+101001 is divided by 6 is:
2
The expression we need to evaluate is:
S=101+102+103+…+101001
First, we need to find the remainder of 10n when divided by 6 for any integer n.
10÷6=1(remainder 4)
Thus,
10≡4mod6
101≡4mod6
102≡42=16≡4mod6
103≡43=64≡4mod6
Continuing this pattern, we see that:
10n≡4mod6for all n≥1
The series 101+102+103+…+101001 has a total of:
1001 terms
Since each term 10n contributes a remainder of 4 when divided by 6, the total contribution of all terms is:
S≡4+4+4+…+4(1001 times)
This can be expressed as:
S≡1001×4mod6
Calculating 1001×4:
1001×4=4004
Now we need to find 4004mod6:
4004÷6=667(remainder 2)
Thus,
4004≡2mod6
The remainder when 101+102+103+…+101001 is divided by 6 is:
2
Topper's Solved these Questions
Similar Questions
Knowledge Check
When
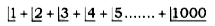 is divided by 10, the remaindeer obtained is ? A3B1C2D9
is divided by 10, the remaindeer obtained is ? A3B1C2D9When 101+102+103+........+1099+10100 is divided by 6, the remainder obtained is
A2B4C0D1When 101+102+103+.......+1032 is divided by 6, the remainder obtained is ?
A4B5C2D1
When 233 is divided by 10, the remainder will be
View SolutionWhen number 101+102+103+104+.............+1011 is divided by 6, the remain- der will be?
View SolutionWhen 252126+244152 is divided by 10, the remainder will be?
View SolutionWhen x3+5x2+10k is divided by (x2+2) the remainder obtained is −2x, then the value of k is
View SolutionWhen number 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 is divided by 16, the remainder will be?
View Solution
