Let u=ax+by+a3√b=0,v=bx−ay+b3√a=0,a,b∈R, be two straight lines. The equations of the bisectors of the angle formed by k1u−k2v=0 and k1u+k2v=0 , for nonzero and real k1 and k2 are Solution in Bengali
u=0
k2u+k1v=0
k2u−k1v=0
v=0
The correct Answer is:A,D
Note that the lines are perpendicular, Assume the coordinates axes to be directed along u=0 and v = 0, Now, the lines k1u−k2v=0 and k1u+k2v=0 are equally inclined with the u-v axes. Hence, the bisectors are u=0 and v=0.
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (LINKED COMPREHENSION TYPE)
27 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MATRIX MATCH TYPE)
8 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (SINGLE CORRECT ANSWER TYPE)
82 Videos
- STRAIGHT LINEBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )
1 Videos
Similar Questions
Let l be the line belonging to the family of straight lines (a+2b)x+(a−3b)y+a−8b=0,a,b∈R, which is farthest from the point (2,2), then area enclosed by the line L and the coordinate axes is
View SolutionMatch the following lists:
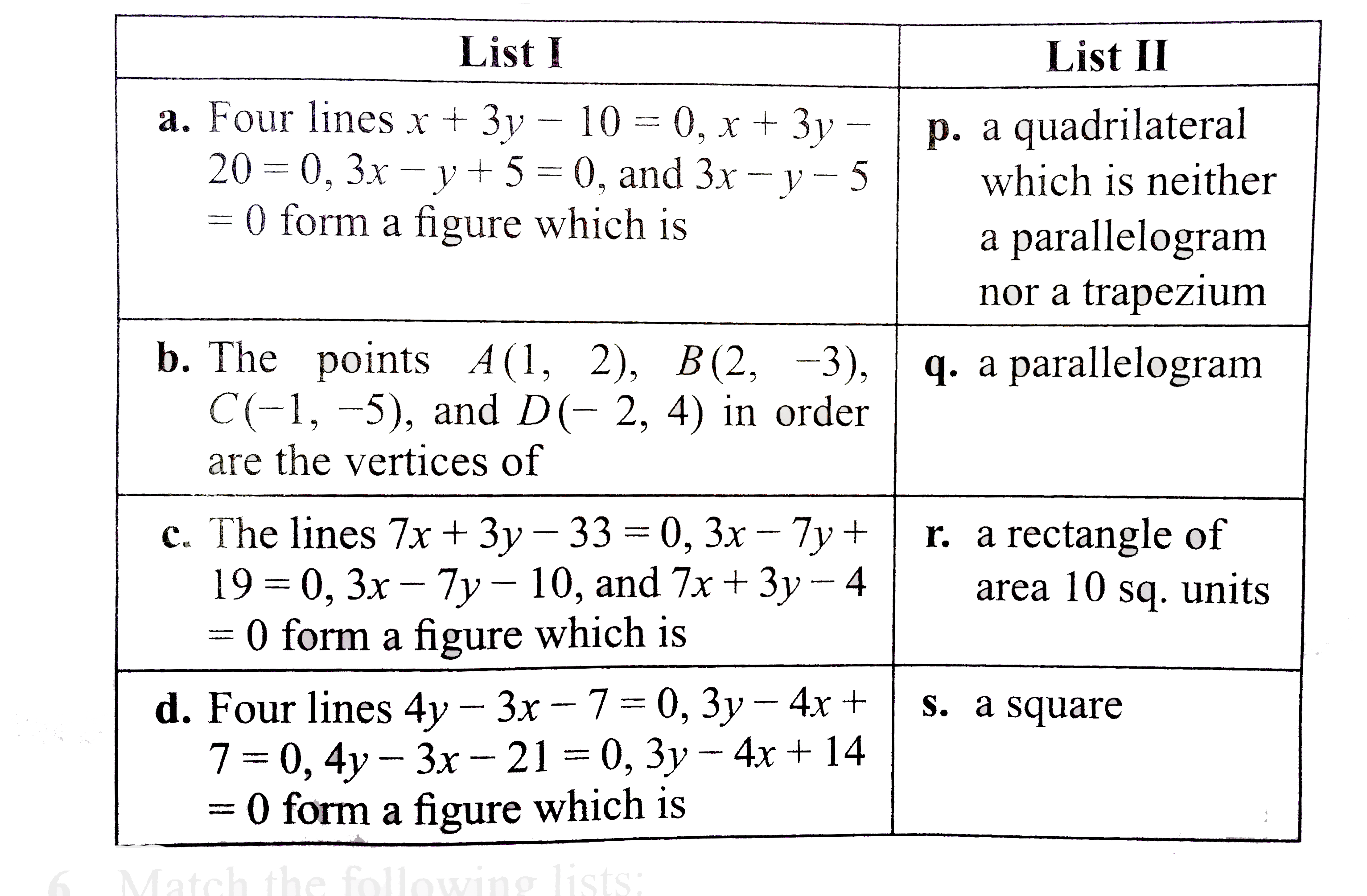
View SolutionFind the equations of the diagonals of the square formed by the lines x=o,y=0,x=1andy=1.
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
View SolutionLet u≡ax+by+ab3=0,v≡bx−ay+ba3=0,a,b∈R, be two straight lines. The equations of the bisectors of the angle formed by k1u−k2v=0 and k1u+k2v=0 , for nonzero and real k1 and k2 are u=0 (b) k2u+k1v=0 k2u−k1v=0 (d) v=0
View SolutionIf x+y=0 is the angle bisector of the angle containing the point (1,0), for the line 3x+4y+b=0;4x+3y+b=0,4x+3y-,b=0 then
View SolutionTriangle formed by variable lines (a+b)x+(a-b)y-2ab=0 and (a-b)x+(a+b)y-2ab=0 and x+y=0 is (where a, b∈R)
View SolutionIf a,b,x,y are real and 3√x+iy=a+ib show that , 3√x−iy=a−ib xa+yb=4(a2−b2).
View SolutionLet A(-1, 0), B(0, 0) and C(3,3√3) be three points. Then the bisector of ∠ABC is :
View SolutionLet u≡ax+by+ab3=0,v≡bx−ay+ba3=0,a,b∈R, be two straight lines. The equations of the bisectors of the angle formed by k1u−k2v=0 and k1u+k2v=0 , for nonzero and real k1 and k2 are u=0 (b) k2u+k1v=0 k2u−k1v=0 (d) v=0
View SolutionLet ABC be a triangle. Let A be the point (1,2),y=x be the perpendicular bisector of AB, and x−2y+1=0 be the angle bisector of ∠C . If the equation of BC is given by ax+by−5=0 , then the value of a+b is (a)1(b) 2(c) 3 (d) 4
View Solution. The lines (a+b)x + (a-b) y-2ab=0, (a-b)x+(a+b)y-2ab = 0 and x+y=0 form an isosceles triangle whose vertical angle is
View SolutionA circle with center (a,b) passes through the origin. The equation of the tangent to the circle at the origin is (a)ax−by=0 (b) ax+by=0 bx−ay=0 (d) bx+ay=0
View SolutionTriangle formed by variable lines (a+b)x+(a-b)y-2ab=0 and (a-b)x+(a+b)y-2ab=0 and x+y=0 is (where a, b∈R)
View SolutionLet u≡ax+by+ab3=0,v≡bx−ay+ba3=0,a,b∈R, be two straight lines. The equations of the bisectors of the angle formed by k1u−k2v=0 and k1u+k2v=0 , for nonzero and real k1 and k2 are
View Solution
