Find the equation of a straight line on which the perpendicular from the origin makes an angle of 300 with x−aξs and which forms a triangle of area 50√3 with the axes.Solution in Bengali
√3x+y−10=0
√3x+y+10=0
x+√3y−10=0
x−√3y−10=0
The correct Answer is:A, B
Let p be the length of the perpendicular from the origin on the given line. Then its equation in normal form is
xcos 30∘+ysin 30∘=p
or √3x+y=2p
This meets the coordintates axes at A(2p/√3,0) and B(0, 2p).
Therefore, the area of ΔAOB is
12(2p√3)2p=2p2√3
By hypothesis,
2p2√3=50√3or p=±5
Hence, the line are √3x+y±10=0.
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (LINKED COMPREHENSION TYPE)
27 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MATRIX MATCH TYPE)
8 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (SINGLE CORRECT ANSWER TYPE)
82 Videos
- STRAIGHT LINEBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )
1 Videos
Similar Questions
Let l be the line belonging to the family of straight lines (a+2b)x+(a−3b)y+a−8b=0,a,b∈R, which is farthest from the point (2,2), then area enclosed by the line L and the coordinate axes is
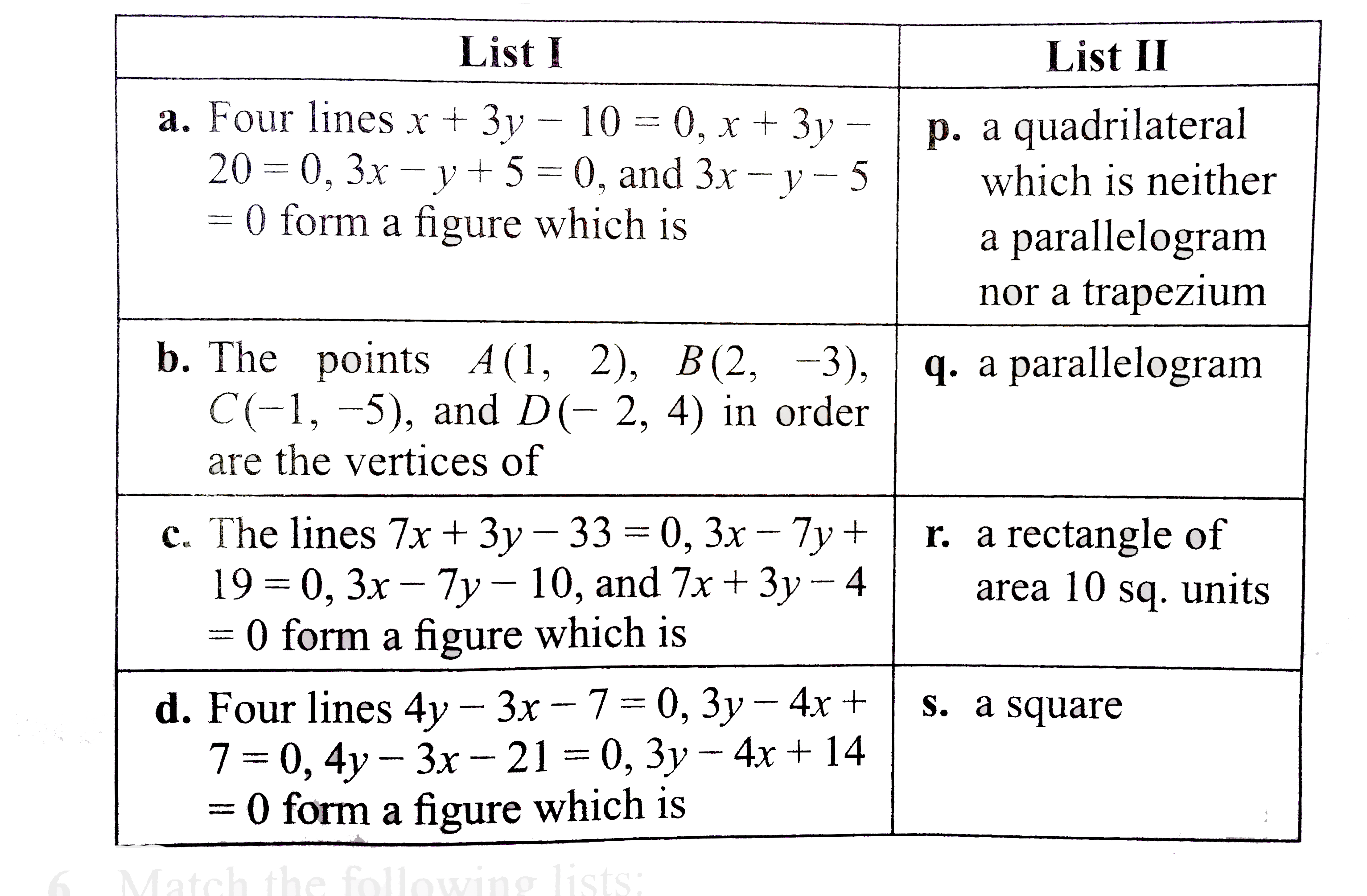
View SolutionMatch the following lists:
View SolutionFind the equations of the diagonals of the square formed by the lines x=o,y=0,x=1andy=1.
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
View SolutionFind the equation of a straight line on which the perpendicular from the origin makes an angle of 300 with x-axis and which forms a triangle of area 50√3 with the axes.
View SolutionFind the equation of the straight line on which the perpendicular from origin makes an angle 30^(@) with positive x-axis and which foms a triangle of area (50)/(sqrt(3))sq, units with the co- ordinates axis.
View SolutionThe equation of the line on which the perpendicular from the origin makes an angle of 30^(@) with x -axis and which forms a triangle of area (50)/(sqrt(3)) with the axes is
View SolutionFind the equation of the straight line which makes a triangle of area 96√3 with the axes and perpendicular from the origin to it makes an angle of 300 with y-axis.
View SolutionFind the equation of a line which makes a triangle of area 96√3 square from co-ordinate axes and the perpendicular drawn from origin to this line makes an angle 60∘ from X-axis.
View Solutionउस सरल रेखा का समीकरण ज्ञात कीजिए जो अक्षों के साथ ऐसा त्रिभुज बनाती है, जिसका क्षेत्रफल 96√3 वर्ग इकाई है तथा इस पर मूल बिंदु से डाला गया लम्ब y-अक्ष से 30∘ का कोण बनाता है।
View Solutionउन रेखाओं का समीकरण जिन पर मूलबिंदु से डाला गया लम्ब x अक्षों के साथ 30∘ का कोण बनाता है ओर उनसे तथा x अक्ष से बने त्रिभुज का क्षेत्रफल 50√3 वर्ग इकाई हो, तब -
View Solutionउस सरल रेखा का समीकरण ज्ञात कीजिये जिस पर मूलबिंदु से डाले गये लंब की लम्बाई 4 इकाई है तथा यह लंब X - अक्ष से 30∘ का कोण बनाता है ।
View SolutionA line forms a triangle of area 54√3square units with the coordinate axes. Find the equation of the line if the perpendicular drawn from the origin to the line makes an angle of 600 with the X-axis.
View SolutionFind the equation of a straight line on which the perpendicular from the origin makes an angle of 300 with x-axis and which forms a triangle of area 50√3 with the axes.
View SolutionFind the equation of line which makes a triangle with area 50√3 unit with axis and perpendicular from origin makes an angle π6 with positive side of X-axis.
View Solution
