θ1andθ2 are the inclination of lines L1andL2 with the x-axis. If L1andL2 pass through P(x1,y1), then the equation of one of the angle bisector of these lines is Solution in Bengali
x−x1cos(θ1+θ22)=y−y1sin(θ1+θ22)
x−x1-sin(θ1−θ22)=y−y1cos(θ1−θ22)
x−x1sin(θ1+θ22)=y−y1cos(θ1+θ22)
x−x1-sin(θ1+θ22)=y−y1cos(θ1+θ22)
The correct Answer is:A, D
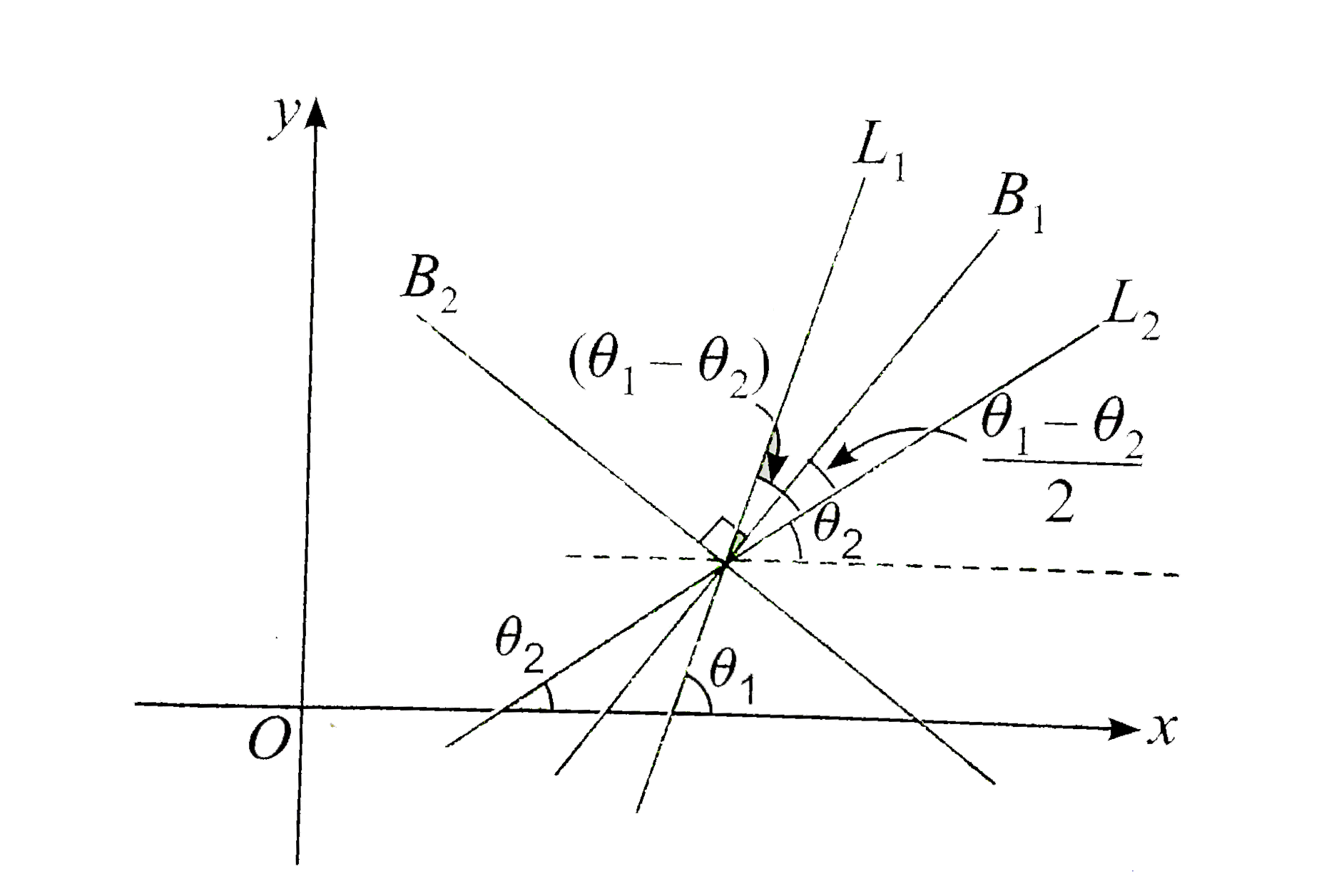
From the figure, angle bisectors B1 and B2 have inclinations of
θ1+θ22 and (π2+θ1+θ22) with the x-axis.
Therefore, equations of angle bisectors are
x−x1cos(θ1+θ22)=y−y1sin(θ1+θ22)
and x−x1cos(π2+θ1+θ22)=y−y1sin(π2+θ1+θ22)
or x−x1-sin(θ1+θ22)=y−y1cos(θ1+θ22)
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (LINKED COMPREHENSION TYPE)
27 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MATRIX MATCH TYPE)
8 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (SINGLE CORRECT ANSWER TYPE)
82 Videos
- STRAIGHT LINEBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )
1 Videos
Similar Questions
Let l be the line belonging to the family of straight lines (a+2b)x+(a−3b)y+a−8b=0,a,b∈R, which is farthest from the point (2,2), then area enclosed by the line L and the coordinate axes is
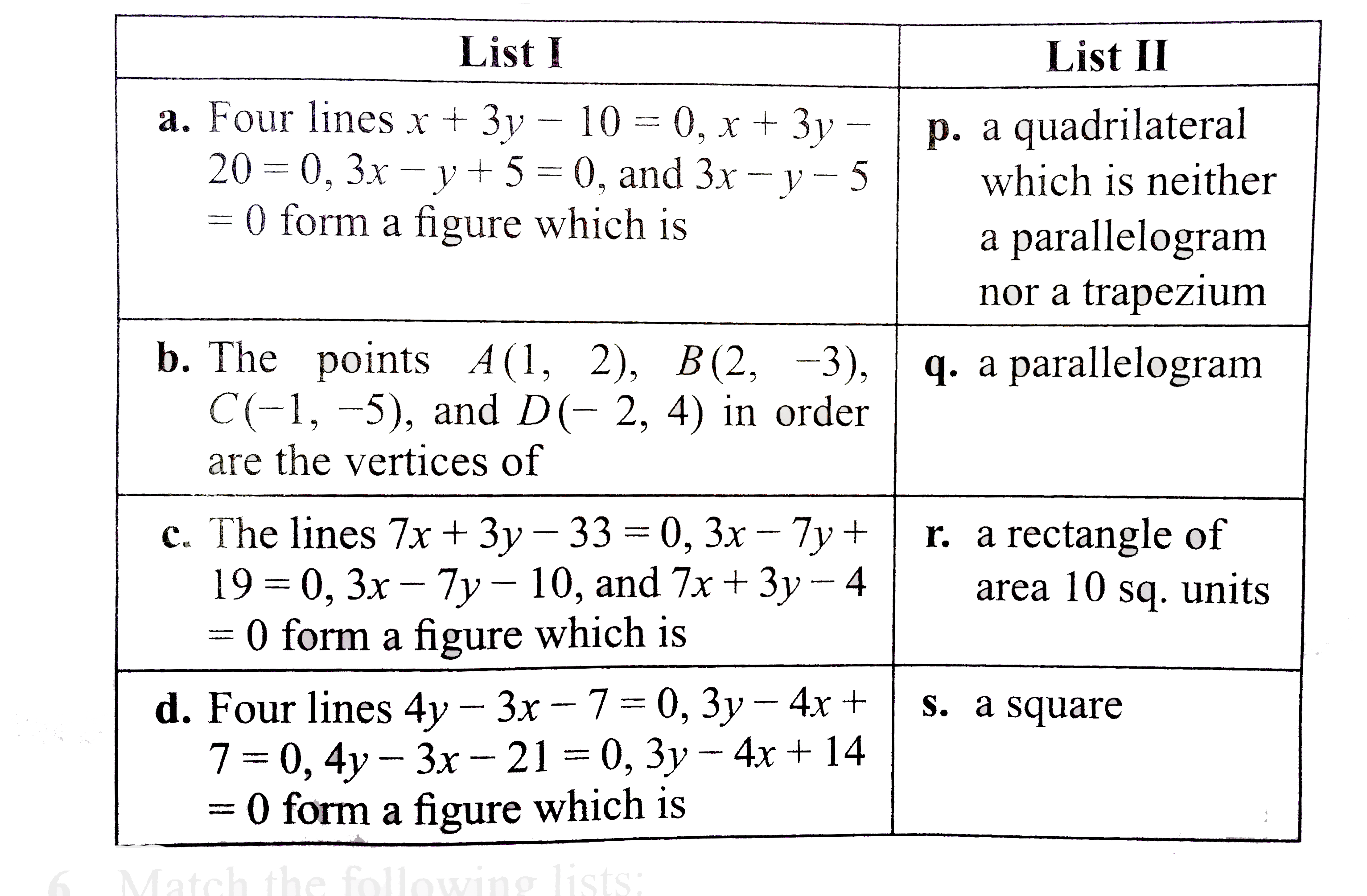
View SolutionMatch the following lists:
View SolutionFind the equations of the diagonals of the square formed by the lines x=o,y=0,x=1andy=1.
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
View Solutionθ1 and θ2 are the inclination of lines L1andL2 with the x-axis. If L1andL2 pass through P(x1,y1) , then the equation of one of the angle bisector of these lines is (a) x−x1cos(θ1−θ22)=y−y1sin(θ1−θ22) (b)x−x1−sin(θ1−θ22)=y−y1cos(θ1−θ22) (c)x−x1sin(θ1−θ22)=y−y1cos(θ1−θ22) (d)x−x1−sin(θ1−θ22)=y−y1cos(θ1−θ22)
View SolutionThe vector equations of two lines L1andL2 are respectively, L1:r=2i+9j+13k+λ(i+2j+3k)andL2:r=−3i+7j+pk+μ(−i+2j−3k) Then, the lines L1andL2 are
View SolutionIf line l1 is perpendicular to line l2 and line l3 has slope 3 then find the equation of line l1
View SolutionLet L1 be a tangent to the parabola y2=4(x+1)andL2 be a tangent to the parabola y2=8(x+2) such that L1andL2 intersect at right angles. Then L1andL2 meet on the straight line :
View Solutionθ1 and θ2 are the inclination of lines L1andL2 with the x-axis. If L1andL2 pass through P(x1,y1) , then the equation of one of the angle bisector of these lines is
(a) x−x1cos(θ1−θ22)=y−y1sin(θ1−θ22) (b)x−x1−sin(θ1−θ22)=y−y1cos(θ1−θ22) (c)x−x1sin(θ1−θ22)=y−y1cos(θ1−θ22) (d)x−x1−sin(θ1+θ22)=y−y1cos(θ1+θ22)
View SolutionThe equations of bisectors of two lines L1&L2 are 2x−16y−5=0 and 64x+8y+35=0. lf the line L1 passes through (−11,4), the equation of acute angle bisector of L1 & L2 is:
View Solutionθ1andθ2 are the inclination of lines L1andL2 with the x-axis. If L1andL2 pass through P(x1,y1), then the equation of one of the angle bisector of these lines is
View SolutionShow that the relation R defined in the set A of all lines as R={(L1,L2):L1andL2 are parallel lines} is an equivalence relation.
View SolutionL1andL2 are two intersecting lines. If the image of L1 w.r.t. L2 and that of L2 w.r.t. L1 concide, then the angle between L1andL2 is
View SolutionThe straight line L≡X+Y+1=0andL1≡X+2Y+3=0 are intersectiong, m is the slope of the straight line L2 such that L is the bisector of the angle between L1andL2 The value of m2 is .
View SolutionThe vector equations of two lines L1andL2 are respectively, L1:r=2i+9j+13k+λ(i+2j+3k)andL2:r=−3i+7j+pk+μ(−i+2j−3k) Then, the lines L1andL2 are
View Solution
