Consider the equation y−y1=m(x−x1) . If mandx1 are fixed and different lines are drawn for different values of y1, then (a) the lines will pass through a fixed point (b) there will be a set of parallel lines (c) all the lines intersect the line x=x1 (d)all the lines will be parallel to the line y=x1Solution in Bengali
the lines will pass through a fixed point
there will be a set of parallel lines
all the lines intersect the line x=x1
all the lines will be parallel to the line y=x1
The correct Answer is:B, C
Draw the diagram and verify.
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (LINKED COMPREHENSION TYPE)
27 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MATRIX MATCH TYPE)
8 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (SINGLE CORRECT ANSWER TYPE)
82 Videos
- STRAIGHT LINEBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )
1 Videos
Similar Questions
Let l be the line belonging to the family of straight lines (a+2b)x+(a−3b)y+a−8b=0,a,b∈R, which is farthest from the point (2,2), then area enclosed by the line L and the coordinate axes is
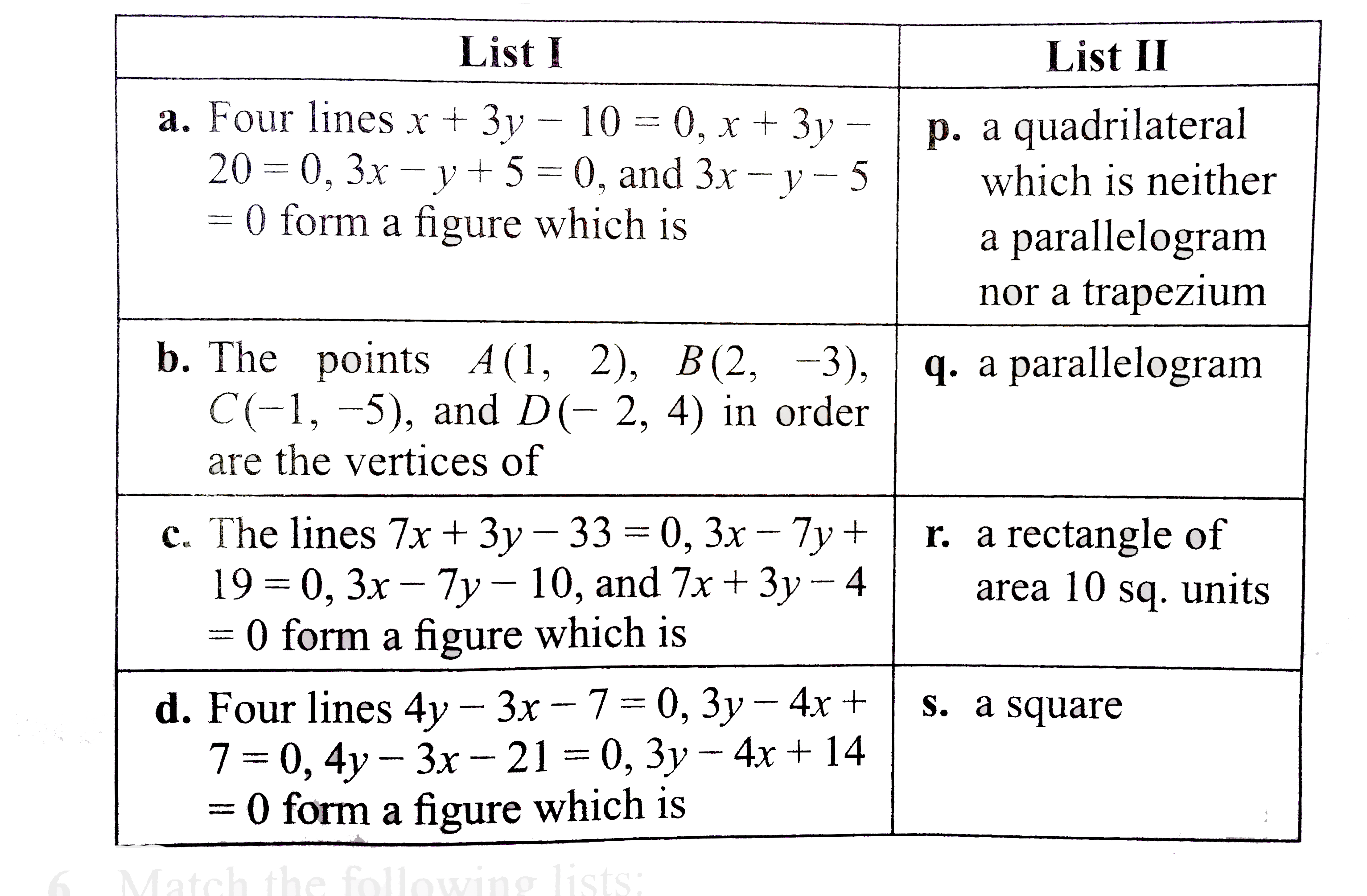
View SolutionMatch the following lists:
View SolutionFind the equations of the diagonals of the square formed by the lines x=o,y=0,x=1andy=1.
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
View SolutionProve that the line through the point x1,y1) and parallel to the line Ax+By+C=0 is A(x−x1)+B(y−y1)=0.
View SolutionProw that the line through the point (x1,y1)and parallel to the line Ax+By+C=0is A(x−x1)+B(y−y1)=0.
View SolutionA line passes through (x1,y1)and (h,k). If slope of the line is m, show that k−y1=m(h−x1).
View SolutionConsider the equation y−y1=m(x−x1) . If mandx1 are fixed and different lines are drawn for different values of y1, then
View SolutionConsider the equation y−y1=m(x−x1) . If mandx1 are fixed and different lines are drawn for different values of y1, then (a) the lines will pass through a fixed point (b) there will be a set of parallel lines (c) all the lines intersect the line x=x1 (d)all the lines will be parallel to the line y=x1
View SolutionProve that the line through the point (x1,y1) and parallel to the line Ax+By+C=0 is A(x−x1)+B(y−y1)=0.
View SolutionA line passes through (x1,y1) and (h, k). If slope of line is m, show that k−y1=m(h−x1).
View SolutionProve that the line through the point (x1,y1) and parallel to the line Ax+By+C=0 is A(x−x1)+B(y−y1)=0.
View SolutionA line passes through (x1,y1) and (h,k). if the slope of the line is m, show that k−y1=m(h−x1)
View SolutionProve that the line through the point (x1,y1) and parallel to the line Ax + By + C = 0 is A(x−x1)+B(y−y1)=0
View Solutionਇੱਕ ਰੇਖਾ (x1,y1) ਅਤੇ (h, k) ਵਿੱਚੋਂ ਲੰਘਦੀ ਹੈ। ਜੇਕਰ ਰੇਖਾ ਦੀ ਢਲਾਣ m ਹੈ ਤਾਂ ਦਿਖਾਉ ਕਿ k−y1=m(h−x1)
View Solution
