If the straight line ax+cy=2b, where a,b,c>0, makes a triangle of area 2 sq. units with the coordinate axes, then (a) a,b,c are in GP (b) a, -b, c are in GP (c) a,2b,c are in GP (d) a,−2b,c are in GPSolution in Bengali
a,b,c are in GP
a,-b, c are in GP
a,2b,c are in GP
a,-2b, c are in GP
The correct Answer is:A, B
The area of the triangle is given by
12×2ba×2bc=2b2ac=2
or b2=ac
Therefore, a,b,c are in GP. So, a,-b, c are alos in GP.
Topper's Solved these Questions
- STRAIGHT LINESBook:CENGAGE PUBLICATIONChapter:STRAIGHT LINESExercise:EXERCISE (LINKED COMPREHENSION TYPE)
Explore 27 Videos
- STRAIGHT LINESBook:CENGAGE PUBLICATIONChapter:STRAIGHT LINESExercise:EXERCISE (MATRIX MATCH TYPE)
Explore 8 Videos
- STRAIGHT LINESBook:CENGAGE PUBLICATIONChapter:STRAIGHT LINESExercise:EXERCISE (SINGLE CORRECT ANSWER TYPE)
Explore 82 Videos
- STRAIGHT LINEBook:CENGAGE PUBLICATIONChapter:STRAIGHT LINEExercise:Multiple Correct Answers Type
Explore 8 Videos
- THEORY OF EQUATIONSBook:CENGAGE PUBLICATIONChapter:THEORY OF EQUATIONSExercise:JEE ADVANCED (Numerical Value Type )
Explore 1 Video
Similar Questions
Let l be the line belonging to the family of straight lines (a+2b)x+(a−3b)y+a−8b=0,a,b∈R, which is farthest from the point (2,2), then area enclosed by the line L and the coordinate axes is
View SolutionMatch the following lists:
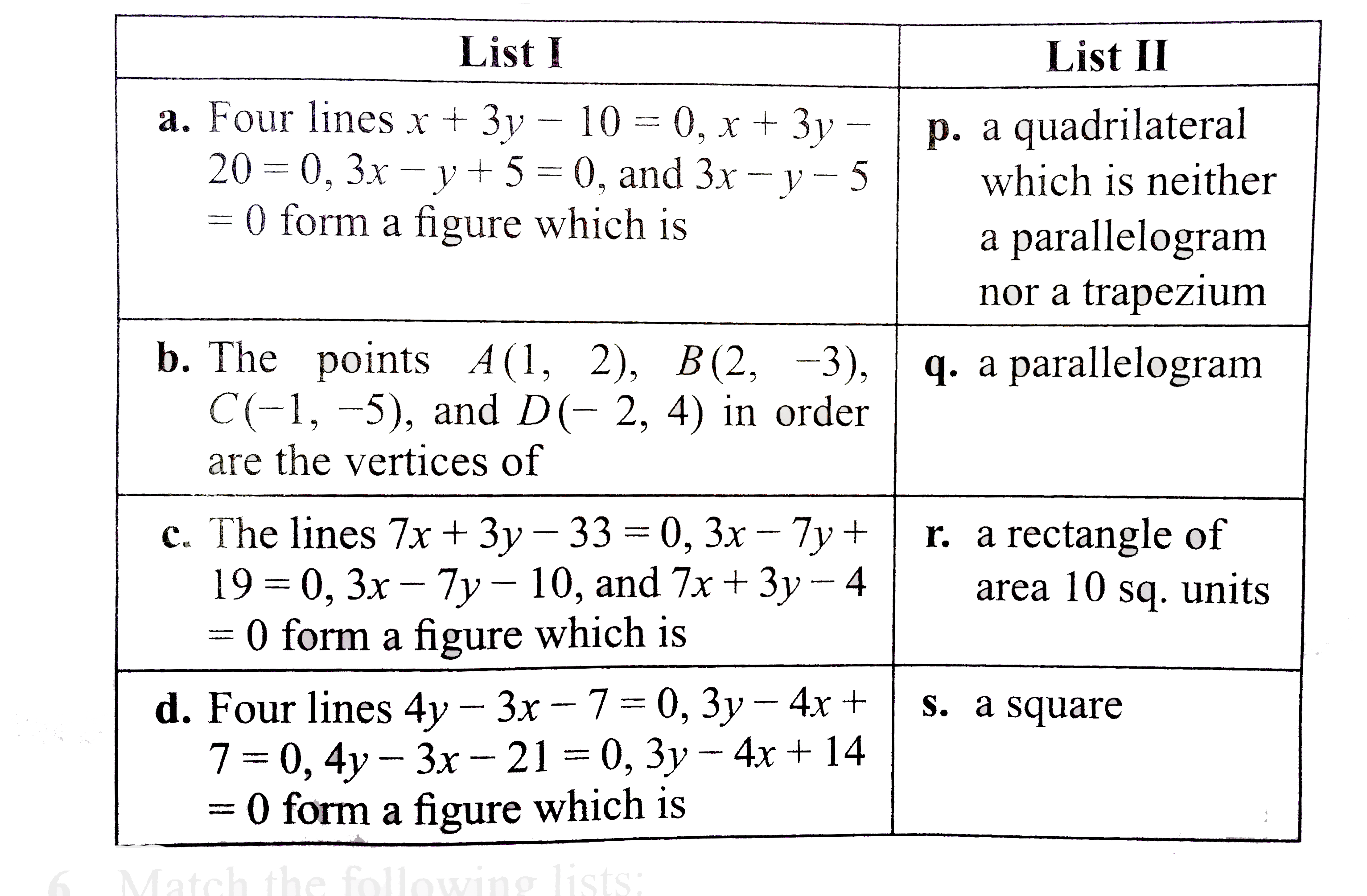
View SolutionFind the equations of the diagonals of the square formed by the lines x=o,y=0,x=1andy=1.
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
View SolutionIf the straight line ax+cy=2b, where a,b,c>0, makes a triangle of area 2 sq. units with the coordinate axes, then (a)a,b,c are in GP (b)a, -b; c are in GP (c)a,2b,c are in GP (d) a,−2b,c are in GP
View SolutionIf a,b,c,d are in GP, then (b+c)2= ______.
View SolutionIf a, b, c are in GP, prove that 1(a+b),12b,1b+c are in AP.
View SolutionIf a, b, c are in GP, prove that a2,b2,c2 are in GP.
View SolutionIf a, b, c are in GP, prove that a3,b3,c3 are in GP.
View SolutionSuppose α,β are roots of ax2+bx+c=0 and γ,δ are roots of Ax2+Bx+C=0.
If a,b,c are in GP as well as α,β,γ,δ are in GP, then A,B,C are in
View SolutionIf the straight line ax+cy=2b, where a,b,c>0, makes a triangle of area 2 sq. units with the coordinate axes, then (a)a,b,c are in GP (b)a, -b; c are in GP (c)a,2b,c are in GP (d) a,−2b,c are in GP
View SolutionIf the straight line ax+cy=2b, where a,b,c>0, makes a triangle of area 2 sq. units with the coordinate axes, then (a) a,b,c are in GP (b) a, -b, c are in GP (c) a,2b,c are in GP (d) a,−2b,c are in GP
View SolutionIf a,b,c,d are in GP then prove that a+b,b+c,c+d are in GP.
View SolutionIf the straight line ax+cy=2b, where a,b,c>0, makes a triangle of area 2 sq. units with the coordinate axes, then
View Solutiona,b,c are sides of a triangle and a,b,c are in GP If loga−log2b,log2b−log3candlog3c−loga are in AP then
View Solution
