Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
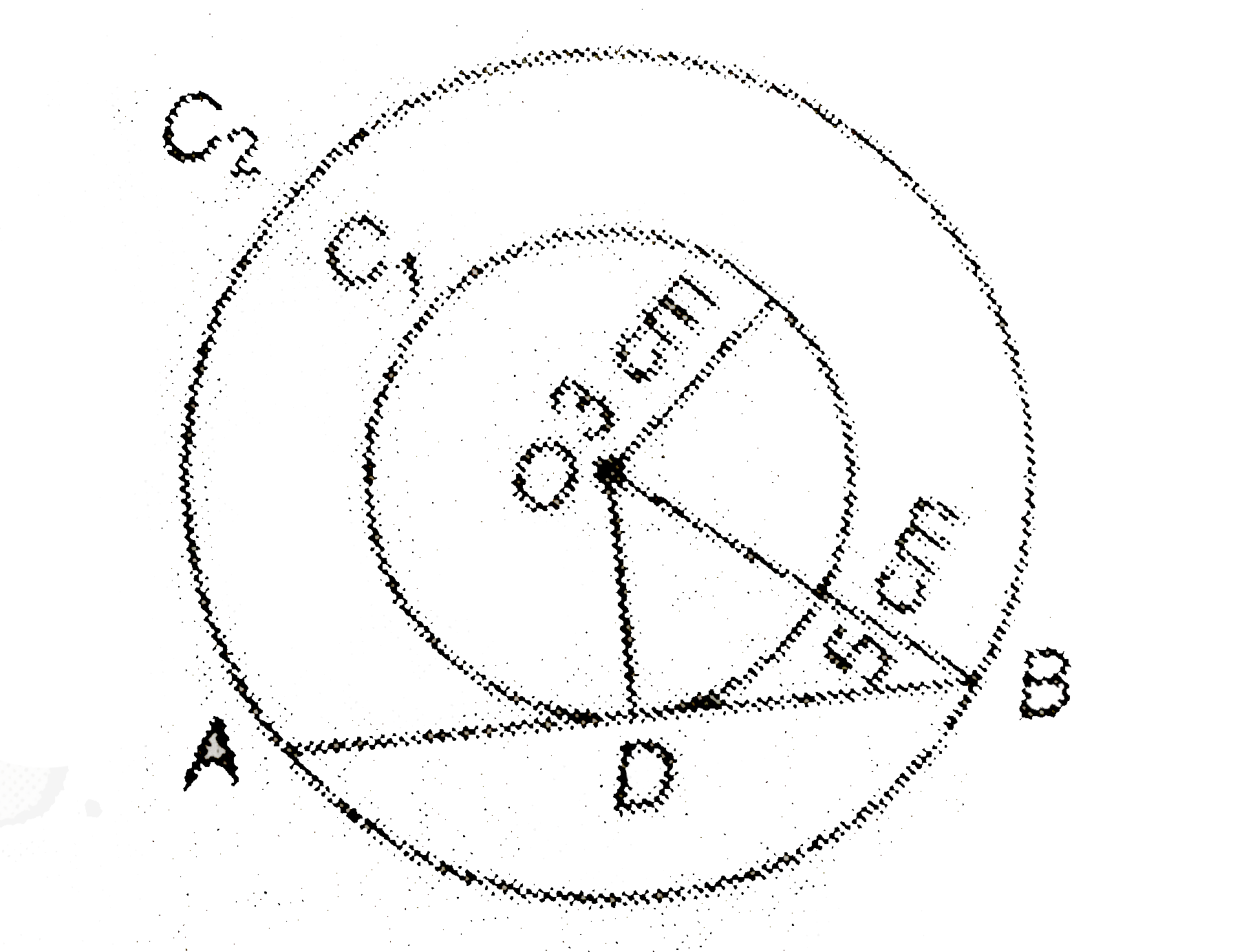
Here, we draw two circles C1 and C2 of radii r1=3cm and r2=5cm Now, we draw a chord AB such that it touches the circle C1 at point D. The centre of concentric circle is O.
Now, we draw a perpendicular bisector from O to AB which meets AB at D.
i.e., AD=BD
In right △OBD
OB2=OD2+DB2 (by Pythagoras theorem)
⇒ 52=32+DB2
DB2=25−9=16
⇒ DB=4cm
Length of chord =AB=2BD=2×4=8cm
Topper's Solved these Questions
- CIRCLESBook:NAGEEN PRAKASHAN ENGLISHChapter:CIRCLESExercise:Exercise
Explore 32 Videos
- CIRCLESBook:NAGEEN PRAKASHAN ENGLISHChapter:CIRCLESExercise:Revision Exercise Very Short Answer Question
Explore 7 Videos
- CIRCLESBook:NAGEEN PRAKASHAN ENGLISHChapter:CIRCLESExercise:Long Answer Questions
Explore 2 Videos
- ARITHMETIC PROGRESSIONBook:NAGEEN PRAKASHAN ENGLISHChapter:ARITHMETIC PROGRESSIONExercise:Revision Exercise Long Answer Question
Explore 5 Videos
- CO-ORDINATE GEOMETRYBook:NAGEEN PRAKASHAN ENGLISHChapter:CO-ORDINATE GEOMETRYExercise:Revision Exercise Short Answer Questions
Explore 10 Videos
Similar Questions
Two concentric circles are of radii 5 cm. and 3 c. Find the length of the chord of the larger circle which touches the cmaller circle.
View SolutionTwo concentric circles are of diameters 30cm and 18cm. Find the length of the chord of the larger circle which touches the smaller circle.
View SolutionTwo concentric circles are of radii 13 cm and 5 cm. Find the length of the chord of the larger circle which touches the inner circle.
View SolutionIf the radii of two concentric circles are 4 cm and 5 cm, then find the length of each chord of one circle which is tangent to the other circle.
View SolutionTwo concentric circle of radii 3cm and 5cm are given. Find the chord BC which touches the inner circle at P.
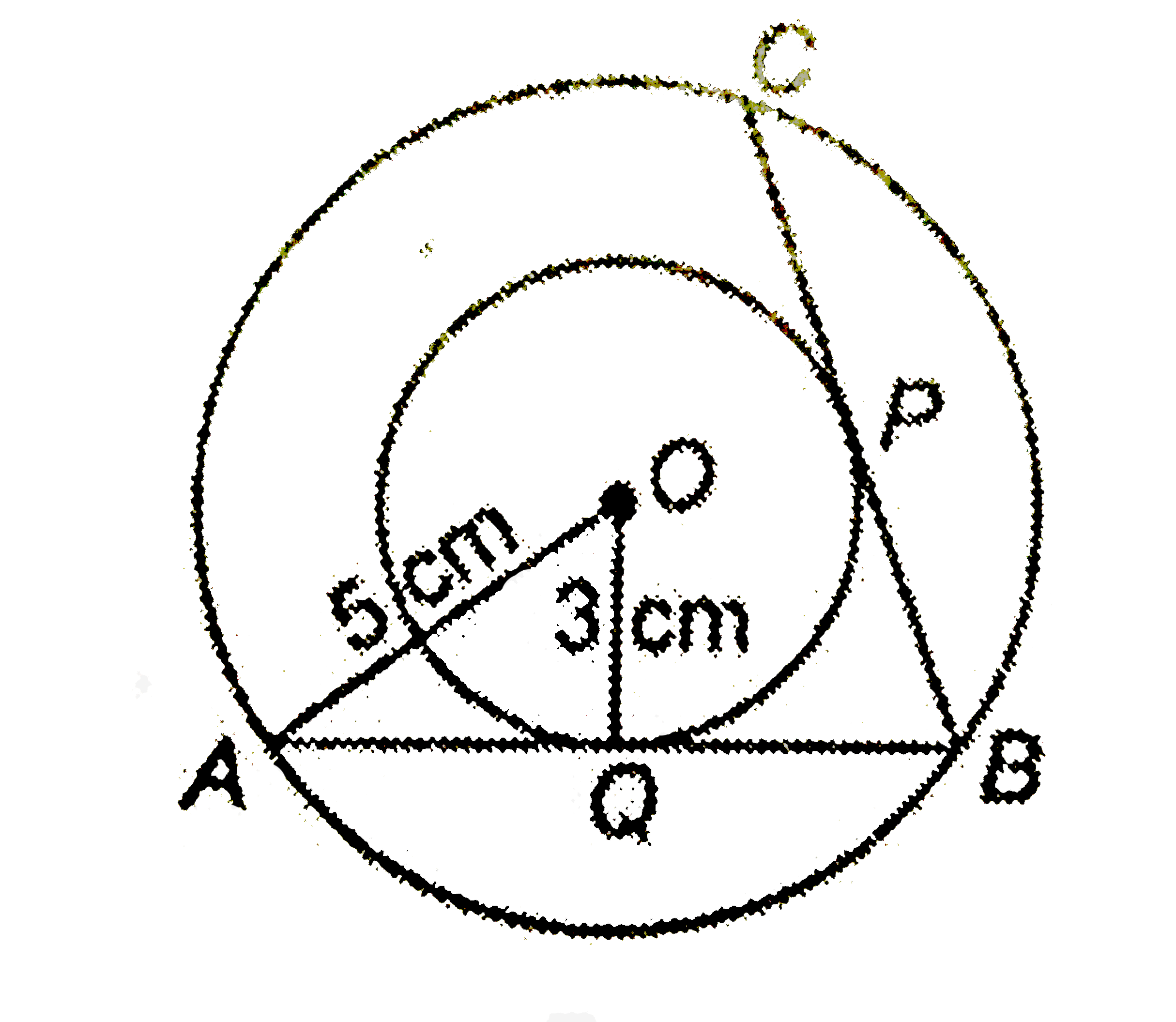
View SolutionTwo concentric circles of radii 3cmand5cm are given. Then length of chord BC which touches the inner circle at P is equal to (a) 4 cm (b) 6cm (c) 8 cm (d) 10 cm
View SolutionIf radii of two concentric circles are 4 cm and 5 cm, then length of each chord of one circle which is tangent to the other circle, is
View SolutionIf radii of the two concentric circles are 15cm and 17cm, then the length of each chord of one circle which is tangent to other is: 8cm (b) 16cm (c) 30cm (d) 17cm
View SolutionIf radii of the two concentric circles are 15cm and 17cm, then the length of each chord of one circle which is tangent to other is: 8cm (b) 16cm (c) 30cm (d) 17cm
View SolutionProve that in two concentric circles, the chord of the larger circle, which touches the smaller circle, is bisected at the point of contact.
View Solution