A nonconducting disk of radius a and uniform positive surface charge density σ is placed on the ground, with its axis vertical. A particle of mass m and positive charge q is dropped, along the axis of the disk, from a height H with zero initial velocity. The particle has q/m=4ε0g/σ.
(i) Find the value of H if the particle just reaches the disk.
(ii) Sketch the potential energy of the particle as a function of its height and find its equilibrium position.
The correct Answer is:4
Potential at a height H on the axis of the disc V(P). The charge dq contained on the ring shown in figure dq=(2πrdr)σ
Potential at P due to this ring
dv=14πε0dpx where x=√H2+x2
∴ Potential due to the complete disc Vp=∫x=2x=0dv=σ2ε0∫r=ar=0rdr√H2+r2
Vp=σ2eπs0[√a2+H2−H]
Potential centre (O) will be
(i) Particle is released from P and it just reaches point O. therefore, from conservation of mechanical energy Decrease in gravitational potential energy = Increase in electrostatic potential energy
(ΔKE=0becauseki=kf=0)∴mgH=q[Vq−Vp]
orgH=(qm)(simga2eπs0[a−√a2+H2+H.....(i)
Subsituting Eq. (i) We get
gH=2g[a+H−√a2+H2 or H2=(a+H)−√a2+H2 or √a2+H2 =a+H2
ora2+H2=a2++H24=aor34HorH−=43a&H=0∴H=(4/3) Potential energy of the particle at height electrostatic potential energy + gravitational potential
energy ∴ U=qV+mqH: Here V= Potenial at height H
U=σq2ε0[√a2+H2−H]+mgH ...(ii)
At equilibrium position F=−dUdH=0, Differentitaing Eq . (ii) w.r.tH
∴mg+2mg[H√a2+H2−1=0 or 1+2H√a2+H2−2=0
or2H√a2+H2−1orH2a2+H2=14
or 3H2−a2
orH=2√3
From eq (ii) , we can write
U - E equations as
U=mg(2√a2+H2−H)
(Paabolic variation)
Therefore, graph will be as shown
Note that at H−a√3U is minimum
Therefore H=a√3 U is is stable equilibrium position
Topper's Solved these Questions
- ELECTROSTATICSBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE MAIN
65 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT EXERCISE - IMPECCABLE
52 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - JEE ADVANCE (ARCHIVE) - TRUE/FALSE TYPE
1 Video
Similar Questions
A uniform cube of mass m and edge a moves on a horizontal surface along the positive x-axis, with initial velocity v0. Then
View SolutionA particle of mass m and charge q is thrown in a region where uniform gravitational field and electric field are present. The path of particle:
View SolutionA smooth chute is made in a dielectric sphere of radius R and uniform volume charge density. ρ. A charge particle of mass m and charge -q is placed at the centre of the sphere. Find the time period of motion of the particle?

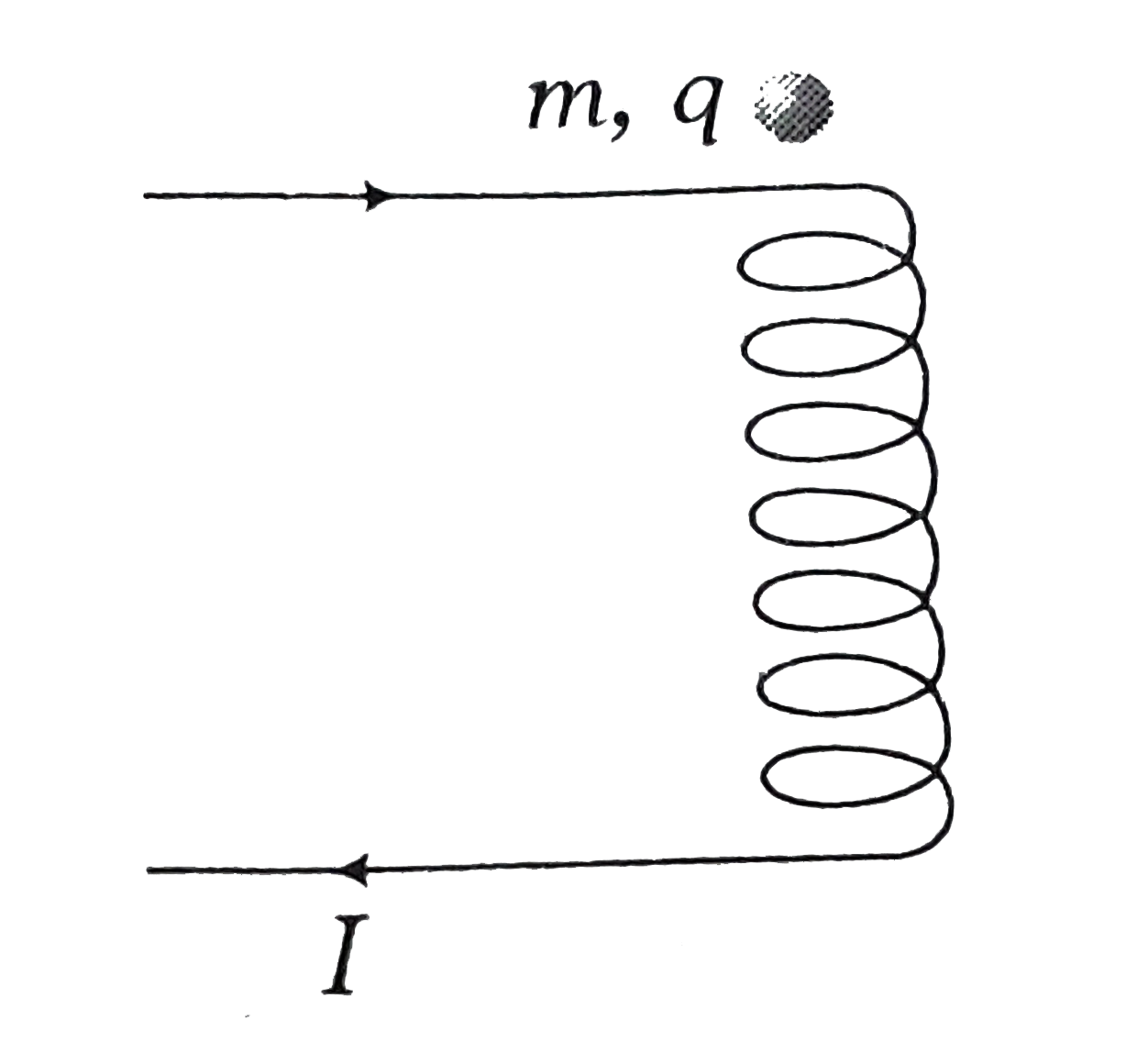
View SolutionA long solenoid carrying a current I is placed with its axis vertical as shown in the figure. A particle of mass m and charge q is released from the top of the solenoid. Its acceleration is (g being acceleration due to gravity)
View SolutionAn infinte wire having linear charge density λ is arranged as shown in fig. A charge particle of mass m and charge q is released charged q is released from point P. Find the initial acceleration of the particle just after the particle is released.
View SolutionA circular ring of radius R with uniform positive charge density λ per unit length is located in the y z plane with its center at the origin O. A particle of mass m and positive charge q is projected from that point p(−√3R,0,0) on the negative x - axis directly toward O, with initial speed V. Find the smallest (nonzero) value of the speed such that the particle does not return to P ?
View SolutionPositive charge Q is distributed uniformly over a circular ring of radius R. A particle having a mass m and a negative charge q, is placed on its axis at a distance x from the centre. Find the force on the particle. Assuming x is very less than R, find the time period of oscillation of the particle if it is released from there.
View SolutionA particle of mass m and positive charge q is projected with a speed of v0 in y–direction in the presence of electric and magnetic field are in x–direction. Find the instant of time at which the speed of particle becomes double the initial speed.
View SolutionA thin circular disk of radius R is uniformly charged with density σ>0 per unit area.The disk rotates about its axis with a uniform angular speed ω.The magnetic moment of the disk is :
View SolutionA charge particle of mass m and charge q is projected with velocity v along y-axis at t=0.

Find the velocity vector and position vector of the particle →v(t)and→r(t) in relation with time.
View Solution