A street car moves rectilinearly from station A to the next station B (from rest to rest) with an acceleration varying according to the law f=a−bx, where a and b are constants and x is the distance from station A. The distance between the two stations and the maximum velocity are
The correct Answer is:2
f=a−bx
For maximum velocity, acceleration should be zero.
i.e. a−bx=0⇒x=ab
∴ At x=ab, the particle has its maximum velocity
f=vdvdx=a−bx⇒v22=ax−bx22+c
At x=0,v=0⇒c=0
Substituting : x=ab, gives
vmax=a√b
Also, the velocity of the car should become zero at station B.
i.e. ax−bx22=0⇒x=0,x=(2ab)
∴ Distance between the two stations is (2a)(b)
∴N=2
Alternate : f=a−bx means particle will do SHM.
At mean position , f=0
⇒x=ab⇒ 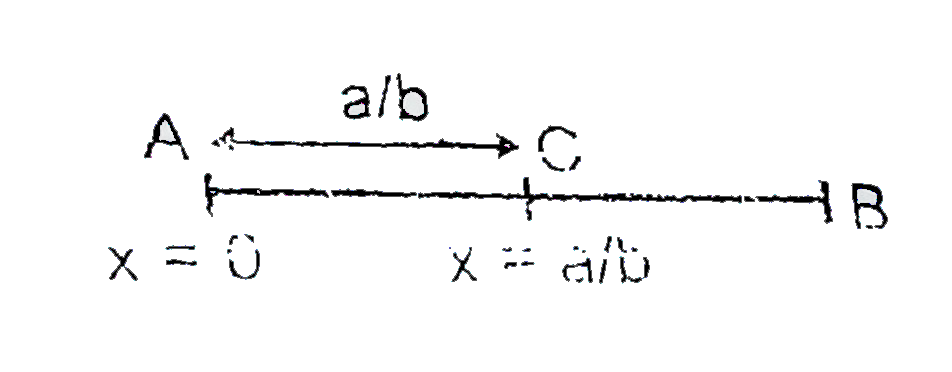
In the figure shown, 'C' is the mean positions and A & B are extreme positions
∴xmax=2ab
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION Book:RESONANCE ENGLISHChapter:SIMPLE HARMONIC MOTION Exercise:Exercise- 2, PART - II
Explore 1 Video
- SIMPLE HARMONIC MOTION Book:RESONANCE ENGLISHChapter:SIMPLE HARMONIC MOTION Exercise:Exercise- 2, PART - III
Explore 12 Videos
- SIMPLE HARMONIC MOTION Book:RESONANCE ENGLISHChapter:SIMPLE HARMONIC MOTION Exercise:Exercise- 1, PART - II
Explore 36 Videos
- SEMICONDUCTORSBook:RESONANCE ENGLISHChapter:SEMICONDUCTORSExercise:Exercise 3
Explore 88 Videos
- TEST PAPERSBook:RESONANCE ENGLISHChapter:TEST PAPERSExercise:PHYSICS
Explore 784 Videos
Similar Questions
Knowledge Check
A particle starts from point A moves along a straight line path with an acceleration given by a = p - qx where p, q are connected and x is distance from point A . The particle stops at point B. The maximum velocity of the particle is
ApqBp√qCqpD√qpA body starts from rest and moves with a uniform acceleration. The ratio of the distance covered in the nth sec to the distance covered in n sec is
A2n−1n2B1n2−1nC2n2−1n2D2n+1n2
A train takes 2 h to reach station B from station A, and then 3 h to return from station B to station A. The distance between the two stations is 200 km. Find : (i) the average speed, (ii) the average velocity of the train.
View SolutionA particle starts from rest and moves with constant acceleration. Then velocity displacement curve is:
View SolutionA particle starting from rest moves with constant acceleration. If it takes 5.0 s to reash the speed 18.0 km/h find a. the average velocity during this period, and b. the distance travelled by the particle during this period.
View SolutionA body moves from rest with a constant acceleration. Which one of the following graphs represents the variation of its kinetic energy K with the distance travelled x?
View SolutionA body moves from rest with a constant acceleration. Which one of the following graphs represents the variation of its kinetic energy K with the distance travelled x?
View SolutionA train goes from rest to rest between two stations with uniform acceleration and retardation. Find the rato of maximum velocity of the average velocity.
View Solution
