The relation between time t and distance x is t=ax2+bx where aandb are constants. The acceleration is
(a)2av2
(b)−2av3
(c)abv2
(d)−2abv2
The correct Answer is:B
To find the acceleration given the relation between time t and distance x as t=ax2+bx, we will follow these steps:
Step 1: Differentiate the equation with respect to x
Given:
t=ax2+bx
We differentiate both sides with respect to x:
dtdx=2ax+b
Step 2: Find the expression for velocity v
We know that velocity v is defined as:
v=dxdt
Taking the reciprocal of the derivative we found in Step 1:
v=dxdt=1dtdx=12ax+b
Step 3: Differentiate velocity with respect to time to find acceleration
Acceleration a is defined as:
a=dvdt
Using the chain rule, we can express this as:
a=dvdx⋅dxdt
We already have dxdt=v. Now we need to find dvdx.
Step 4: Differentiate v with respect to x
From Step 2:
v=12ax+b
Now differentiate v with respect to x:
dvdx=−2a(2ax+b)2
Step 5: Substitute dvdx and dxdt into the acceleration formula
Now substituting back into the acceleration formula:
a=dvdx⋅v=−2a(2ax+b)2⋅12ax+b
This simplifies to:
a=−2av2(2ax+b)3
Final Expression for Acceleration
Thus, the acceleration is given by:
a=−2Av3
Given:
t=ax2+bx
We differentiate both sides with respect to x:
dtdx=2ax+b
We know that velocity v is defined as:
v=dxdt
Taking the reciprocal of the derivative we found in Step 1:
v=dxdt=1dtdx=12ax+b
Acceleration a is defined as:
a=dvdt
Using the chain rule, we can express this as:
a=dvdx⋅dxdt
We already have dxdt=v. Now we need to find dvdx.
From Step 2:
v=12ax+b
Now differentiate v with respect to x:
dvdx=−2a(2ax+b)2
Now substituting back into the acceleration formula:
a=dvdx⋅v=−2a(2ax+b)2⋅12ax+b
This simplifies to:
a=−2av2(2ax+b)3
Thus, the acceleration is given by:
a=−2Av3
Topper's Solved these Questions
- KINEMATICS OF A PARTICLE Book:VMC MODULES ENGLISHChapter:KINEMATICS OF A PARTICLE Exercise:JEE Advanced (archive)
Explore 14 Videos
- KINEMATICS OF A PARTICLE Book:VMC MODULES ENGLISHChapter:KINEMATICS OF A PARTICLE Exercise:LEVEL 2
Explore 65 Videos
- JEE MAIN REVISON TEST-23Book:VMC MODULES ENGLISHChapter:JEE MAIN REVISON TEST-23Exercise:PHYSICS (SECTION 2)
Explore 1 Video
- LAWS OF MOTION Book:VMC MODULES ENGLISHChapter:LAWS OF MOTION Exercise:IMPECCABLE
Explore 53 Videos
Similar Questions
The relation between time t and displacement x is t=αx2+βx, where αandβ are constants. The retardation is
View SolutionThe relation between time t and distance x of a particle is given by t=Ax2+Bx, where A and B are constants. Then the
(A) velocity is given by v=2Ax+B
(B) velocity is given by v=(2Ax+B)−1
(C) retardation is given by 2Av3
(D) retardation is given by 2Bv2
Select correct statement :−
View Solution
Knowledge Check
A particle moves rectilinearly. Its displacement x at time t is given by x2=at2+b where a and b are constants. Its acceleration at time t is proportional to
A1x3B1x−1x2C−1x2D1x−t2x3
The equation of motion of a projectile is y=ax−bx2, where a and b are constants of motion. Match the quantities in Column I with the relations in Column II.
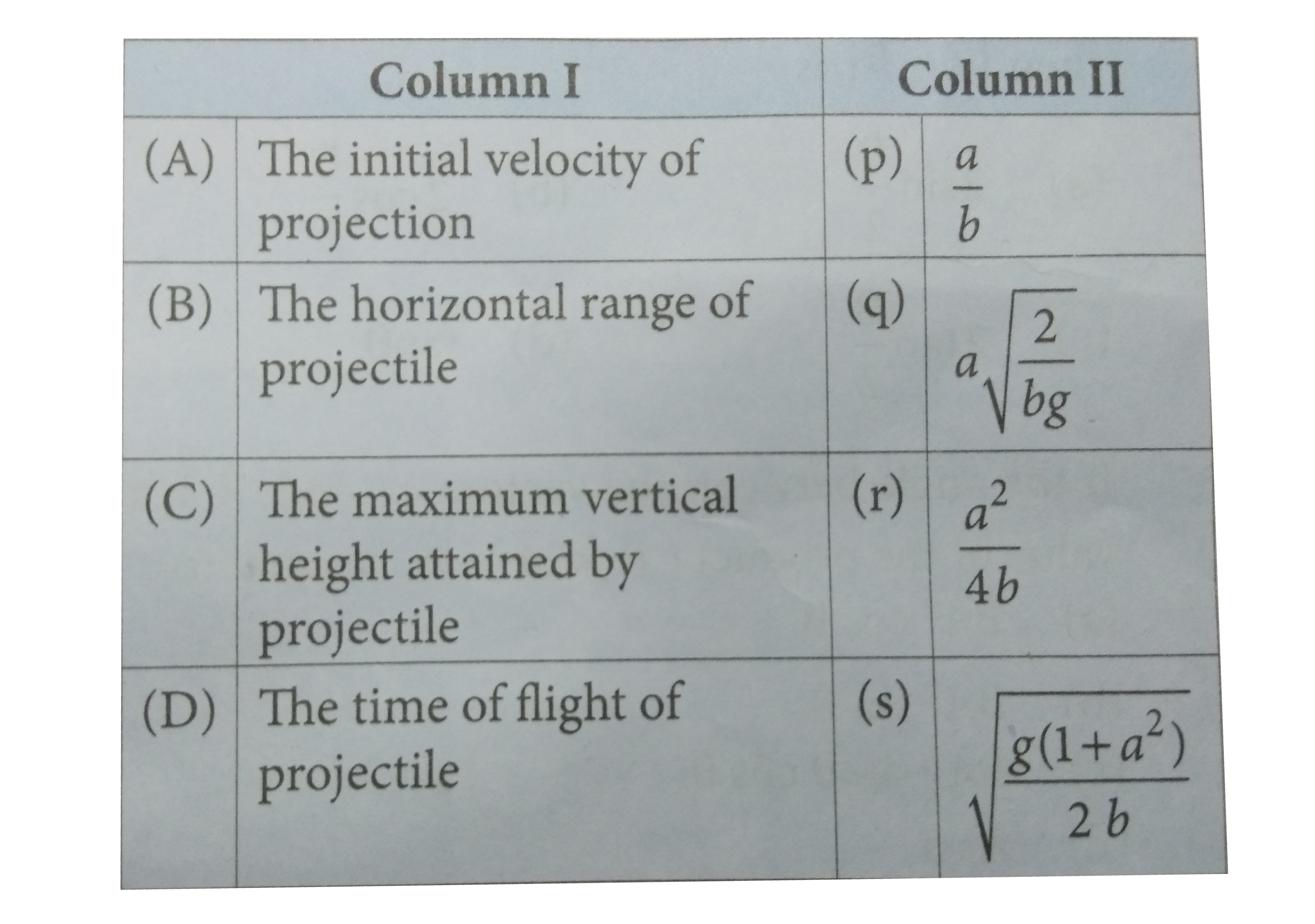
View SolutionThe x and y coordinates of a particle at any time t are given by x=2t+4t2andy=5t , where x and y are in metre and t in second. The acceleration of the particle at t = 5 s is
View SolutionThe potential energy between two atoms in a molecule is given by
U=ax2−bx2
where a and b are positive constants and x is the distance between the atoms. The atom is in stable equilibrium when x is equal to :−
View SolutionThe reation between the time t and position x for a particle moving on x-axis is given by t=px2+qx, where p and q are constants. The relation between velocity v and acceleration a is as
View Solution(−3x2+5x−2)−2(x2−2x−1)
If the expression above is rewritten in the form ax2+bx+c , where a, b, and c are constants, what is the value of b ?
View SolutionThe position of a particle as a function of time t, is given by x(t)=at+bt2−ct3 where a,b and c are constants. When the particle attains zero acceleration, then its velocity will be :
View SolutionThe velocity v of a particle at time t is given by v=at+bt+c, where a, b and c are constants. The dimensions of a, b, c are respectively :-
View Solution