In the figure shown mass 2m is at rest and in equilibrium. A particle of mass m is released from height 4.5mgk from plate. The particle sticks to the plate. Neglecting the duration of collision. Starting from the when the particles sticks to plate to the time when the spring is in maximum compression for the first time is 2π√mak then find a.
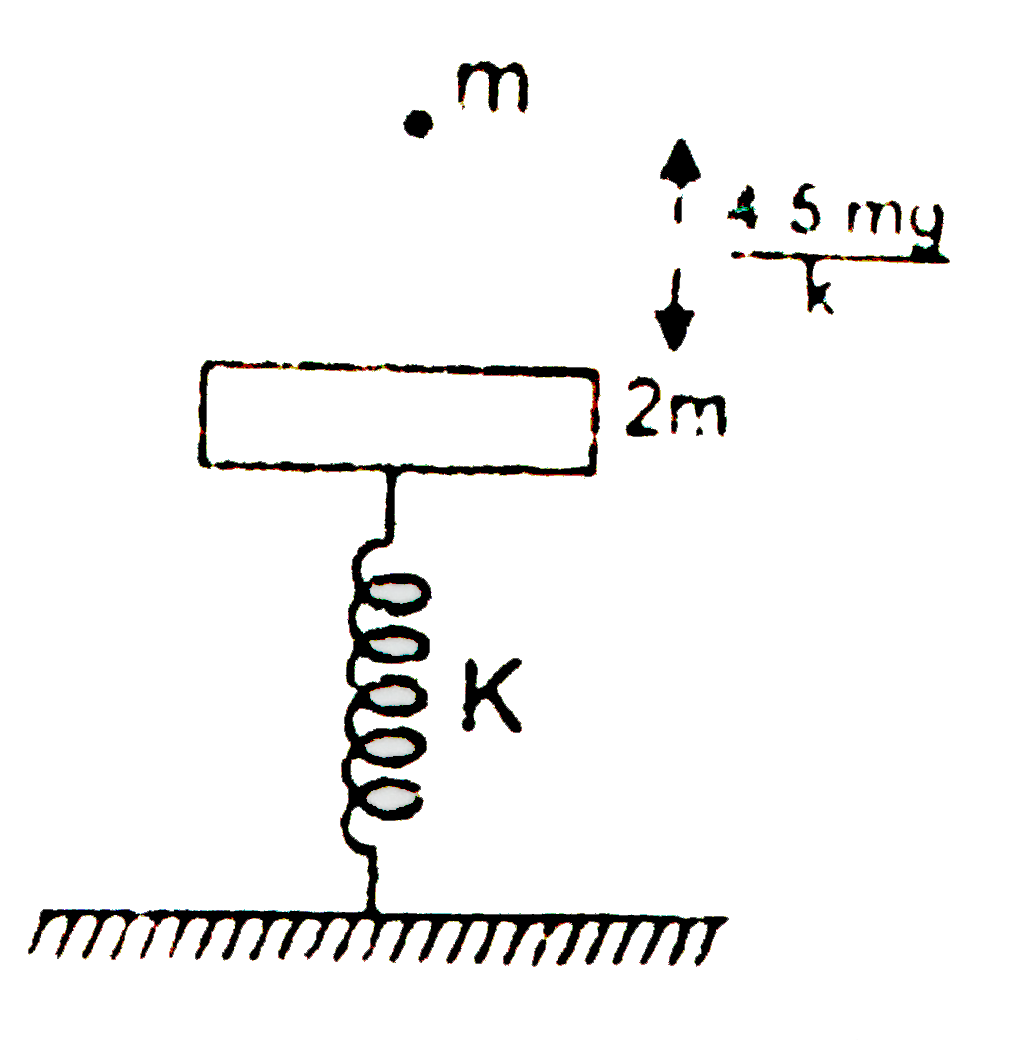
The correct Answer is:3
Velocity of the narticle just before collision
u=√2g×4.5mgK
u=3g√mK
Now it collides with the plate.
Now just after collision velocity of systeam of plate + particle
μ=3mv
⇒V=u3=g√mK
Now system perform 's SHM with time period T=2π√3mKand mean position as mgK distance below the point of collision.
Let the equation of motion be.
y=Asin(ωt+ϕ)
for t=0y=ng/K
mgK=Asinϕ....(1)
Now for amplitude
V=ω√A2−x2
g√mK=√K3m√A2−m2g2K2,(√3mgK)2=A2−m2g2K2
A=2mgK....(2)
By (1) & (2)
T=2π√3mK
X=A2 to x=0⇒t=T12
x=0 to x=A⇒t=T/4
total time =T12+T4=2π3√3mK=2π√m3k∴a=3
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 2, PART - II1videos
SIMPLE HARMONIC MOTION
- BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 2, PART - III12videos
SIMPLE HARMONIC MOTION
- BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 1, PART - II36videos
SIMPLE HARMONIC MOTION
- BOOK - RESONANCE ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Exercise 388videos
SEMICONDUCTORS
- BOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PHYSICS784videos
TEST PAPERS
Similar Questions
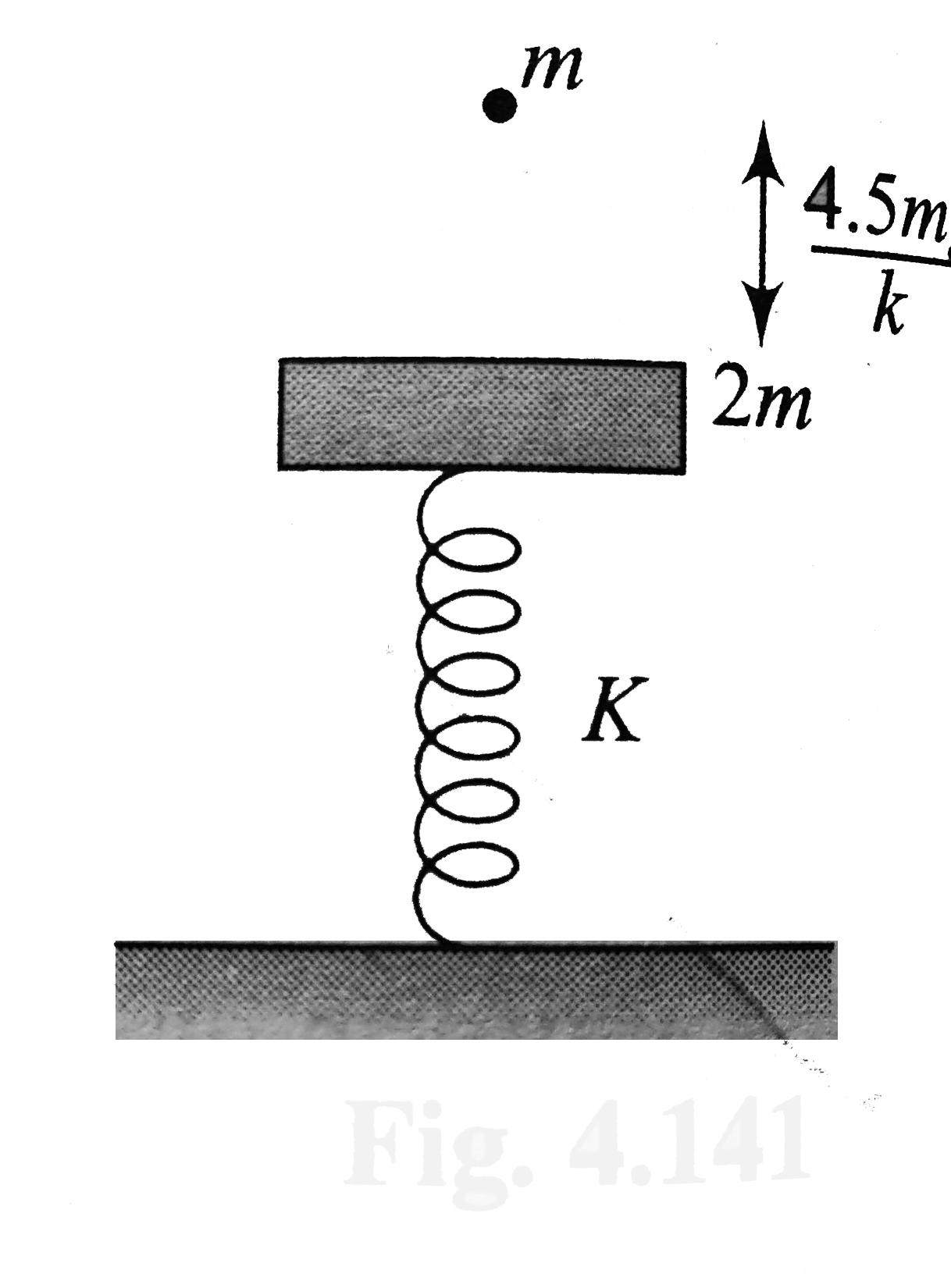
In the figure shown, mass 2m connected with a spring of force constant k is at rest and in equilibrium. A particle of mass m is released from height 4.5mg/k from 2m . The particle stick to the block. Neglecting the duration of collision find time from the release of m to the moment when the spring has maximum compression.
 View Solution
View SolutionIn the figure shown, mass 2m connected with a spring of force constant k is at rest and in equilibrium. A partical of mass m is released from height 4.5mg/k from 2m . The partical stick to the block. Neglecting the duration of collision find time from the release of m to the moment when the spring has maximum compression.
 View Solution
View SolutionA particle starts from rest. Its acceleration is varying with time as shown in the figure. When the particle comes to rest, its distance from its starting point is
 View Solution
View SolutionA particle of mass m and charge q is released from rest in uniform electric field of intensity E. Calculate the kinetic energy it attains after moving a distance y between the plates.
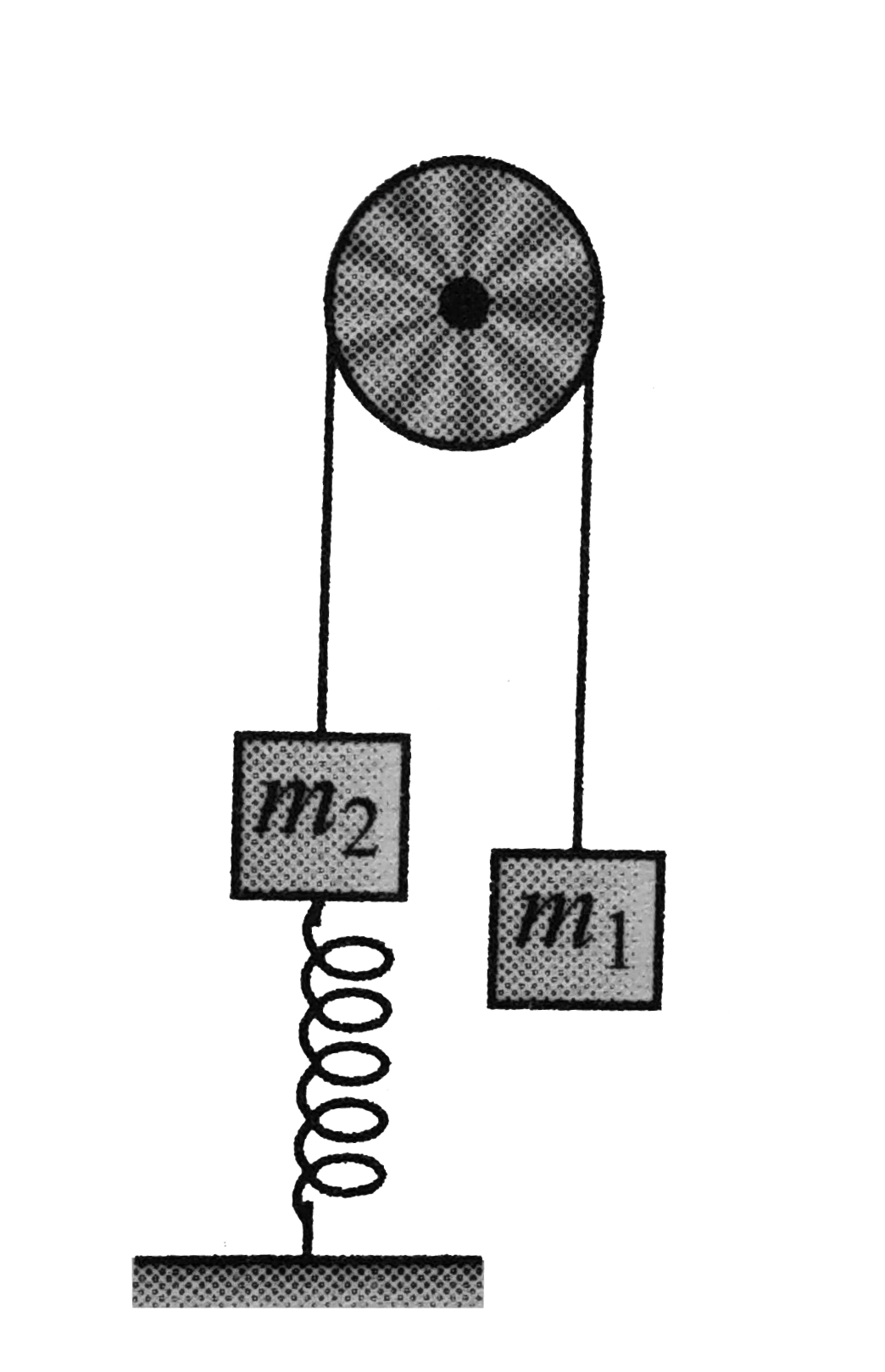
View SolutionIn an ideal pulley particle system, mass m2 is connected with a vertical spring of stiffness k. If mass m2 is released from rest, when the spring is underformed, find the maximum compression of the spring.
 View Solution
View SolutionA particle of mass m is released from rest and follow a particle part as shown Assuming that the displacement of the mass from the origin is small which graph correctly depicts the position of the particle as a function of time?
 View Solution
View SolutionA point particle of mass m is released from a distance √3R along the axis of a fixed ring of mass M and radius R as shown in figure. Find the speed of particle as it passes through the centre of the ring.
 View Solution
View SolutionA block of mass m is released from a height h from the top of a smooth surface. There is an ideal spring of spring constant k at the bottom of the track. Find the maximum compression in the spring (Wedge is fixed)
 View Solution
View SolutionA particle of mass m is driven by a machine that delivers a constant power k watts. If the particle starts from rest the force on the particle at time t is
View SolutionA particle of mass M starting from rest undergoes uniform acceleration. If the speed acquired in time T is V, the power delivered to the particle is -
View Solution
