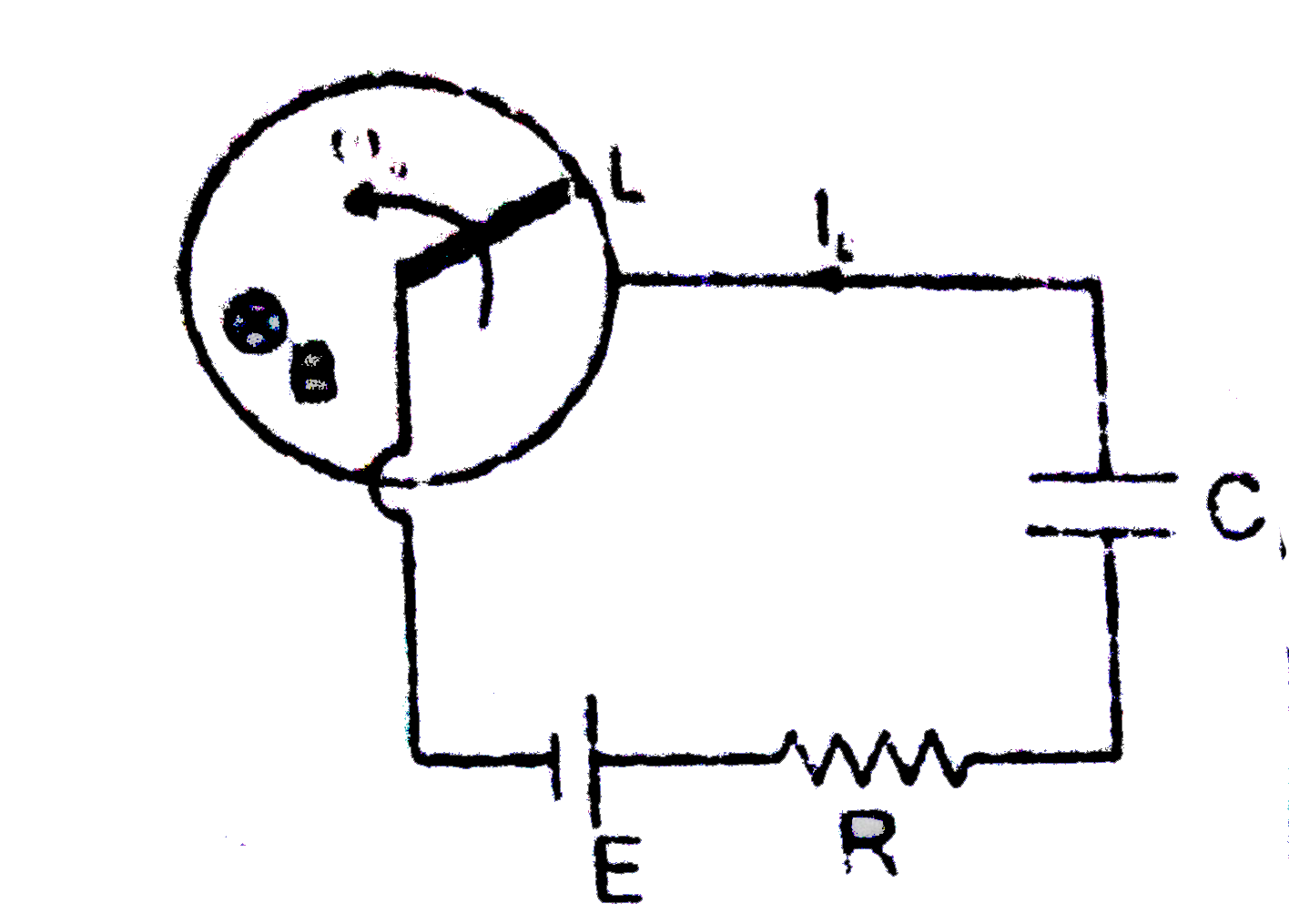
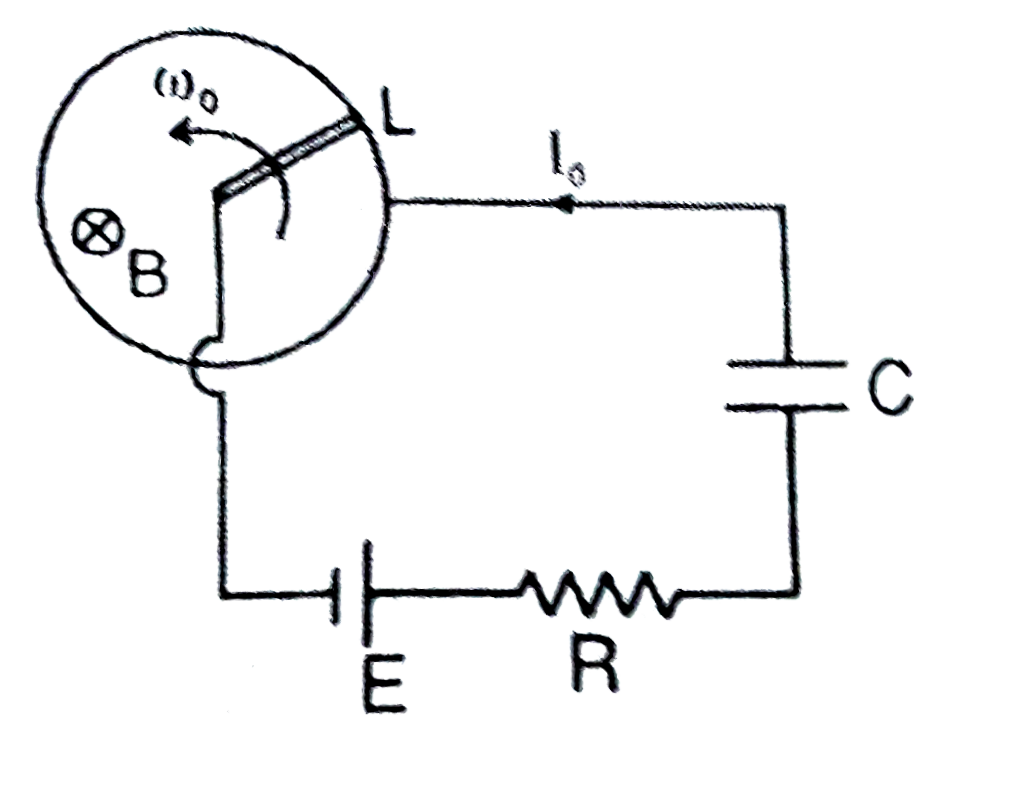
L is a smooth conducting loop of radius l=1.0m & fixed in a horizontal plane.A conducting rod of mass m=1.0kg and length slightly greater than l higed at the centre of the loop can rotate in the horizontal plane such that the free end slides on the rim of the loop.There is a uniform magnetic field of strength B=1.0T directed vertically downward.The rod is rotated with angular velocity ω0=1.0rad/s and left.The fixed end of the rod and the rim of the loop are connected through a battery of e.m.f E a resistance R=1Ω, and initially uncharged capacitor of capacitance C=1.0F in series.Find
(i)the time dependence of e.m.f M such that the current I0=1.0A in the circuit is contant.
(ii)energy supplied by the battery by the time rod stops.
The correct Answer is:A, B, C, D
(i)The magnetic equivalent of the loop-rod system.
ε=12ωl2
where ω=angular velocity of rod at any time t then ω=ω0−αt
∵τ=Iα⇒(I0lB)l2=ml23α
⇒α=3I0B2=32rad/s2
applying loop law, ε+E−I0R−qC=0
12Bl2(ω0−αt)+E−I0R−I0tC=0⇒E=I0(R+tC)−12Bl2(ω0−αt)=12+7t4
(ii)ω=0=ω0−32t0 so t0=2ω03=23sec.
Also energy supplied by battery =t∘∫0EI0di==23∫0(12+7t4)I0dt=t2+7t28∣230=1318J
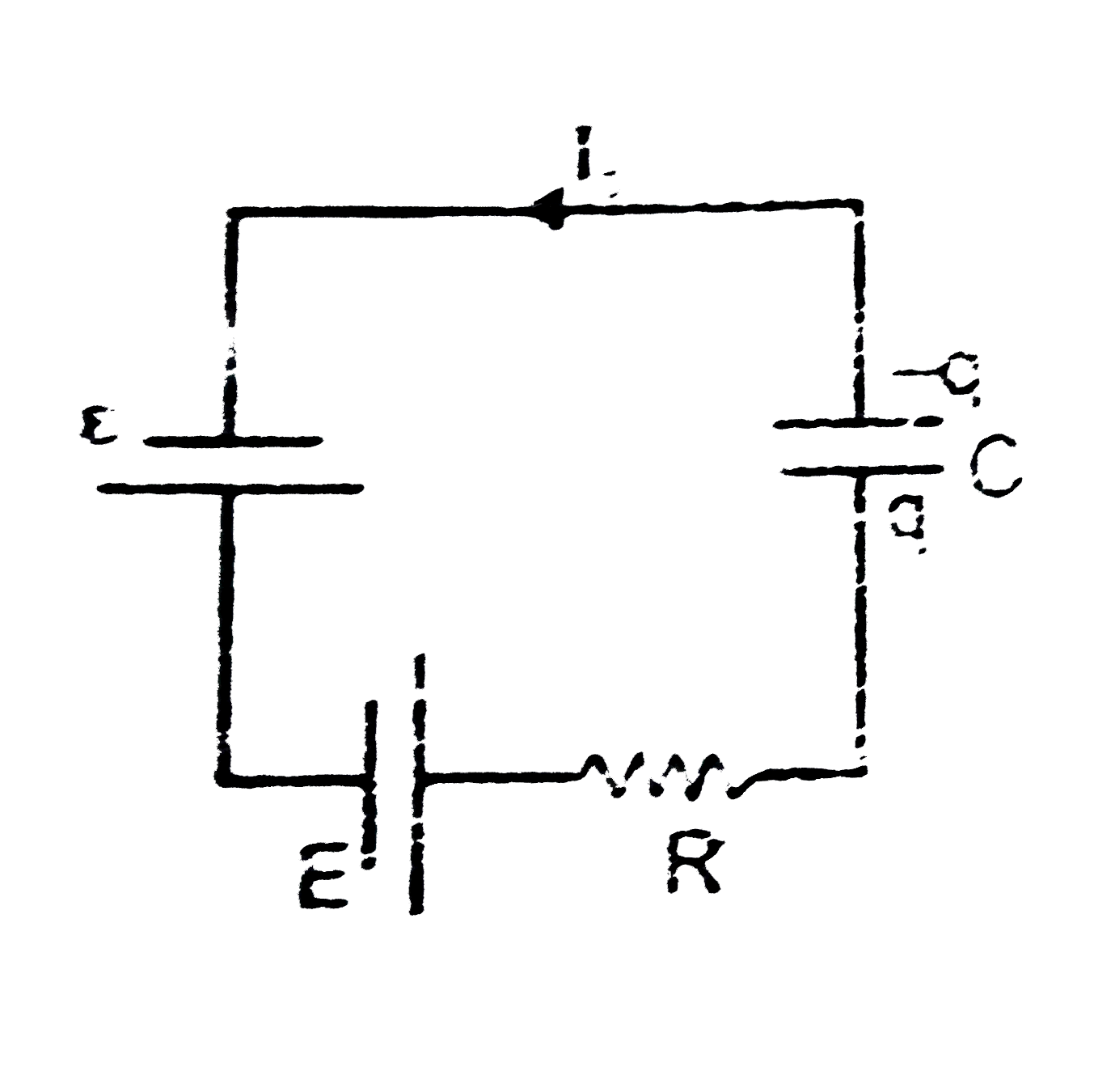
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-3 PART 3
16 Videos
- ELECTRODYNAMICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTRODYNAMICSEXERCISE - Advanced level problems
31 Videos
- ELECTROSTATICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - HLP
40 Videos
Similar Questions
A mercury drop of radius 1 cm is sprayed into 106 droplets of equal size. Calculate the energy expended if surface tension of mercury is 35×10−3N/m .
View SolutionCalculate the magnetic moment (in Am2 ) of a thin wire with a current I = 8A, wound tightly on half a torus (see figure). The diameter of the cross section of the torus is equal to d = 5 cm, and the number of turns is N = 500.

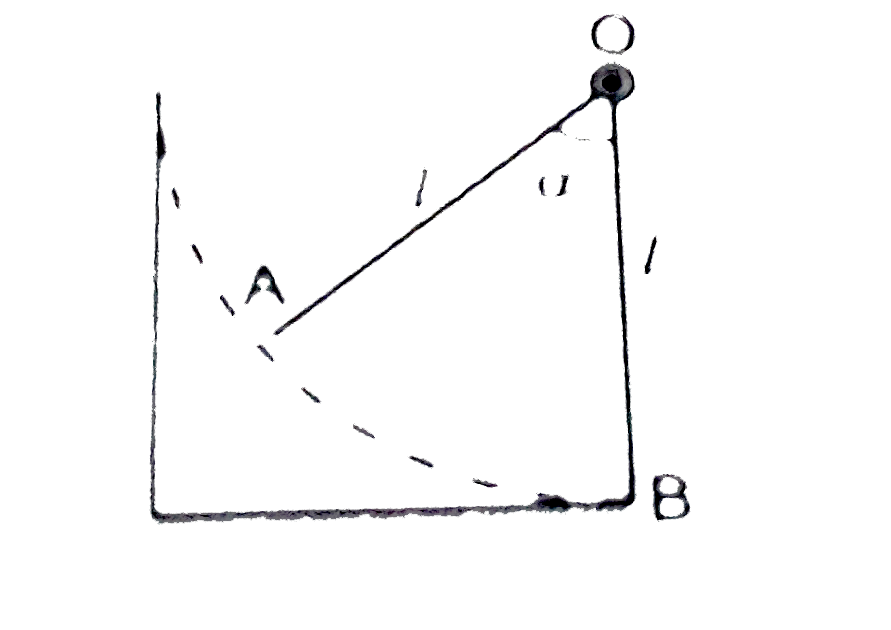
View SolutionAn electrometer consister of a fixed vertical metal bar OB at the top of which is attached a thin rod OA which gets deflected from the bar under the action of an electric charge (fig.). The rod can rotate in vertical plane about fixed horizontal axis passing through O. The reading is taken on a quadrant graduated in degrees. The length of the rod is l and its mass is m. what will be the charge when the rod of such an electrometer is deflected through an angle α in equilibrium. Find the answer using the following two assumptions :
The charge on the electrometer is equally distributed between the bar & the rod and the charges are concentrated at point A on the rod & at point B on the bar.
View SolutionA uniform rod of mass m , length l rests on a smooth horizontal surface. Rod is given a sharp horizontal impulse p perpendicular to the rod at a distance l//4 from the centre. The angular velocity of the rod will be
View SolutionA uniform rod AB of mass m and length L rotates about a fixed vertical axis making a constant angle theta with it as shown in figure. The rod is rotated about this axis, so that point B the free end of the rod moves with a uniform speed V in the horizontal plane then the angular momentum of the rod about the axis is:
View SolutionA conducting square loop of side length a is held vertical with the help of a non conducting rod of length L. The rod is made to rotate in horizontal circle about a vertical axis through its end. The loop rotates with the rod while its plane always remains perpendicular to the rod. The resistance of the loop is R and angu- lar speed of the rod is ω. There is a uniform horizontal magnetic field B in the entire space. Find the average rate of heat dissipation in the loop during one rotation.
View SolutionL is a smooth conducting loop of radius l=1.0 m & fixed in a horizontal plane.A conducting rod of mass m=1.0 kg and length slightly greater than l higed at the centre of the loop can rotate in the horizontal plane such that the free end slides on the rim of the loop.There is a uniform magnetic field of strength B=1.0 T directed vertically downward.The rod is rotated with angular velocity omega_(0)=1.0 rad//s and left.The fixed end of the rod and the rim of the loop are connected through a battery of e.m.f E a resistance R=1 Omega , and initially uncharged capacitor of capacitance C=1.0 F in series.Find (i)the time dependence of e.m.f M such that the current I_(0)=1.0 A in the circuit is contant. (ii)energy supplied by the battery by the time rod stops.
View SolutionL is a smooth conducting loop of radius l=1.0 m & fixed in a horizontal plane. A conducting rod of mass m=1.0 kg and length slightly greater than l hinged at the centre of the loop can rotate in the horizontal plane such that the free end slide on the rim of the loop. There is a uniform magnetic field of strength B=1.0 T directed vertically downward. The rod is rotated with angular velocity ω0=1.0rad/s and left. The fixed end of the rod and the rim of the loop are connected through a battery of emf . E, a resistor of resistance R=1.0Ω, and intially uncharged capacitor of capacitance C=1.0 F in series. Find :
(i) the time dependence of emf . E such that the current I0=1.0A in the circuit is constant.
(ii) energy supplied by the battery by the time rod stops.
View SolutionA long uniform rod of length L, mass M is free to rotate in a horizontal plane about a vertical axis through its end. Two springs of constant K each are connected as shown. On equilibrium, the rod was horizontal. The frequency will be –
View Solutionl लम्बाई की एक भारहीन छड़ पर दो समान द्रव्यमान m चित्रनुसार लगाए गए हैं ,छड़ बिंदु C के पारित : ऊर्ध्वाधर तल मे घूर्णन कर सकती है। छड़ के सिरे A पर कितना क्षैतिज वेग दिया जाना चाइए की यह क्षैतिज दिशा मे मुड़ जाए ?

View SolutionA conducting rod of length l is higed at point O. It is free to rotate in a vertical plane. There exists a uniform magnetic field vec(B) in horizontal direction. The rod is released from the position shown in figure. Potential difference between the two ends of the rod is proportional to
View Solution
A uniform rod AB of mass m and length L rotates about a fixed vertical axis making a constant angle θ with it as shown in figure. The rod is rotated about this axis, so that point B the free end of the rod moves with a uniform speed V in the horizontal plane then the angular momentum of the rod about the axis is:
View SolutionA uniform rod of length L is free to rotate in a vertical plane about a fixed horizontal axis through B . The rod begins rotating from rest. The angular velocity omega at angle theta is given as
View SolutionA uniform rod of length l and mass m is free to rotate in a vertical plane about A as shown in Fig. The rod initially in horizontal position is released. The initial angular acceleration of the rod is

View SolutionA uniform rod of mass m, length l rests on a smooth horizontal surface. Rod is given a sharp horizontal impulse p perpendicular to the rod at a distance l/4 from the centre. The angular velocity of the rod will be
View SolutionA horizontal rod of length 'l' rotates about a vertical axis with a uniform angular velocity 'omega' . A uniform magnetic field of induction B exists parallel to the axis of rotation. The potential different between the ends of the rod is
View Solution
