the linear charge density of a thin metallic rod varies with the distance x from the end as λ=λ0x2(0≤x≤l) The total charge on the rod is
The correct Answer is:B, C
To find the total charge on the thin metallic rod with a linear charge density that varies with distance, we can follow these steps:
1. Understand the Linear Charge Density:
The linear charge density is given by the equation:
λ(x)=λ0x2
where λ0 is a constant and x varies from 0 to L.
2. Define a Small Element of Charge:
Consider a small element of the rod at a distance x with a small length dx. The charge dQ on this small element can be expressed as:
dQ=λ(x)⋅dx=λ0x2⋅dx
3. Integrate to Find Total Charge:
To find the total charge Q on the rod, we need to integrate dQ from x=0 to x=L:
Q=∫L0dQ=∫L0λ0x2dx
4. Perform the Integration:
The integral can be computed as follows:
Q=λ0∫L0x2dx
The integral of x2 is:
∫x2dx=x33
Therefore, we have:
Q=λ0(x33)L0=λ0(L33−0)=λ0L33
5. Final Result:
Thus, the total charge Q on the rod is:
Q=λ0L33
The linear charge density is given by the equation:
λ(x)=λ0x2
where λ0 is a constant and x varies from 0 to L.
Consider a small element of the rod at a distance x with a small length dx. The charge dQ on this small element can be expressed as:
dQ=λ(x)⋅dx=λ0x2⋅dx
To find the total charge Q on the rod, we need to integrate dQ from x=0 to x=L:
Q=∫L0dQ=∫L0λ0x2dx
The integral can be computed as follows:
Q=λ0∫L0x2dx
The integral of x2 is:
∫x2dx=x33
Therefore, we have:
Q=λ0(x33)L0=λ0(L33−0)=λ0L33
Thus, the total charge Q on the rod is:
Q=λ0L33
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBook:RESONANCE ENGLISHChapter:ELECTROMAGNETIC INDUCTIONExercise:Exercis-3 PART 3
Explore 16 Videos
- ELECTRODYNAMICSBook:RESONANCE ENGLISHChapter:ELECTRODYNAMICSExercise:Advanced level problems
Explore 31 Videos
- ELECTROSTATICSBook:RESONANCE ENGLISHChapter:ELECTROSTATICSExercise:HLP
Explore 40 Videos
Similar Questions
The density of a thin rod of length l varies with the distance x from one end as ρ=ρ0x2l2. Find the position of centre of mass of rod.
View SolutionIf linear charge density of a wire as shown in the figure is λ

View Solution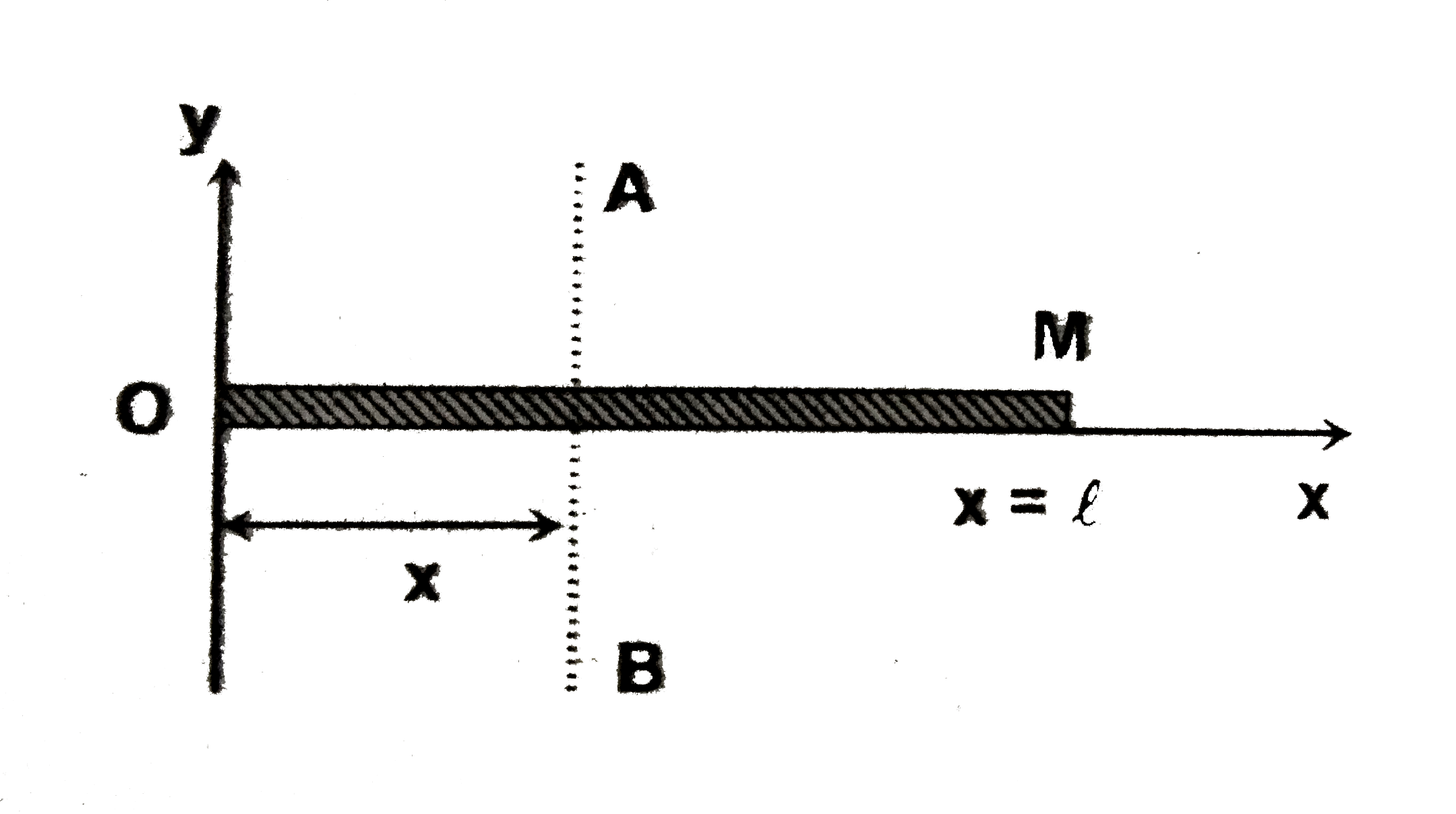
A non uniform rod OM (of length l m) is kept along x-axis and rotating about an axis AB, which is perpendicular to rod as shown in the figure. The rod has linear mass density that varies with the distance x from left end of the rod according to λ=λ0(x3L3)
Where unit of λ0 is kg/m. What is the value of x so that moment of inertia of rod about axis AB (IAB) is minimum?
View SolutionLinear mass density of a rod AB(of≤n>h10m) varies with distance x from its end A as λ =λ0x3 (λ0 is positive constant) . Distance of centre of mass of the rod , from end B is
View Solution
A non uniform rod OM (of length l m) is kept along x-axis and rotating about an axis AB, which is perpendicular to rod as shown in the figure. The rod has linear mass densty that varies with the distance x from left end of the rod according to λ=λ0(x3L3)
Where unit of λ0 is kg/m. What is the value of x so that moment of inertia of rod about axis AB (IAB) is minimum?
View SolutionLinear mass density of a rod AB ( of length 10 m) varied with distance x from its end A as λ=λ0x3 (λ0 is poitive constant). Distance of centre of mass the rod, form end B is
View SolutionA rod of length L is placed along the X-axis between x=0andx=L. The linear density (mass/length) ρ of the rod varies with the distance x from the origin as ρ=a+bx. a.) Find the SI units of a and b b.) Find the mass of the rod in terms of a,b, and L.
View SolutionA rod of length L is placed along the x-axis between x=0 and x=L. The linear mass density (mass/length) ρ of the rod varies with the distance x from the origin as ρ=a+bx. Here, a and b are constants. Find the position of centre of mass of this rod.
View SolutionLinear mass density of a rod PQ of length l and mass m is varying with the distance x (from P), as λ=m2l(1+ax)
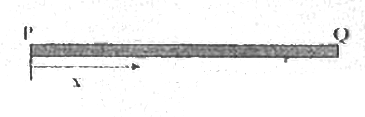
(i) Determine the value of a
(ii) Also determine the distance of c.m. from the end P.
View SolutionThe linear charge density on a dielectric ring of radius R vanes with θ as λ=λ0cosθ/2, where λ0 is constant. Find the potential at the center O of the ring [in volt].
View Solution