An extended object is placed perpendicular to the principal axis of a concave mirror of radius of curvature 20cm at a distance of 15 cm from the pole. Find the lateral magnification produced.
To solve the problem of finding the lateral magnification produced by a concave mirror when an extended object is placed perpendicular to the principal axis, we can follow these steps:
1. Identify Given Values:
- Radius of curvature (R) = 20 cm
- Object distance (U) = -15 cm (the negative sign indicates that the object is in front of the mirror)
2. Calculate the Focal Length (f):
- The focal length (f) of a concave mirror is given by the formula:
f=−R2
- Substituting the value of R:
f=−20 cm2=−10 cm
3. Use the Mirror Formula:
- The mirror formula is given by:
1f=1v+1u
- Substituting the known values:
1−10=1v+1−15
4. Rearranging the Equation:
- To find 1v:
1v=1−10+115
- Finding a common denominator (30):
1v=−330+230=−130
5. Calculate the Image Distance (v):
- Taking the reciprocal to find v:
v=−30 cm
6. Calculate the Lateral Magnification (M):
- The magnification (M) is given by:
M=−vu
- Substituting the values:
M=−−30−15=2
Final Answer:
The lateral magnification produced is 2.
- Radius of curvature (R) = 20 cm
- Object distance (U) = -15 cm (the negative sign indicates that the object is in front of the mirror)
- The focal length (f) of a concave mirror is given by the formula:
f=−R2
- Substituting the value of R:
f=−20 cm2=−10 cm
- The mirror formula is given by:
1f=1v+1u
- Substituting the known values:
1−10=1v+1−15
- To find 1v:
1v=1−10+115
- Finding a common denominator (30):
1v=−330+230=−130
- Taking the reciprocal to find v:
v=−30 cm
- The magnification (M) is given by:
M=−vu
- Substituting the values:
M=−−30−15=2
The lateral magnification produced is 2.
Topper's Solved these Questions
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Problem
33 Videos
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Exercise-1
141 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCE ENGLISHCHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
- GRAVITATIONBOOK - RESONANCE ENGLISHCHAPTER - GRAVITATIONEXERCISE - HIGH LEVEL PROBLEMS
16 Videos
Similar Questions
An object is placed on the principal axis of concave mirror of focal length 10 cm at a distance of 8.0 cm from the pole. Find the position and the nature of the image.
View SolutionAn object of height 1cm is kept perpendicular to the principle axis of a convex mirror of radius of curvature 20cm. IF the distance of the object form the mirror is 20cm then the distance (in cm) between heads of the image and the object will be:
View Solution
Knowledge Check
An object is kept on the principal axis of a concave mirror of focal length 10cm, at a distance of 15 cm from its pole. The image formed by the mirror is:
AVirtual and magnifiedBVirtual and diminishedCReal and magnifiedDReal and diminished
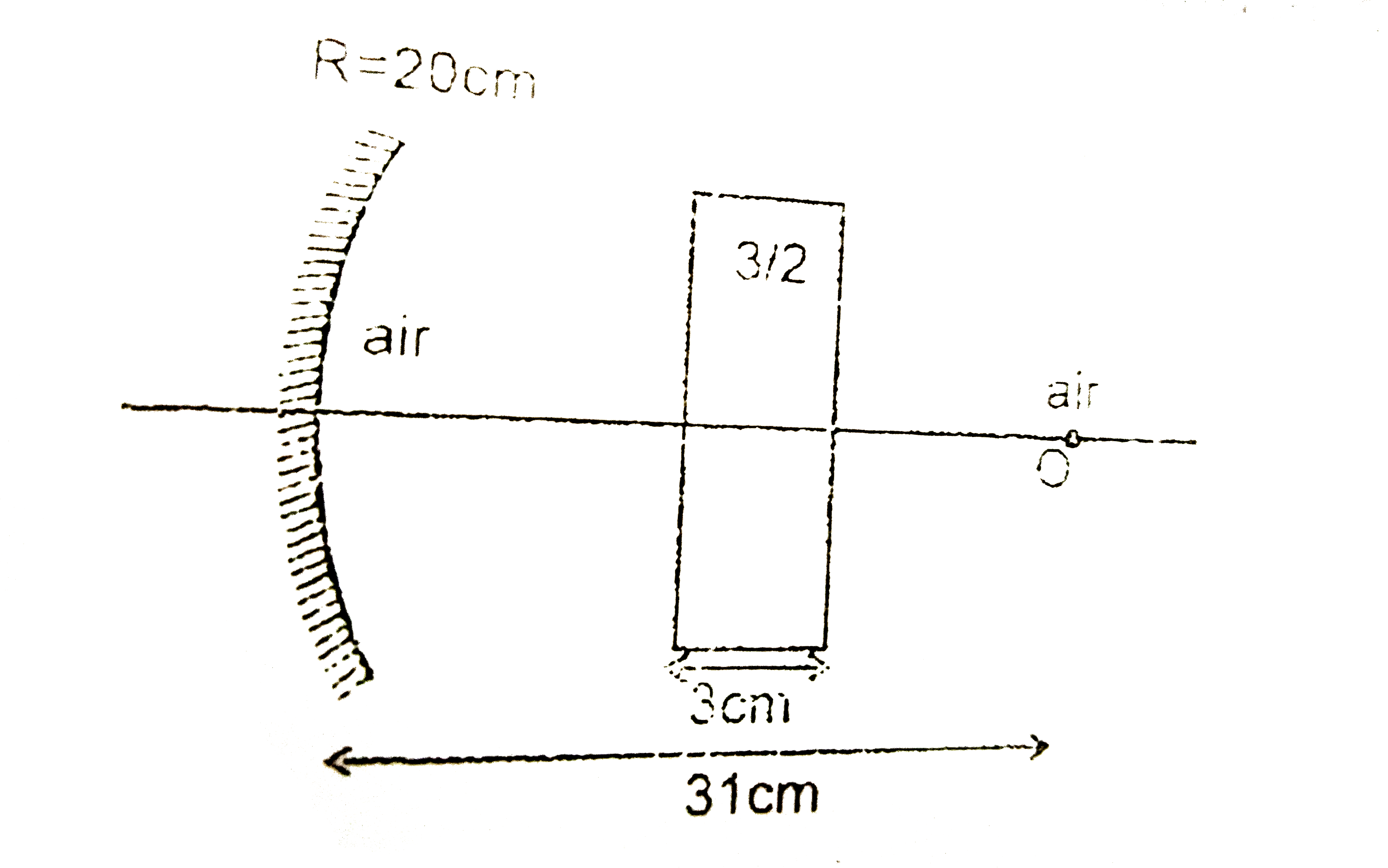
A point object is placed on principal axis of a concave mirror of radius of curvature 20cm at a distance 31cm from poll of the mirror. A glass slab of thikness 3cm and refractive index 1.5 is placed between object and mirror as shown in the figure. Find the distance (in cm) of final image formed by the system from the mirror.
View SolutionA real point object is kept on the pricipal axis of a concave mirror (radius of curvature =20cm) at a distance 60 cm from the pole. Take pole as origin and direction of incident light as positive direction of X axis. Y axis is perpendicular to the principal axis. Select correct statement(s).
View SolutionAn extended real object of size 2cm is palced perpendicular to the principle axis of a converging lens of focal length 20cm. The distance between the object and the lens is 30cm
(i) Find the laterial magnification produced by the lens.
(ii) Find the height of the image.
View SolutionA 1 cm object is placed perpendicular to the principal axis of a convex mirror of focal length 7.5 cm. Find its distance from the mirror if the image formed is 0.6 cm in size.
View SolutionA 2.0cm tall object is placed perpendicular to the principal axis of a convex lens of focal length 10 cm. The distance of the object from the lens is 15 cm. Find the nature, position and size of the image. Also, find its magnification.
View SolutionA point object is placed at a distance 20 cm from the focus of a concave mirror of radius of curvature 20 cm. Find the distance (in cm) of the image from the focus.
View SolutionA 4 cm object is placed perpendicular to the principal axis of a convex mirror of focal length 7.5 cm. The distance of the image from the mirror if it is 0.6 cm in size is 51/x where x is
View Solution
