Two plane mirrors makes an angle of 120∘ with each other. The distance between the two images of a point source formed in them is 20 cm . Determine the distance from the light source of the point where the mirrors touch, the light source lies on the bisector of the angle formed by the mirrors.
The correct Answer is:11.5cm=20√3
To solve the problem, we need to determine the distance from the light source to the point where the two mirrors touch, given that the distance between the two images formed by the mirrors is 20 cm and the angle between the mirrors is 120 degrees.
1. Understanding the Geometry:
- We have two plane mirrors making an angle of 120∘ with each other.
- The light source lies on the bisector of this angle, which means it is at an angle of 60∘ from each mirror (since 120∘/2=60∘).
2. Setting Up the Diagram:
- Let the point source be at point O and the point where the mirrors touch be point A.
- The images formed by the mirrors will be denoted as I1 and I2.
- The distance between the two images I1 and I2 is given as 20cm.
3. Identifying the Angles:
- The angle between the line OI1 and the line OA is 60∘ (the angle bisector).
- The angle between the line OI2 and the line OA is also 60∘.
4. Using Trigonometry:
- The distance from the light source O to the point where the mirrors touch A is denoted as D.
- The distance from O to I1 and I2 can be expressed using trigonometric functions:
Distance from O to I1=Dcos(60∘)=D⋅12
Distance from O to I2=Dcos(60∘)=D⋅12
5. Calculating the Total Distance:
- The total distance between the two images I1 and I2 can be expressed as:
Distance I1I2=2⋅Dcos(60∘)=2⋅D⋅12=D
- We know from the problem statement that this distance is 20cm:
D=20cm
6. Finding the Distance from the Light Source:
- Since we have established that the distance D is equal to 20cm, we can now find the distance from the light source O to the point where the mirrors touch A:
D=20cos(30∘)
- The value of cos(30∘) is √32:
D=20√32=20⋅2√3=40√3≈23.09cm
Final Answer:
The distance from the light source to the point where the mirrors touch is approximately 23.09cm.
- We have two plane mirrors making an angle of 120∘ with each other.
- The light source lies on the bisector of this angle, which means it is at an angle of 60∘ from each mirror (since 120∘/2=60∘).
- Let the point source be at point O and the point where the mirrors touch be point A.
- The images formed by the mirrors will be denoted as I1 and I2.
- The distance between the two images I1 and I2 is given as 20cm.
- The angle between the line OI1 and the line OA is 60∘ (the angle bisector).
- The angle between the line OI2 and the line OA is also 60∘.
- The distance from the light source O to the point where the mirrors touch A is denoted as D.
- The distance from O to I1 and I2 can be expressed using trigonometric functions:
Distance from O to I1=Dcos(60∘)=D⋅12
Distance from O to I2=Dcos(60∘)=D⋅12
- The total distance between the two images I1 and I2 can be expressed as:
Distance I1I2=2⋅Dcos(60∘)=2⋅D⋅12=D
- We know from the problem statement that this distance is 20cm:
D=20cm
- Since we have established that the distance D is equal to 20cm, we can now find the distance from the light source O to the point where the mirrors touch A:
D=20cos(30∘)
- The value of cos(30∘) is √32:
D=20√32=20⋅2√3=40√3≈23.09cm
The distance from the light source to the point where the mirrors touch is approximately 23.09cm.
Topper's Solved these Questions
Similar Questions
A point object is kept between a plane mirror and a concave mirror facing each other. The distance between the mirrors is 22.5 cm . The distance between the mirrors is 20 cm. what should be the distance of the object from the concave mirror so that after two successive reflections the final image is formed on the object itself ? (consider first reflection from concave mirror).
View SolutionTwo plane mirror are inclined at an angle of 72∘. The number of images of a point object placed between them will be
View SolutionTwo plane mirrors are placed parallel to each other. The distance between the mirrors is 10cm. An object is placed between the mirrors at a distance of 4cm from one of them, say M1. What is the distance between the first time formed at M1 and the second image formed at M2 ?
View SolutionThe focal length of a convex mirror is 40 cm. A point source of light is kept at distance 40 cm from the mirror. Find the distance of image from the mirror.
View SolutionThe distance between two point sources of light is 24cm. Find out where would you place a converging lens of focal length 9cm, so that the images of both the sources are formed at the same point.
View SolutionThe distance between two point sources of light is 24cm. Find out where would you place a converging lens of focal length 9cm, so that the images of both the sources are formed at the same point.
View SolutionA bi-convex lens is placed between a light source and a concave mirror as shwon such that image of the light source coincides with itself. Then,
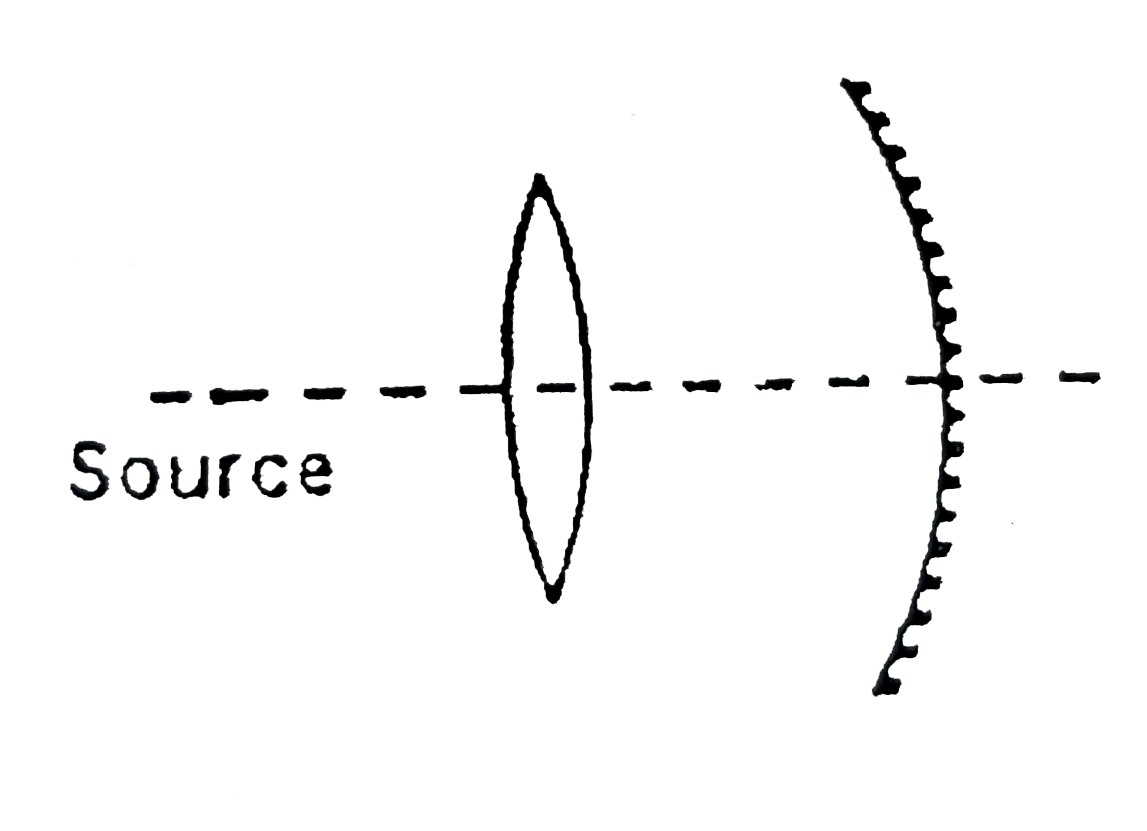
View SolutionTwo plane mirrors each 1.6 m long, are facing each other. The distance between the mirrors is
20 cm. A light incident on one end of one of the mirrors at an angle of incidence of 30∘. How many times is the ray reflected before it reaches the other end?
View SolutionTwo plane mirrors are making an angle of 60∘ to each other. A light ray falls on one of the mirrors. The light ray is incident parallel to angular bisector of mirrors. How many reflection does the light ray undergo?
View SolutionA source of light is at 10 cm from a convex mirror and is then moved to a distance of 2 cm from the mirror. How much does the image move if the radius of curvature of the mirror is 4.8 cm?
View Solution
