A real point object is kept on the pricipal axis of a concave mirror (radius of curvature =20cm) at a distance 60 cm from the pole. Take pole as origin and direction of incident light as positive direction of X axis. Y axis is perpendicular to the principal axis. Select correct statement(s).
Option1 If velocity of object t=1ˆi and velocity of mirror =0 then velocity of image will be −125ˆi
Option2 If velocity of object =1ˆj velocity of mirror =0 then velocity of image will be −15ˆj
Option3 if velocity of object =0 and velocity of mirror =1ˆi then velocity of image will be 125ˆi
Option4 if velocity of object =0 ad velocity of mirror =1ˆj then velocity of image will be 15ˆj
The correct Answer is:A, B
To solve the problem, we need to analyze the situation involving a concave mirror and the object placed in front of it. Here’s a step-by-step breakdown of the solution:
Step 1: Identify the focal length of the concave mirror
The radius of curvature (R) of the concave mirror is given as 20 cm. The focal length (F) of a concave mirror is given by the formula:
F=−R2
Substituting the value of R:
F=−20cm2=−10cm
Step 2: Determine the object distance (U)
The object is placed at a distance of 60 cm from the pole of the mirror. Since we take the pole as the origin and the direction of incident light as positive, the object distance (U) will be:
U=−60cm(real object is negative)
Step 3: Calculate the magnification (M)
The magnification (M) of a mirror is given by the formula:
M=FF−U
Substituting the values of F and U:
M=−10−10−(−60)=−10−10+60=−1050=−15
Step 4: Analyze the velocity of the object and image
1. Case 1: Object moving in the x-direction
- Let the velocity of the object with respect to the mirror be vo=1^i (in the positive x-direction).
- The velocity of the image with respect to the mirror is given by:
vi=−M2⋅vo
Substituting the values:
vi=−(−15)2⋅(1^i)=−125^i
2. Case 2: Object moving in the y-direction
- Let the velocity of the object with respect to the mirror be vo=1^j (in the positive y-direction).
- The velocity of the image with respect to the mirror is given by:
vi=−M⋅vo
Substituting the values:
vi=−(−15)⋅(1^j)=15^j
Step 5: Evaluate the options
- Option 1: Velocity of object =1^i, velocity of mirror =0 → Velocity of image =−125^i (Correct)
- Option 2: Velocity of object =1^j, velocity of mirror =0 → Velocity of image =15^j (Correct)
- Option 3: Velocity of object =0, velocity of mirror =−^i → Velocity of image calculation shows it is not correct.
- Option 4: Similar analysis shows it is not correct.
Conclusion
The correct statements are:
- Option 1 and Option 2 are correct.
The radius of curvature (R) of the concave mirror is given as 20 cm. The focal length (F) of a concave mirror is given by the formula:
F=−R2
Substituting the value of R:
F=−20cm2=−10cm
The object is placed at a distance of 60 cm from the pole of the mirror. Since we take the pole as the origin and the direction of incident light as positive, the object distance (U) will be:
U=−60cm(real object is negative)
The magnification (M) of a mirror is given by the formula:
M=FF−U
Substituting the values of F and U:
M=−10−10−(−60)=−10−10+60=−1050=−15
1. Case 1: Object moving in the x-direction
- Let the velocity of the object with respect to the mirror be vo=1^i (in the positive x-direction).
- The velocity of the image with respect to the mirror is given by:
vi=−M2⋅vo
Substituting the values:
vi=−(−15)2⋅(1^i)=−125^i
- Let the velocity of the object with respect to the mirror be vo=1^j (in the positive y-direction).
- The velocity of the image with respect to the mirror is given by:
vi=−M⋅vo
Substituting the values:
vi=−(−15)⋅(1^j)=15^j
- Option 1: Velocity of object =1^i, velocity of mirror =0 → Velocity of image =−125^i (Correct)
- Option 2: Velocity of object =1^j, velocity of mirror =0 → Velocity of image =15^j (Correct)
- Option 3: Velocity of object =0, velocity of mirror =−^i → Velocity of image calculation shows it is not correct.
- Option 4: Similar analysis shows it is not correct.
The correct statements are:
- Option 1 and Option 2 are correct.
Similar Questions
An object is placed on the principal axis of concave mirror of focal length 10 cm at a distance of 8.0 cm from the pole. Find the position and the nature of the image.
View SolutionAn extended object is placed perpendicular to the principal axis of a concave mirror of radius of curvature 20cm at a distance of 15 cm from the pole. Find the lateral magnification produced.
View Solution
Knowledge Check
An object is kept on the principal axis of a concave mirror of focal length 10cm, at a distance of 15 cm from its pole. The image formed by the mirror is:
AVirtual and magnifiedBVirtual and diminishedCReal and magnifiedDReal and diminished
An extended object is placed perpendicular to the principal axis of a concave mirror of radius of curvature 20cm at a distance of 15 cm from the pole. Find the lateral magnification produced.
View SolutionAn object is present on the principal axis of a concave mirror at a distance 30cm from it. Focal length of mirror is 20cm.
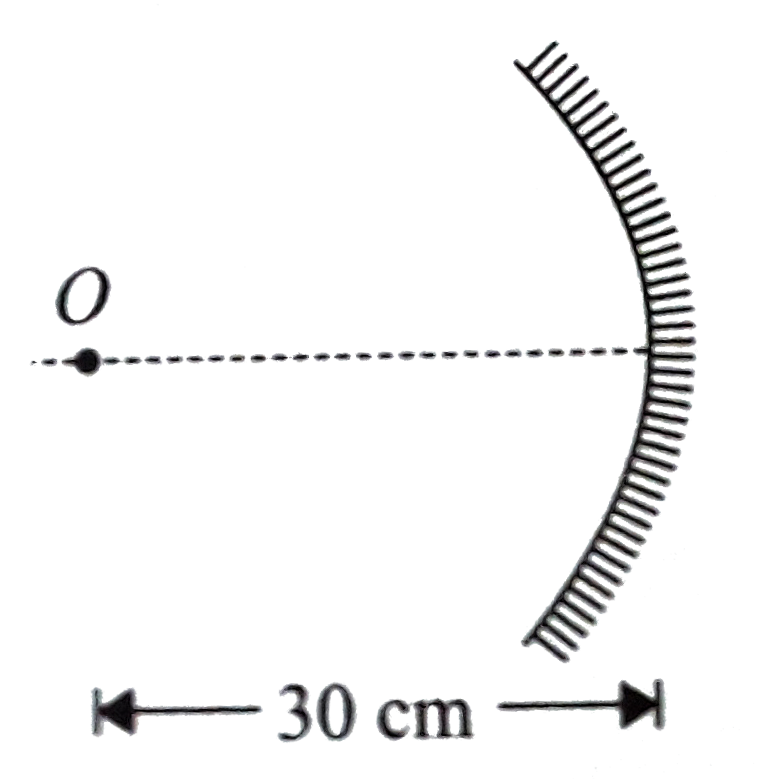
Q. Image formed by mirror is
View SolutionA point object is placed at a distance 20 cm from the focus of a concave mirror of radius of curvature 20 cm. Find the distance (in cm) of the image from the focus.
View SolutionAn object is kept on the principal axis of a convex mirror of focal length 10cm at a distance of 10cm from the pole. The object starts moving at a velocity 20mm/sce towards the mirror at angle 30∘ with the principal axis. What will be the speed of its image and direction with the principal axis at that instant.
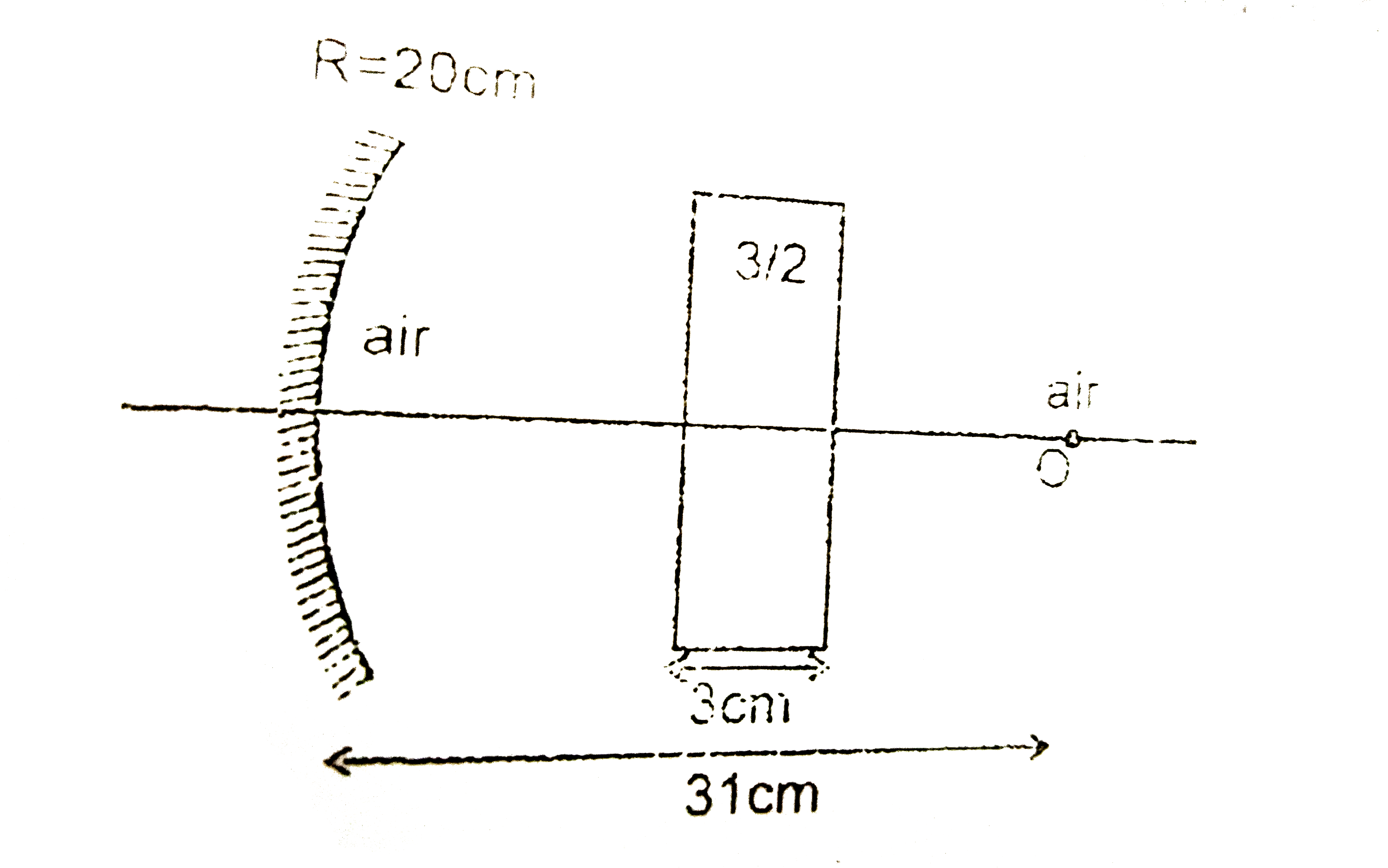
View SolutionA point object is placed on principal axis of a concave mirror of radius of curvature 20cm at a distance 31cm from poll of the mirror. A glass slab of thikness 3cm and refractive index 1.5 is placed between object and mirror as shown in the figure. Find the distance (in cm) of final image formed by the system from the mirror.
View SolutionA 2.0 cm high object is placed on the principal axis of a concave mirror at a distance of 12 cm from the pole. Ilf the image is inverted, real and 5.0 cm high, find the location of the image the focal length of the mirror.
View SolutionA point object O is placed on the principal axis of a convex lens of focal length f=20cm at a distance of 40 cm to the left of it. The diameter of the lens is 10. An eye is placed 60 cm to right of the lens and a distance h below the principal axis. The maximum value of h to see the image is
View Solution
