Two planets A and B are revolving in circular orbits around a fixed sun. Time period of the planet B is 2√2 times that of planet A. Find the ratio of solar constant for planet A to that of B. Neglect gravitational force between the planets.
The correct Answer is:4
To solve the problem, we will follow these steps:
Step 1: Understand the relationship between time period and radius
According to Kepler's third law of planetary motion, the square of the time period T of a planet is directly proportional to the cube of the radius r of its orbit around the sun. This can be expressed as:
T2∝r3
From this, we can derive the relationship:
r∝T2/3
Step 2: Relate the time periods of planets A and B
Let the time period of planet A be TA and that of planet B be TB. According to the problem, we have:
TB=2√2⋅TA
Step 3: Find the radius of planet B in terms of radius of planet A
Using the relationship derived from Kepler's law, we can express the radius of planet B in terms of the radius of planet A:
rB∝T2/3B=(2√2⋅TA)2/3
Calculating this gives:
rB∝(2√2)2/3⋅T2/3A
Calculating (2√2)2/3:
(2√2)2/3=(21⋅21/2)2/3=2(1+1/2)⋅(2/3)=2(3/2)⋅(2/3)=21=2
Thus, we have:
rB=2⋅rA
Step 4: Find the ratio of the solar constants
The solar constant (intensity of sunlight) at a distance r from the sun is inversely proportional to the square of the distance from the sun:
I∝1r2
Thus, the ratio of the solar constants IA and IB for planets A and B can be expressed as:
IAIB=r2Br2A
Substituting rB=2rA:
IAIB=(2rA)2r2A=4r2Ar2A=4
Final Answer
The ratio of the solar constant for planet A to that of planet B is:
IAIB=4
According to Kepler's third law of planetary motion, the square of the time period T of a planet is directly proportional to the cube of the radius r of its orbit around the sun. This can be expressed as:
T2∝r3
From this, we can derive the relationship:
r∝T2/3
Let the time period of planet A be TA and that of planet B be TB. According to the problem, we have:
TB=2√2⋅TA
Using the relationship derived from Kepler's law, we can express the radius of planet B in terms of the radius of planet A:
rB∝T2/3B=(2√2⋅TA)2/3
Calculating this gives:
rB∝(2√2)2/3⋅T2/3A
Calculating (2√2)2/3:
(2√2)2/3=(21⋅21/2)2/3=2(1+1/2)⋅(2/3)=2(3/2)⋅(2/3)=21=2
Thus, we have:
rB=2⋅rA
The solar constant (intensity of sunlight) at a distance r from the sun is inversely proportional to the square of the distance from the sun:
I∝1r2
Thus, the ratio of the solar constants IA and IB for planets A and B can be expressed as:
IAIB=r2Br2A
Substituting rB=2rA:
IAIB=(2rA)2r2A=4r2Ar2A=4
The ratio of the solar constant for planet A to that of planet B is:
IAIB=4
Topper's Solved these Questions
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS
106 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- TEST SERIESBOOK - RESONANCE ENGLISHCHAPTER - TEST SERIESEXERCISE - PHYSICS
130 Videos
Similar Questions
Knowledge Check
A planet revolves in elliptical orbit around the sun. (see figure). The linear speed of the planet will be maximum at
 AABBCCDD
AABBCCDD
A planet revolves in elliptical orbit around the sun. (see figure). The linear speed of the planet will be maximum at

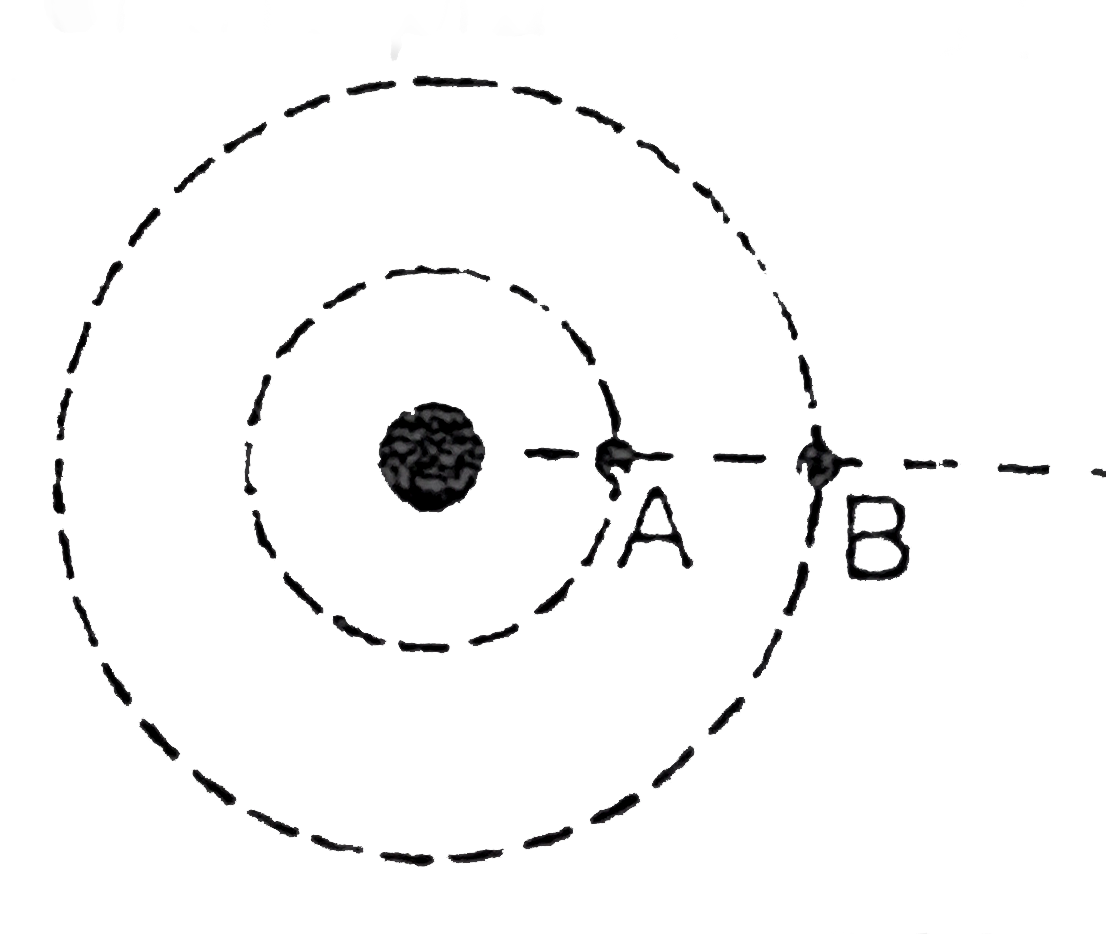
View SolutionTwo planets A and B travel counter clockwise is circular orbits around a fixed star. The radii of their orbits are in the ratio 1:4. At some time, they are aligned as shown in the figure, making a straight line with the star. After a certain time, planet A comes back to its initial positio completing one full circle about the star in the same time, angular displacement of the planet B is :
View SolutionConsider a planet moving in an elliptical orbit round the sun. The work done on the planet by the gravitational force of the sun
View SolutionConsider a planet moving in an elliptical orbit round the sun. The work done on the planet by the gravitational force of the sun
View SolutionTwo satellites of masses M and 16 M are orbiting a planet in a circular orbitl of radius R. Their time periods of revolution will be in the ratio of
View SolutionTwo satellites A and B go around a planet in circular orbits of radii 4 R and R respectively. If the speed of the satellite A is 3 V, then the speed of the satellite B will be
View SolutionThe distance of the planet Jupiter from the Sun is 5.2 times that of the Earth. Find the period of Jupiter's revolution around the Sun.
View Solution
