A point charge is kept at point the flux through the inclined surface of cone is . Now another charge is also placed at point . If net flux through the inclined surface is Find . 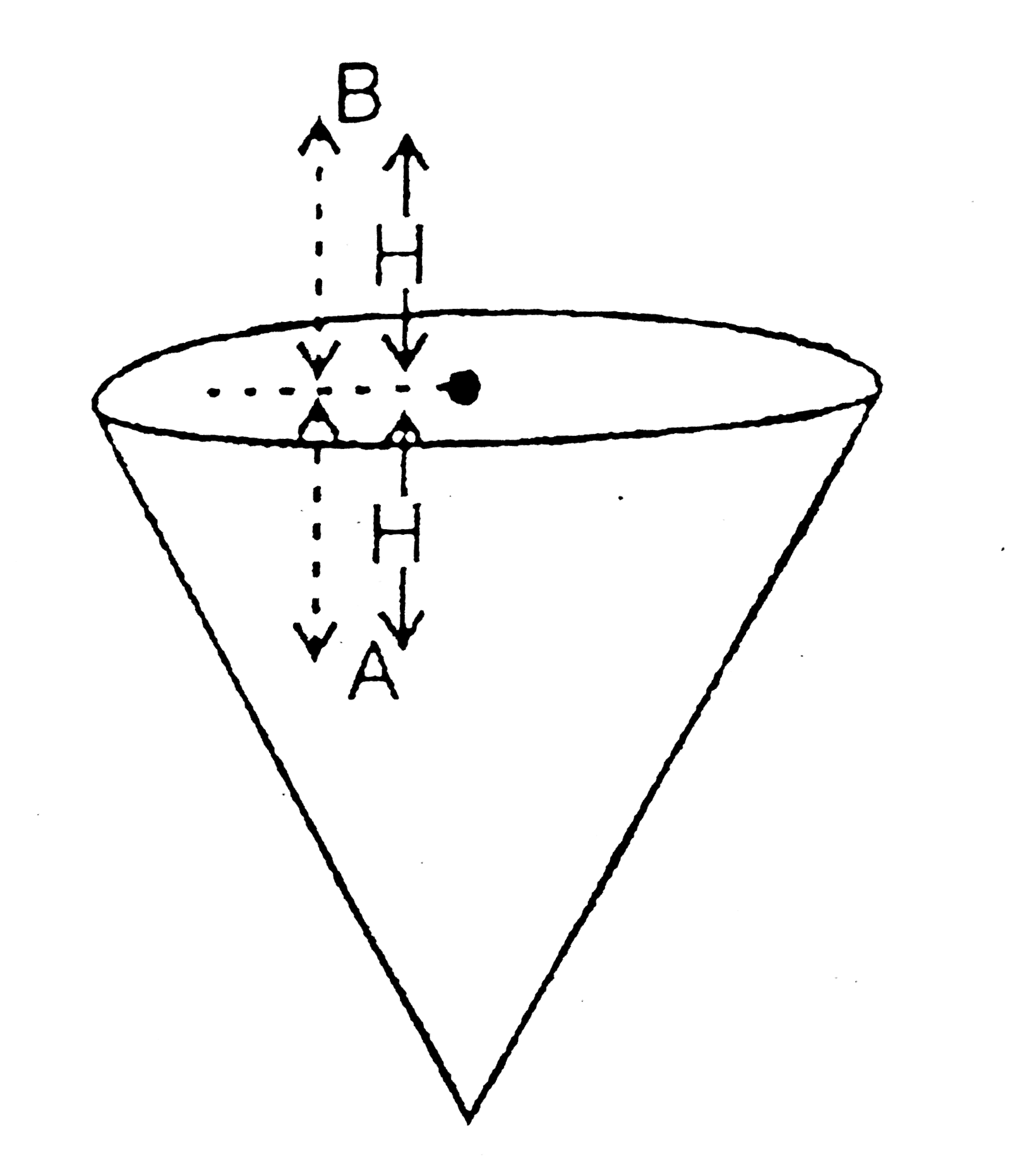
The correct Answer is:1
Topper's Solved these Questions
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS
106 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- TEST SERIESBOOK - RESONANCE ENGLISHCHAPTER - TEST SERIESEXERCISE - PHYSICS
130 Videos
Similar Questions
If the charge is placed at the center of one side, flux through the cube is qnε0 where .n. is
View SolutionA point charge .Q. is placed at a point inside the cone as shown. The flux due to the charge through the curved surface is given as 2Q3ε0. Now another charge .Q. is placed vertically above at the same distance from the base. The flux through the curved surface due to both charges is .

View SolutionA point charge Q is placed at the corner of a square of side a. Find the flux through the square.
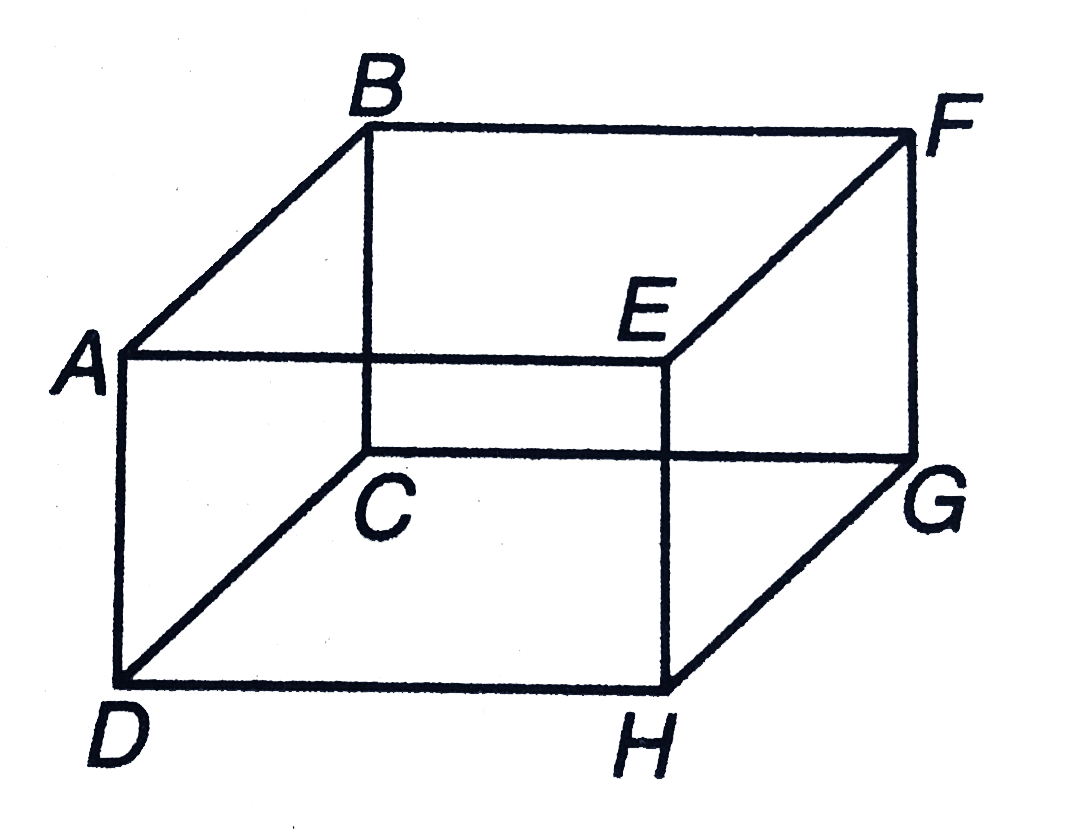
View SolutionA charge q is placed at point D of the cube. Find the electric flux passing through the EFGH and face AEHD.
View SolutionWhen a 10 μC charge is enclosed by a closed surface, the flux passing through the surface is ϕ. Now another 10 µC charge is placed inside the closed surface, then the flux passing through the surface is ______.
View SolutionFigure shown a closed surface which intersects a conducting sphere. If a positive charge is placed at the point P, the flux of the electric field through the closed surface

View SolutionIf a point charge q is placed at one corner of a cube, what is the flux linked with the cube?
View SolutionFind the flux of point charge q through the square surface ABCD as shown
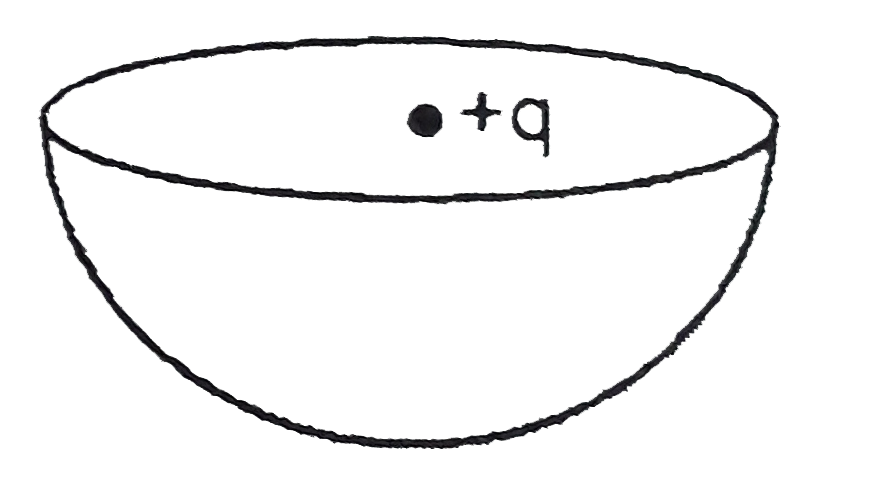
View SolutionA point charge +q is placed at the centre of curvature of a hemisphere. Find flux through the hemispherical surface.
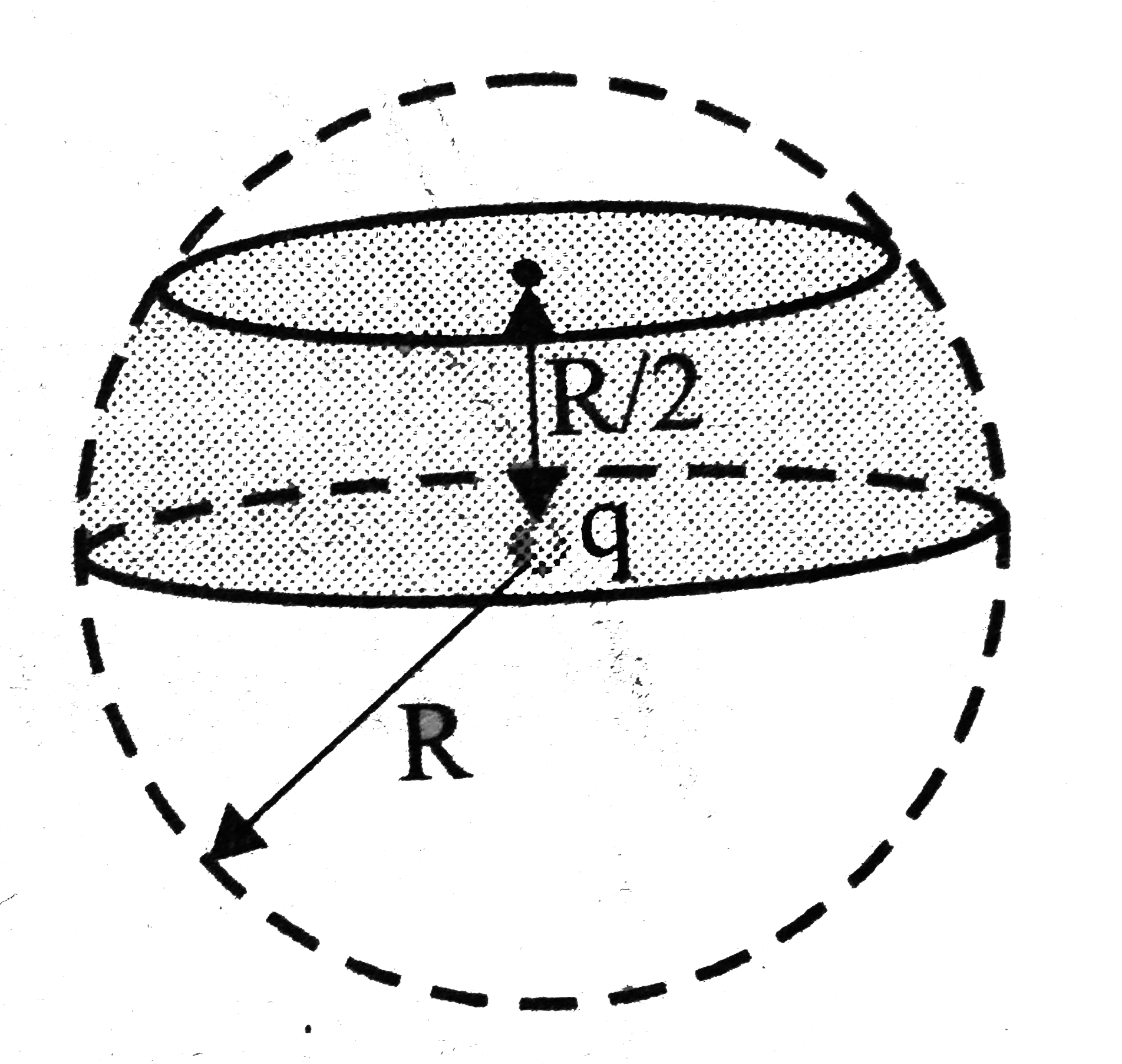
View SolutionFlux passing through the shaded surface of a sphere when a point charge q is placed at the center is (radius of the sphere is R)
View Solution
