Radiowaves of wavelength 3m are incident on a rectangular hole of width 5m. A screen is placed at a distance 200m from the hole. The width of the central is 100bm. Then find b.
The correct Answer is:3
To solve the problem, we need to find the value of b given the parameters of the setup involving radio waves and a rectangular hole.
1. Identify the parameters:
- Wavelength of the radio waves, λ=3m
- Width of the rectangular hole, d=5m
- Distance from the hole to the screen, L=200m
- Width of the central maximum, W=100bm
2. Understanding the formation of minima:
- The positions of the minima in a single slit diffraction pattern are given by the formula:
dsinθ=nλ
- For small angles, sinθ≈θ (in radians), hence:
dθ=nλ
3. Determine the angular spread for the first minima:
- The first minima occur at n=1 on either side of the central maximum. Thus, the angular spread for the first minima on either side is:
θ=λd
4. Calculate the angular spread:
- Substituting the values:
θ=3m5m=0.6radians
5. Calculate the linear width of the central maximum:
- The width of the central maximum is the distance between the first minima on either side, which is:
W=2×L×θ
- Substituting the values:
W=2×200m×0.6=240m
6. Relate the width of the central maximum to b:
- We know that the width of the central maximum is also given by:
W=100b
- Setting the two expressions for W equal gives:
100b=240
7. Solve for b:
- Dividing both sides by 100:
b=240100=2.4
Final Answer:
The value of b is 2.4.
---
- Wavelength of the radio waves, λ=3m
- Width of the rectangular hole, d=5m
- Distance from the hole to the screen, L=200m
- Width of the central maximum, W=100bm
- The positions of the minima in a single slit diffraction pattern are given by the formula:
dsinθ=nλ
- For small angles, sinθ≈θ (in radians), hence:
dθ=nλ
- The first minima occur at n=1 on either side of the central maximum. Thus, the angular spread for the first minima on either side is:
θ=λd
- Substituting the values:
θ=3m5m=0.6radians
- The width of the central maximum is the distance between the first minima on either side, which is:
W=2×L×θ
- Substituting the values:
W=2×200m×0.6=240m
- We know that the width of the central maximum is also given by:
W=100b
- Setting the two expressions for W equal gives:
100b=240
- Dividing both sides by 100:
b=240100=2.4
The value of b is 2.4.
Topper's Solved these Questions
- TEST PAPERSBook:RESONANCE ENGLISHChapter:TEST PAPERSExercise:PART - II PHYSICS
Explore 106 Videos
- SIMPLE HARMONIC MOTION Book:RESONANCE ENGLISHChapter:SIMPLE HARMONIC MOTION Exercise:Advanced Level Problems
Explore 13 Videos
- TEST SERIESBook:RESONANCE ENGLISHChapter:TEST SERIESExercise:PHYSICS
Explore 130 Videos
Similar Questions
Monochromatic light of wavelength 580 nm is incident on a slit of width 0.30 mm. The screen is 2m from the slit . The width of the central maximum is
View SolutionA light of wavelength 6000Å is incident on a single slit . First minimum is obtained at a distance of 0.4 cm from the centre . If width of the slit is 0.3 mm, the distance between slit and screen will be
View SolutionLight of wavelength 6000Å is incident on a slit of width 0.30 mm. The screen is placed 2 m from the slit. Find (a) the position of the first dark fringe and (b). The width of the central bright fringe.
View SolutionLight of wavelength 6328Å is incident normally on a slit having a width of 0.2mm. The distance of the screen from the slit is 0.9m. The angular width of the central maximum is
View SolutionLight of wavelength λ=5000Å falls normally on a narrow slit. A screen is placed at a distance of 1m from the slit and perpendicular to the direction of light. The first minima of the diffraction pattern is situated at 5mm from the centre of central maximum. The width of the slit is
View SolutionPlane sound waves of wavelength 0.12 m are incident on two narrow slits in a box nonreflecting walls, as shown. At a distance of 5.0 m from the center of the slits, a first-order maximum occurs at point P, which is 3.0 m from the central maximum. The distance between the slits is most nearly.
_E01_040_Q01.png) .
.
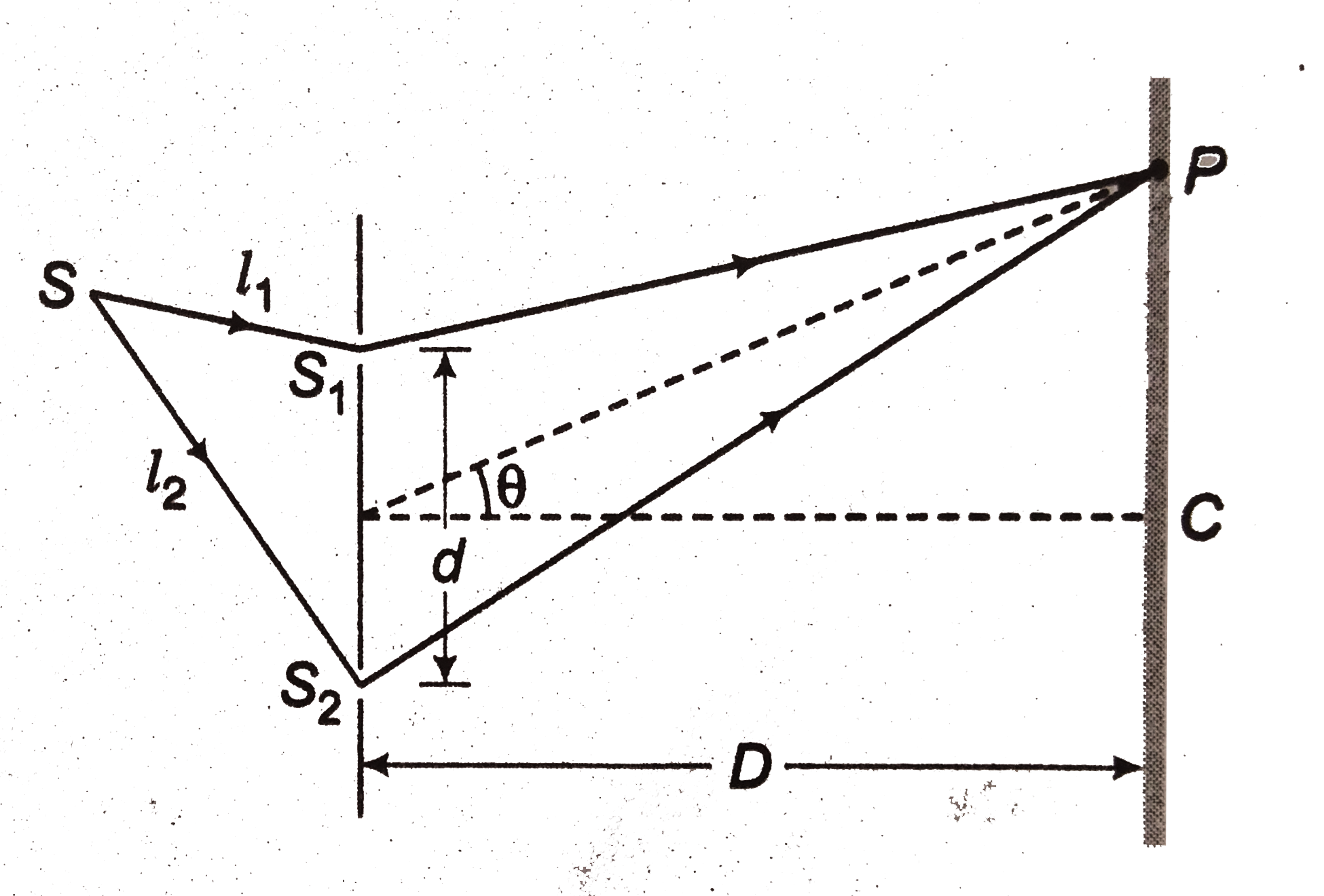
View SolutionIn a Young's double slit experiment, the light sources is at distance l1=20μm and l2=40μm form the main slit. The light of wavelength lambda = 500nm is incident on slits separated at a distance 10μm. A screen is placed at a distance D = 2 away from the slits as shown in figure. Find
(a) the values of θ relative to the central line where maxima appear on the screen?
(b) how many maxima will appear on the screen?
what should be the minimum thickness of a slab of refractive index 1.5 placed on the path of one of the ray so that minima occurs at C ?
 .
.
View SolutionLight of wavelength 5000 Å is incident on a slit of width 0.1 mm. Find out the width of the central bright line on a screen distance 2m from the slit?
View SolutionThe light of wavelength 6328 oA is incident on a slit of width 0.1 mm perpendicularly situated at a distance of 9 m and the central maxima between two minima, the angular width is approximately:
View SolutionA parallel beam of monochromatic light of wavelength 500 nm is incident normally on a rectangular slit. The angular width of the central fringe of Fraunhofer diffraction is found to be 60∘. Find the width of the slit in metre.
View Solution