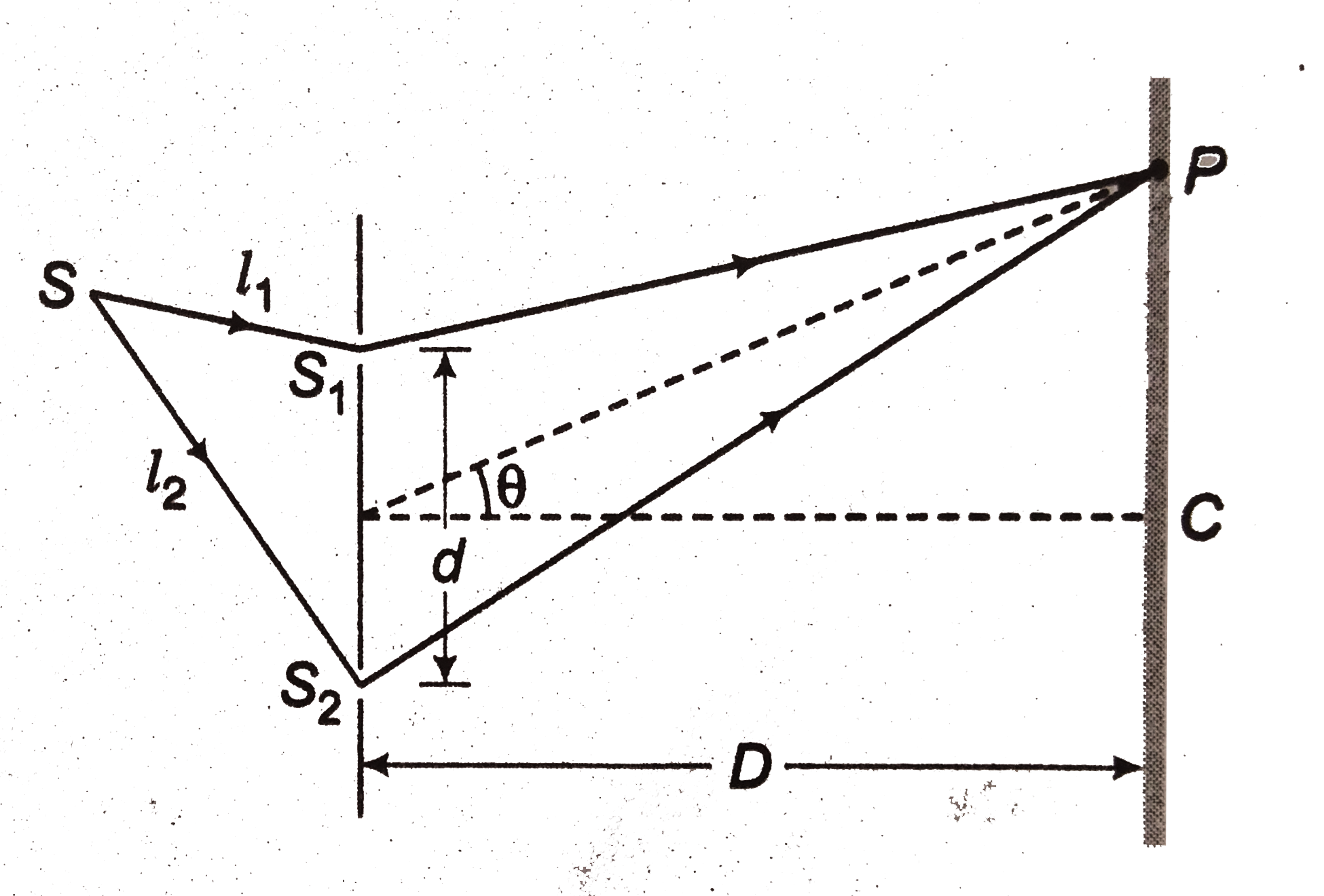
In a Young's double slit experiment, the light sources is at distance l1=20μm and l2=40μm form the main slit. The light of wavelength lambda = 500nm is incident on slits separated at a distance 10μm. A screen is placed at a distance D = 2 away from the slits as shown in figure. Find
(a) the values of θ relative to the central line where maxima appear on the screen?
(b) how many maxima will appear on the screen?
what should be the minimum thickness of a slab of refractive index 1.5 placed on the path of one of the ray so that minima occurs at C ?
 .
.
(a) The optical path difference between the beams arriving at P,
Δx=(l2−l1)+dsinθ
The condition for maximum intensity is [n=0,±1,±2………….]
Δx=nλ
Thus, sinθ=1d[Δx−(l2−l1)]=1d[nλ−(l2−l1)]
=110×(10−6)[n×500×10−9×20×10−6]
=2[(n40)−1]
Hence, θ=sin−1[2((n40)−1)]
(b)|sinθ|≤1
∴(−1≤2[(n40)−1]≤1)
or −20≤(n−40)≤20
or 20≤n≤60
Hence, number of maximas = 60-20 = 40
(c) At C, phase difference ϕ=2πλ(l2−l1)=(2π500×10−9)(20×10−6)
=80π
Hence, maximum intensity will appear at C. For minimum intensity at C,
(μ−1)t=λ2
or l t=λ2(μ−1)=500×10−92×0.5=500nm .
Topper's Solved these Questions
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Exercise 32.1
3 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Exercise 32.2
6 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Example Type 6
3 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
Similar Questions
In a Young's double slit experiment, the slit separation is 1mm and the screen is 1m from the slit. For a monochromatic light of wavelength 500nm, the distance of 3rd minima from the central maxima is
View SolutionLight of wavelength λ is incident on a slit of width d and distance between screen and slit is D. Then width of maxima and width of slit will be equal if D is
View Solution
Knowledge Check
In a double slit experiment, the distance between the slits is d. The screen is at a distance D from the slits. If a bright fringe is formed opposite to one of the slits, its order is
AdλBλ2dDCD22λDDd22Dλ
In Young's double-slit experiment, the slit separation is 0.5 mm and the screen is 0.5 m away from the slit. For a monochromatic light of wavelength 500 nm, the distance of 3rd maxima from the 2nd minima on the other side of central maxima is
View SolutionIn a double-slit experiment, the slits are separated by a distance d and the screen is at a distance D from the slits. If a maximum is formed just opposite to each slit, then what is the order or the fringe so formed?
View SolutionIn a Young's double slit experiment, the fringe width will remain same, if ( D = distance between screen and plane of slits, d = separation between two slits and λ = wavelength of light used)
View SolutionIn Yonung's double-slit experiment, two slits which are separated by 1.2 mm are illuminated with a monochromatic light of wavelength 6000Å The interference pattern is observed on a screen placed at a distance of 1 m from the slits. Find the number of bright fringes formed over 1 cm width on the screen.
View SolutionLight of wavelength 6000A0 is incident on a single slit. The first minimum of the diffraction pattern is obtained at 4 mm from the centre. The screen is at a distance of 2m from the slit. The slit width will be
View SolutionIn Young.s double slit experiment, blue-green light of wavelength 500nm is used. The slits are 1.20mm apart, and the viewing screen is 5.40m away from the slits. What is the fringe width.
View SolutionIn Young's double slit experiment, the separation between the slits is halved and the distance between the slits and the screen is doubled. The fringe width is
View Solution
