A dipole is kept near an infinite line of charge. If the net force on the dipole is zero, then its dipolse moment is
parallel to the line of charge
along the perpendicular drawn from the dipole to the line of charge
along the normal to the plane formed by the above two lines
net force onn a dipole kept near an infinte line of charge can never be zero
The correct Answer is:A
To solve the problem of a dipole placed near an infinite line of charge with the condition that the net force on the dipole is zero, we can follow these steps:
1. Understanding the Dipole: A dipole consists of two equal and opposite charges (let's say +q and -q) separated by a distance 'd'. The dipole moment (p) is defined as:
p=q⋅d
where d is a vector pointing from the negative charge to the positive charge.
2. Force on a Dipole in an Electric Field: When a dipole is placed in an electric field E, it experiences a torque τ given by:
τ=p×E
and a net force F which can be expressed as:
F=qE+−qE−
where E+ is the field at the position of the positive charge and E− is the field at the position of the negative charge.
3. Electric Field due to an Infinite Line of Charge: The electric field E due to an infinite line of charge with linear charge density λ at a distance r from the line is given by:
E=λ2πϵ0r
This field points radially outward from the line of charge.
4. Condition for Zero Net Force: For the net force on the dipole to be zero, the forces acting on the positive and negative charges of the dipole must be equal in magnitude and opposite in direction. This occurs when the dipole is aligned such that the dipole moment p is parallel to the electric field E of the line charge.
5. Conclusion: Since the dipole moment must be parallel to the line of charge for the net force to be zero, we can conclude that the dipole moment is:
Dipole moment p is parallel to the line of charge.
Final Answer:
The dipole moment is parallel to the line of charge.
p=q⋅d
where d is a vector pointing from the negative charge to the positive charge.
τ=p×E
and a net force F which can be expressed as:
F=qE+−qE−
where E+ is the field at the position of the positive charge and E− is the field at the position of the negative charge.
E=λ2πϵ0r
This field points radially outward from the line of charge.
Dipole moment p is parallel to the line of charge.
The dipole moment is parallel to the line of charge.
Topper's Solved these Questions
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS
106 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- TEST SERIESBOOK - RESONANCE ENGLISHCHAPTER - TEST SERIESEXERCISE - PHYSICS
130 Videos
Similar Questions
Knowledge Check
Which of the following has zero dipole moment?
A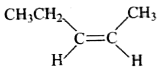 B
B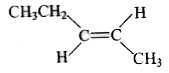 C
C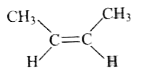 D
D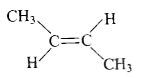
The molecule which does not exhibit net dipole moment is
ANH3BCHCl3CH2ODCCl4
Which of the following has zero dipole moment ?
View SolutionWhich of the following has zero dipole moment ?
View SolutionWhich of the following has no net dipole moment ?
View SolutionWhich of the following has zero dipole moment?
View SolutionThe dipole moment is zero for the compound
View SolutionWhich of the following is having zero dipole moment ?
View Solution
