A particle is projected from a point (0,1) of Y-axis (assume + Y direction vertically upwards) aiming towards a point (4,9). It fell on ground along x axis in 1 sec. Taking g=10ms2 and all coordinate in metres. Find the X-coordinate where it fell.
(3,0)
(4,0)
(2,0)
(2√5,0)
The correct Answer is:C
To solve the problem step by step, we will follow the kinematic equations of motion and the geometry of the projectile motion.
Step 1: Understand the problem
The particle is projected from the point (0, 1) aiming towards the point (4, 9). It falls on the ground (x-axis) after 1 second. We need to find the x-coordinate where it fell.
Step 2: Determine the angle of projection
The angle of projection can be determined using the coordinates of the two points. The vertical displacement (y-axis) is from 1 to 9, which is 9−1=8 meters, and the horizontal displacement (x-axis) is from 0 to 4, which is 4−0=4 meters.
Using the tangent function:
tanθ=Vertical DisplacementHorizontal Displacement=84=2
Thus, tanθ=2.
Step 3: Write the equation of motion in the vertical direction
The vertical motion can be described by the equation:
y=y0+uyt−12gt2
Where:
- y0=1 m (initial height)
- g=10m/s2 (acceleration due to gravity)
- t=1s (time of flight)
At the time of falling to the ground, y=0:
0=1+uy(1)−12⋅10⋅(1)2
This simplifies to:
0=1+uy−5
uy=4m/s
Step 4: Relate uy to the initial velocity u and angle θ
The vertical component of the initial velocity is given by:
uy=usinθ
From the previous step, we found uy=4. Thus:
4=usinθ
Step 5: Find the horizontal component of the initial velocity
Using the relationship of tanθ:
tanθ=usinθucosθ=2
This implies:
usinθucosθ=2⇒4ucosθ=2
Thus:
ucosθ=2
Step 6: Find the x-coordinate where it fell
The x-coordinate at the time of falling can be calculated using:
x=ux⋅t=(ucosθ)⋅t
Substituting t=1:
x=(ucosθ)⋅1=2
Final Answer
The x-coordinate where the particle fell is 2m.
---
The particle is projected from the point (0, 1) aiming towards the point (4, 9). It falls on the ground (x-axis) after 1 second. We need to find the x-coordinate where it fell.
The angle of projection can be determined using the coordinates of the two points. The vertical displacement (y-axis) is from 1 to 9, which is 9−1=8 meters, and the horizontal displacement (x-axis) is from 0 to 4, which is 4−0=4 meters.
tanθ=Vertical DisplacementHorizontal Displacement=84=2
Thus, tanθ=2.
The vertical motion can be described by the equation:
y=y0+uyt−12gt2
Where:
- y0=1 m (initial height)
- g=10m/s2 (acceleration due to gravity)
- t=1s (time of flight)
0=1+uy(1)−12⋅10⋅(1)2
This simplifies to:
0=1+uy−5
uy=4m/s
The vertical component of the initial velocity is given by:
uy=usinθ
From the previous step, we found uy=4. Thus:
4=usinθ
Using the relationship of tanθ:
tanθ=usinθucosθ=2
This implies:
usinθucosθ=2⇒4ucosθ=2
Thus:
ucosθ=2
The x-coordinate at the time of falling can be calculated using:
x=ux⋅t=(ucosθ)⋅t
Substituting t=1:
x=(ucosθ)⋅1=2
The x-coordinate where the particle fell is 2m.
Topper's Solved these Questions
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type
51 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - Multiple correct Answer Type
54 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer
5 Videos
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Single Answer Correct Type
22 Videos
Similar Questions
Two lines cut on the axis of x intercepts 4and−4 and on the axis of y intercepts 2 and 6 respectively. Find the coordinates of their point of intersection.
View Solution
A particle is projected from the horizontal x-z plane, in vertical x-y plane where x-axis is horizontal and positive y-axis vertically upwards. The graph of y coordinate of the particle v/s time is as shown . The range of the particle is √3. Then the speed of the projected particle is:
View SolutionA particle of mass m is released in vertical plane from a point P at x=x0 on the x-axis. It falls vertically parallel to the y-axis. Find the torque τ acting on the particle at a time about origin.

View SolutionTwo particles are moving along x-axis. Particle-1 starts from x=−10m with velocity 4m/s along negative x-direction and acceleration 2m/s2 along positive x-direction. Particle-2 starts from x=+2m with velocity 6m/s along positive x-direction and acceleration 2m/s2 along negative x-direction.
(a) Find the time when they collide.
(b) Find the x-coordinates where they collide. Both start simultaneously.
View SolutionA train is moving with a constant speed of 10m/s in a circle of radius 16πm. The plane of the circle lies in horizontal x-y plane. At time t = 0, train is at point P and moving in counter- clockwise direction. At this instant, a stone is thrown from the train with speed 10m/s relative to train towards negative x-axis at an angle of 37∘ with vertical z-axis . Find
(a) the velocity of particle relative to train at the highest point of its
trajectory.
(b) the co-ordinates of points on the ground where it finally falls and that of the hightest point of its trajectory. (Take g =10m/s2,sin37∘=35
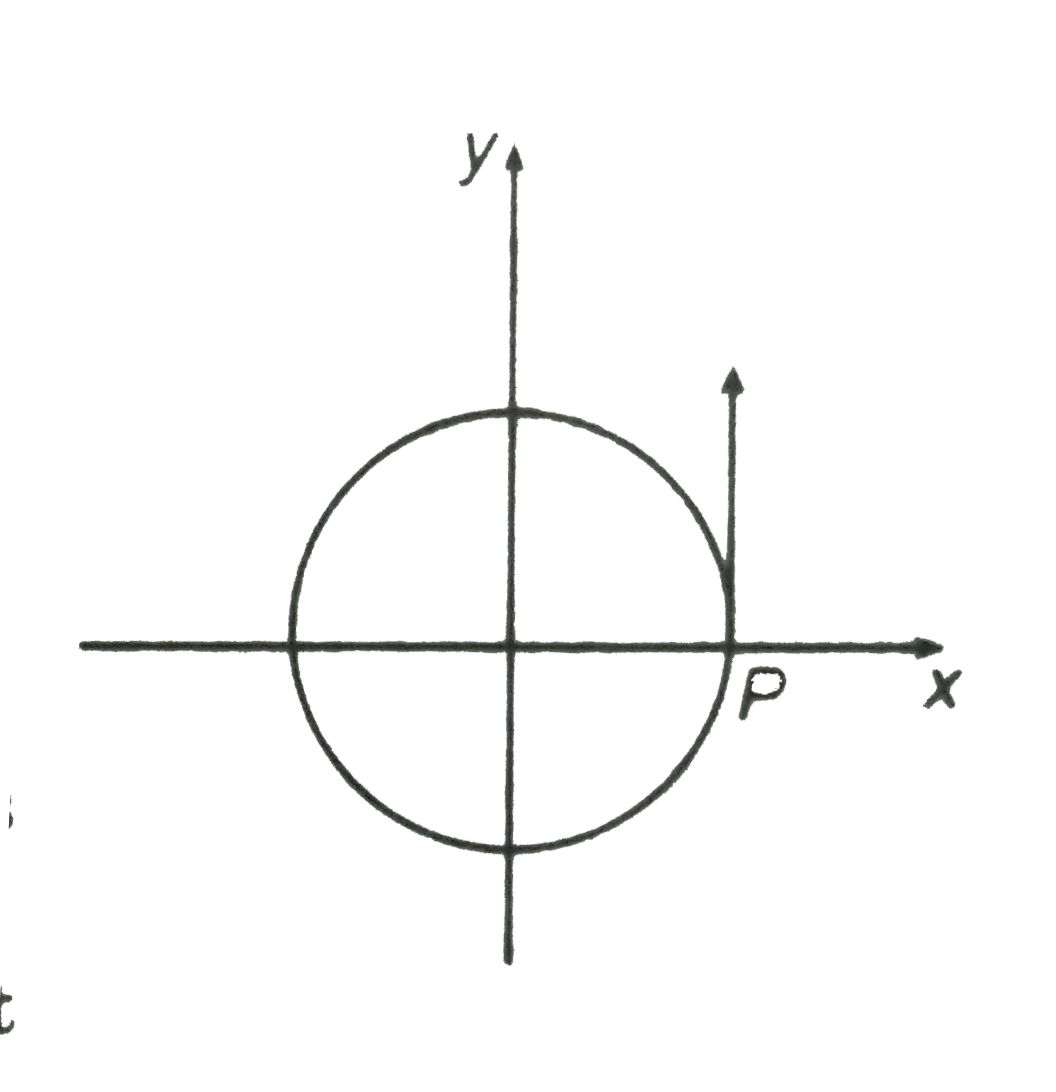
View SolutionA particle is projected horizontally with spee u from point A, which is 10 above the ground . If the particle hits the inclined perpendicularly at point B. [ g =10m/s2]
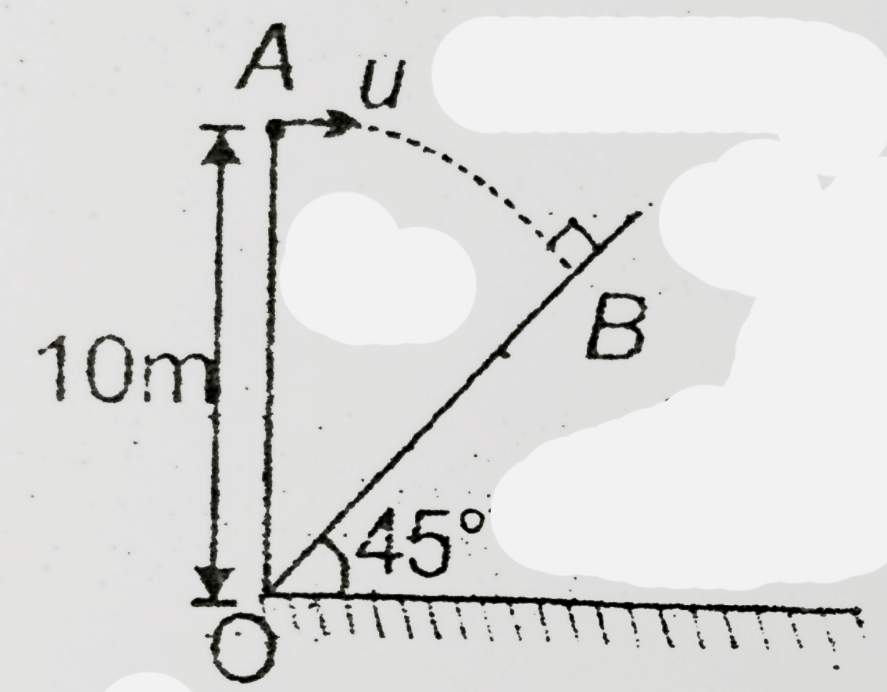
Find the length OB along the inclined plane
View SolutionA particle is projected vertically upwards from ground with velocity 10 m/s. Find the time taken by it to reach at the highest point ?
View SolutionTaking horizontal ground as xz- plane and y-axis vertically upwards. A particle is projected from the horizontal ground from a point whose position vector is ˆi+ˆk meter with initial velocity 2i +3j+k from the origin when it hits ground is given by 15[11ˆi+nˆk], find n. (Take g=10m/s2 in negative y - direction)
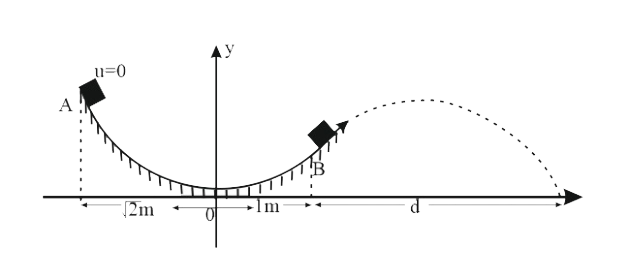
View SolutionA small body is released from point A of smooth parabolic path y=x2, where y is vertical axis and x is horizontal axis at ground, as shown. The body leaves the surface from point B. If g=10ms−2 then what is the value of d (in m)?
View SolutionFind the equation of a curve passing through the point (0,1) if the slope of the tangent to the curve at any point (x,y) is equal to the sum of the x coordinate (abscissa) and the product of the x coordinate and y coordinate (ordinate) of that point.
View Solution
