A uniform rod of thermal conductivity of 65J/m−s−∘C is surrounded by an insulator on its sides. One of its ends is put in a furnace, while the other end is kept exposed. If the temperature gradient of the rod is −75∘C/m, find the emissive power of the exposed end.
To solve the problem, we need to find the emissive power of the exposed end of a uniform rod given its thermal conductivity and the temperature gradient. Here’s a step-by-step solution:
Step 1: Understand the Given Data
We have the following information:
- Thermal conductivity of the rod, k=65J/m-s-°C
- Temperature gradient, ΔTΔx=−75°C/m
Step 2: Define the Emissive Power
The emissive power E can be defined in terms of the heat transfer per unit area and temperature:
E=QA⋅T
Where:
- Q is the heat transfer,
- A is the area,
- T is the temperature.
Step 3: Relate Heat Transfer to Temperature Gradient
According to Fourier's law of heat conduction, the rate of heat transfer QT can be expressed as:
QT=k⋅A⋅ΔTΔx
We can rearrange this to express emissive power E:
E=k⋅ΔTΔx
Step 4: Substitute the Known Values
Now, we substitute the known values into the equation:
- Take the magnitude of the temperature gradient: |ΔT/Δx|=75°C/m
- Substitute k=65J/m-s-°C and |ΔT/Δx|=75°C/m:
E=65⋅75
Step 5: Calculate the Emissive Power
Now we perform the multiplication:
E=65⋅75=4875W/m2
Final Answer
The emissive power of the exposed end of the rod is:
E=4875W/m2
---
We have the following information:
- Thermal conductivity of the rod, k=65J/m-s-°C
- Temperature gradient, ΔTΔx=−75°C/m
The emissive power E can be defined in terms of the heat transfer per unit area and temperature:
E=QA⋅T
Where:
- Q is the heat transfer,
- A is the area,
- T is the temperature.
According to Fourier's law of heat conduction, the rate of heat transfer QT can be expressed as:
QT=k⋅A⋅ΔTΔx
We can rearrange this to express emissive power E:
E=k⋅ΔTΔx
Now, we substitute the known values into the equation:
- Take the magnitude of the temperature gradient: |ΔT/Δx|=75°C/m
- Substitute k=65J/m-s-°C and |ΔT/Δx|=75°C/m:
E=65⋅75
Now we perform the multiplication:
E=65⋅75=4875W/m2
The emissive power of the exposed end of the rod is:
E=4875W/m2
Topper's Solved these Questions
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Single Correct
148 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Multiple Correct
25 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Exercise 1.3
18 Videos
- BASIC MATHEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.6
20 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Video
Similar Questions
The temperature gradient in a rod of 0.5m length is 80∘C/m. It the temperature of hotter end of the rod is 30∘C, then the temperature of the cooler end is
View SolutionA brass rod of length 1m is fixed to a vertical wall at one end, with the other end keeping free to expand. When the temperature of the rod is increased by 120∘C, the length increases by 3cm. What is the strain?
View SolutionOne end of a rod of length 20cm is inserted in a furnace at 800K The sides of the rod are covered with an insulating material and the other end emits radiation like a black body. The temperature of this end is 750K in the steady state The temperature of the surrounding air is 300K Assuming radiation to be the only important mode of energy transfer between the surrounding and the open end of the rod, find the thermal conductivity of the rod Stefan's constant σ=6.0×10−8Wm−2K−4 .
View SolutionA rod AB is 1m long. The temperature of its one end A is maintained at 100∘C and other end B at 10∘C, the temperature at a distance of 60 cm from point B is
View Solution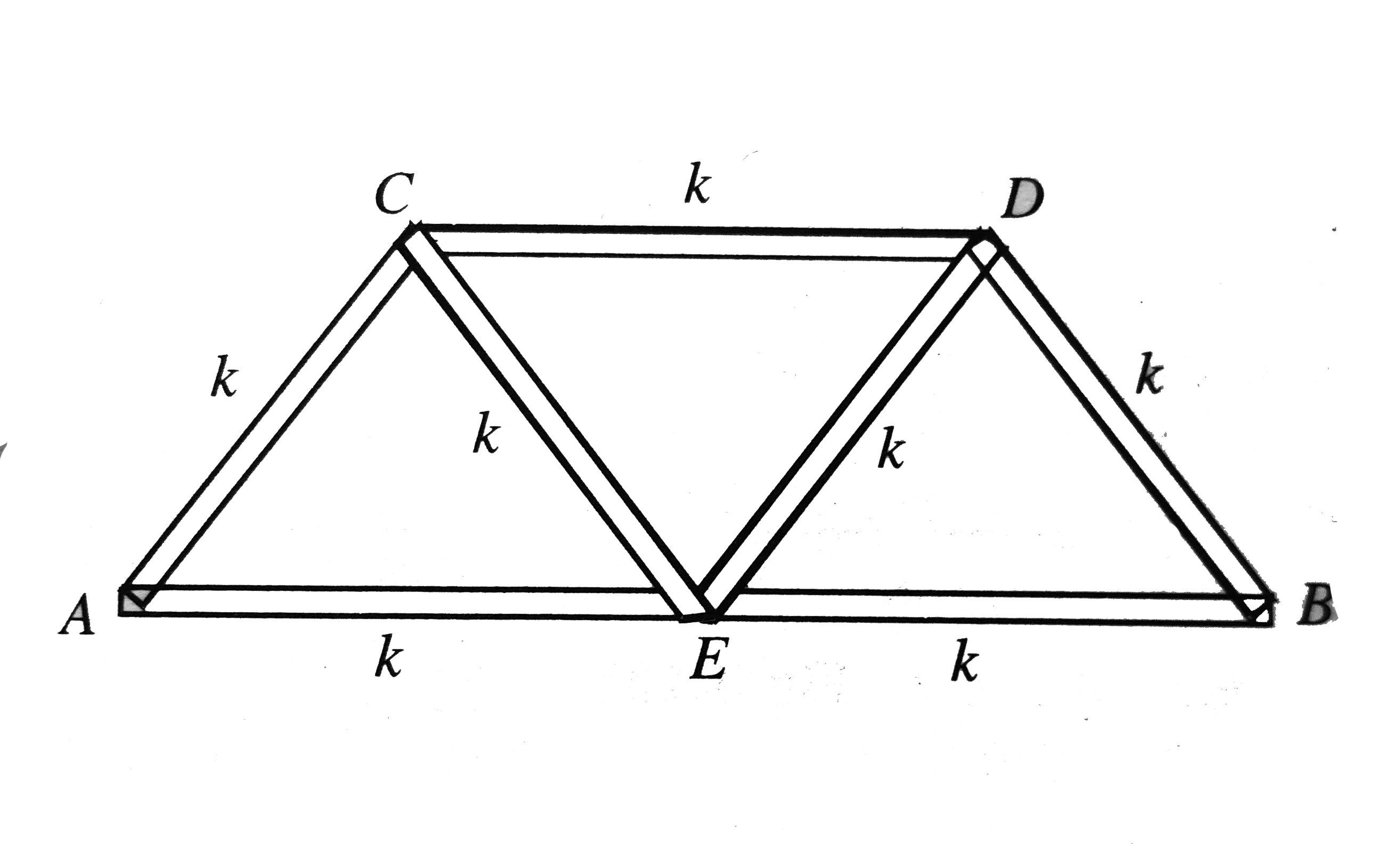
Seven identical rods of material of thermal conductivity k are connected as shown in Fig. All the rods are of identical length l and cross sectional area A If the one end A is kept at 100∘C and the other end is kept at 0∘C what would be the temperatures of the junctions C, D and E(θC,θ)D and θE) in the steady state?
View SolutionOne end of a rod length 20cm is inserted in a furnace at 800K. The sides of the rod are covered with an insulating material and the other end emits radiation like a blackbody. The temperature of this end is 750K in the steady state. The temperature of the surrounding air is 300K. Assuming radiation to be the only important mode of energy transfer between the surrounding and the open end of the rod, find the thermal conductivity of the rod. Stefan constant σ=6.0×10−1Wm−2K−4 .
View SolutionOne end of a rod length 20cm is inserted in a furnace at 800K. The sides of the rod are covered with an insulating material and the other end emits radiation like a blackbody. The temperature of this end is 750K in the steady state. The temperature of the surrounding air is 300K. Assuming radiation to be the only important mode of energy transfer between the surrounding and the open end of the rod, find the thermal conductivity of the rod. Stefan constant σ=6.0×10−1Wm−2K−4 .
View SolutionA thermal conductor is heated at one end. What is the theoretical value of its thermal conductivity in order that the other end also attains the same temperature?
View SolutionA thin copper rod of uniform cross section A square metres and of length L metres has a spherical metal sphere of radius r metre at Its one end symmetrically attached to the copper rod. The thermal conductivity of copper is K and the emissivity of the spherical surface of the sphere is ε.The free end of the copper rod is maintained at the temperature T kelvin by supplying thermal energy from a P watt source. Steady state conditions are allowed to be established while the rod is properly insulated against heat loss from its lateral surface. Surroundings are at 0∘C Stefan's constant=σW/m2K4.
After the steady state conditions are reached,the temperature of the spherical end of the rod, TS is
View SolutionA thin copper rod of uniform cross section A square metres and of length L metres has a spherical metal sphere of radius r metre at its one end symmetrically attached to the copper rod. The thermal conductivity of copper is K and the emissivity of the spherical surface of the sphere is ε.The free end of the copper rod is maintained at the temperature T kelvin by supplying thermal energy from a P watt source. Steady state conditions are allowed to be established while the rod is properly insulated against heat loss from its lateral surface. Surroundings are at 0∘C Stefan's constant=σW/m2K4.
After the steady state conditions are reached,the temperature of the spherical end of the rod, TS is
View Solution
