One end of a rod length 20cm is inserted in a furnace at 800K. The sides of the rod are covered with an insulating material and the other end emits radiation like a blackbody. The temperature of this end is 750K in the steady state. The temperature of the surrounding air is 300K. Assuming radiation to be the only important mode of energy transfer between the surrounding and the open end of the rod, find the thermal conductivity of the rod. Stefan constant σ=6.0×10−1Wm−2K−4 .
The correct Answer is:A, D
To solve the problem, we need to find the thermal conductivity of the rod given the conditions of heat transfer through radiation. Here’s a step-by-step solution:
Step 1: Understand the Heat Transfer Mechanism
The rod is in a furnace at 800 K, and one end of the rod is at 750 K, while the surrounding air is at 300 K. The only mode of heat transfer from the open end of the rod to the surroundings is through radiation.
Step 2: Set Up the Heat Transfer Equations
In the steady state, the heat conducted through the rod equals the heat radiated from the open end.
1. Heat Conducted (Q_conducted):
Qconducted=K⋅A⋅(Tf−T1)L
where:
- K = thermal conductivity of the rod
- A = cross-sectional area of the rod (we will see it cancels out)
- Tf = temperature of the furnace = 800 K
- T1 = temperature of the open end = 750 K
- L = length of the rod = 20 cm = 0.2 m
2. Heat Radiated (Q_radiated):
According to the Stefan-Boltzmann law:
Qradiated=ϵ⋅σ⋅A⋅(T41−T4s)
where:
- ϵ = emissivity (for a black body, ϵ=1)
- σ = Stefan-Boltzmann constant = 6.0×10−8W/m2K4
- Ts = temperature of the surroundings = 300 K
Step 3: Equate the Heat Conducted and Heat Radiated
Setting the two equations equal:
K⋅A⋅(800−750)0.2=ϵ⋅σ⋅A⋅(7504−3004)
Step 4: Simplify the Equation
Since the area A appears on both sides, we can cancel it out:
K⋅(800−750)0.2=σ⋅(7504−3004)
Step 5: Calculate the Values
1. Calculate the left side:
K⋅500.2=250K
2. Calculate the right side:
- First, calculate 7504 and 3004:
7504=316406250000and3004=8100000000
- Now calculate 7504−3004:
7504−3004=316406250000−8100000000=308306250000
- Now substitute into the equation:
K⋅250=6.0×10−8⋅308306250000
Step 6: Solve for K
K⋅250=6.0×10−8⋅308306250000
Calculating the right side:
K⋅250=18498.375
Now solve for K:
K=18498.375250=73.9935≈74W/m°C
Final Answer
The thermal conductivity of the rod is approximately 74 W/m°C.
The rod is in a furnace at 800 K, and one end of the rod is at 750 K, while the surrounding air is at 300 K. The only mode of heat transfer from the open end of the rod to the surroundings is through radiation.
In the steady state, the heat conducted through the rod equals the heat radiated from the open end.
Qconducted=K⋅A⋅(Tf−T1)L
where:
- K = thermal conductivity of the rod
- A = cross-sectional area of the rod (we will see it cancels out)
- Tf = temperature of the furnace = 800 K
- T1 = temperature of the open end = 750 K
- L = length of the rod = 20 cm = 0.2 m
According to the Stefan-Boltzmann law:
Qradiated=ϵ⋅σ⋅A⋅(T41−T4s)
where:
- ϵ = emissivity (for a black body, ϵ=1)
- σ = Stefan-Boltzmann constant = 6.0×10−8W/m2K4
- Ts = temperature of the surroundings = 300 K
Setting the two equations equal:
K⋅A⋅(800−750)0.2=ϵ⋅σ⋅A⋅(7504−3004)
Since the area A appears on both sides, we can cancel it out:
K⋅(800−750)0.2=σ⋅(7504−3004)
1. Calculate the left side:
K⋅500.2=250K
- First, calculate 7504 and 3004:
7504=316406250000and3004=8100000000
- Now calculate 7504−3004:
7504−3004=316406250000−8100000000=308306250000
- Now substitute into the equation:
K⋅250=6.0×10−8⋅308306250000
K⋅250=6.0×10−8⋅308306250000
Calculating the right side:
K⋅250=18498.375
Now solve for K:
K=18498.375250=73.9935≈74W/m°C
The thermal conductivity of the rod is approximately 74 W/m°C.
Topper's Solved these Questions
- HEAT TRANSFERBook:HC VERMA ENGLISHChapter:HEAT TRANSFERExercise:QUESTIONS FOR SHORT ANSWER
Explore 11 Videos
- HEAT TRANSFERBook:HC VERMA ENGLISHChapter:HEAT TRANSFERExercise:OBJECTIVE II
Explore 6 Videos
- HEAT AND TEMPERATUREBook:HC VERMA ENGLISHChapter:HEAT AND TEMPERATUREExercise:Objective 2
Explore 6 Videos
- INTRODUCTION TO PHYSICSBook:HC VERMA ENGLISHChapter:INTRODUCTION TO PHYSICSExercise:Question for short Answer
Explore 4 Videos
Similar Questions
One end of a rod of length 20cm is inserted in a furnace at 800K The sides of the rod are covered with an insulating material and the other end emits radiation like a black body. The temperature of this end is 750K in the steady state The temperature of the surrounding air is 300K Assuming radiation to be the only important mode of energy transfer between the surrounding and the open end of the rod, find the thermal conductivity of the rod Stefan's constant σ=6.0×10−8Wm−2K−4 .
View SolutionOne end of a metal rod is kept in a furnace. In steady state, the temperature of the rod
View SolutionOne end of a metal rod is kept in a furnace. In steady state, the temperature of the rod
View SolutionOn heating one end of a rod the temperature of the whole rod will be uniform when .
View SolutionOn heating one end of a rod the temperature of the whole rod will be uniform when .
View SolutionThe ratio of energies of emitted radiation by a black body at 600 K and 900 K , when the surrounding temperature is 300 K , is
View SolutionSteady state temperature are shown in the diagram. Find ratio of thermal conductivity k1k2 if length of all rods are same
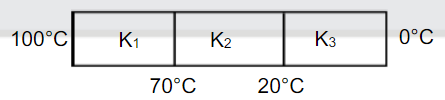
View Solution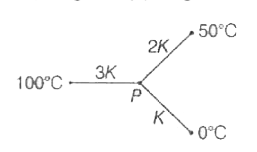
Three rods of the same dimension have thermal conductivity 3K , 2K and K. They are arranged as shown in the figure below
Then , the temperature of the junction in steady - state is
View SolutionThree rods of same dimensions have thermal conductivity 3K,2K and K They are arranged as shown in Then the temperature of the junction in steady state is
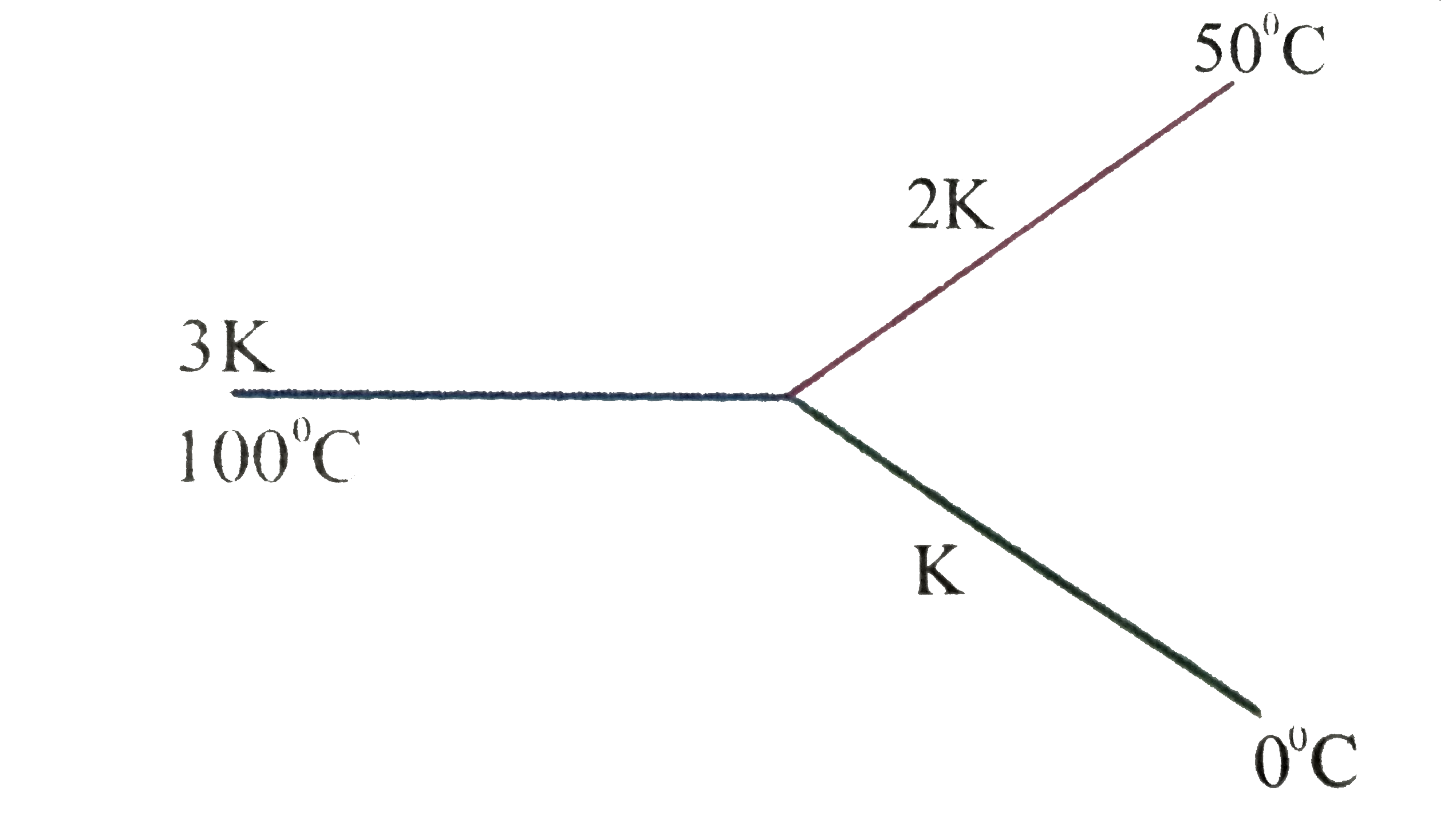 .
.
View SolutionWhich of the following cylindrical rods will conduct most heat, when their ends are maintained at the same steady temperature
View Solution