Question

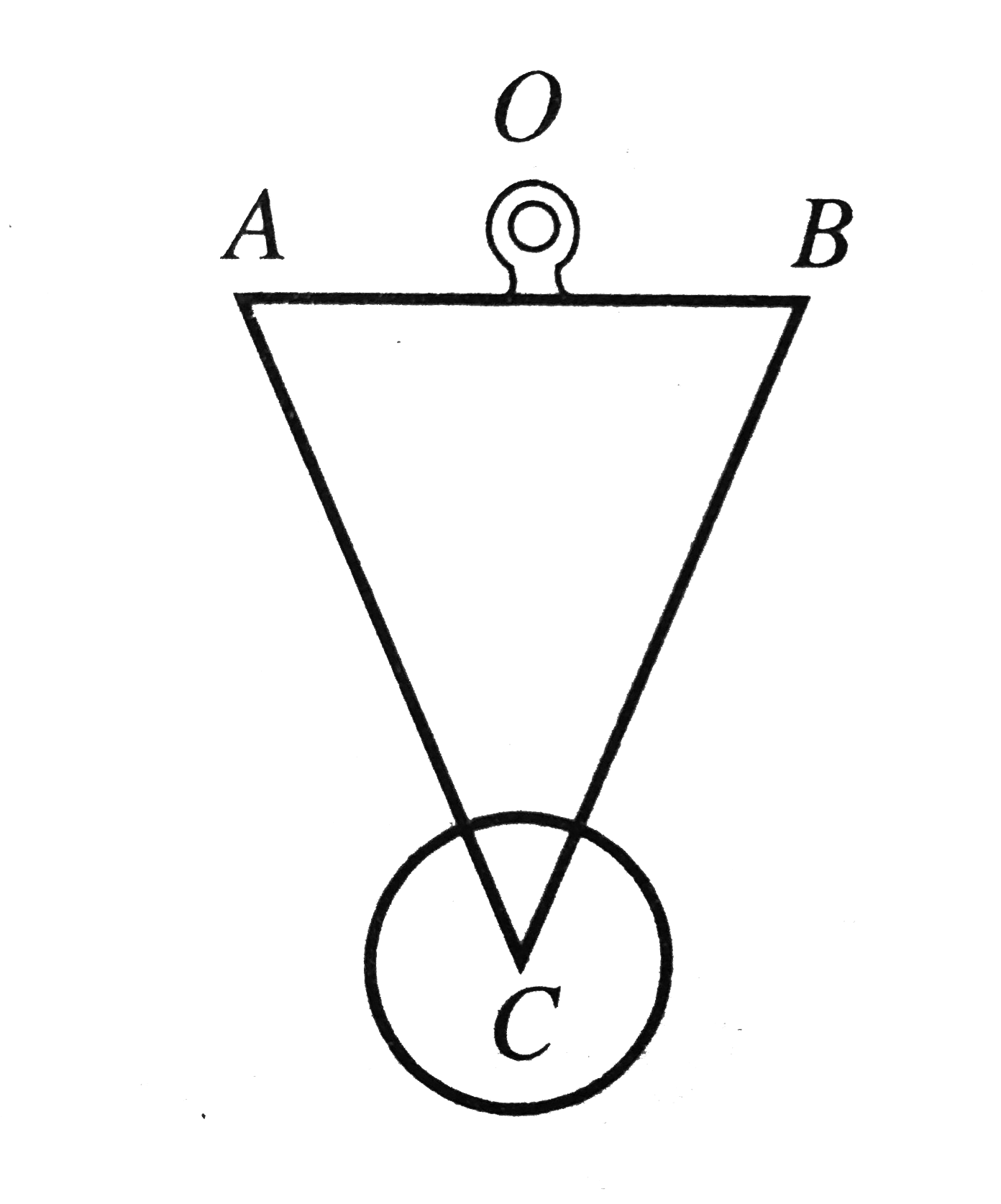
A compensated pendulum shown if Fig. is in the from of an isosceles Delta of base length $l_1=5$cm and coefficent of linar expansion ${\alpha }_1=18\times {10}^{-6}$ and side length $l_2$ and coefficient of linear expansion ${\alpha }_2=12\times {10}^{-6}$. find $l_2$ so the the distance of centre of mass of the bob from suspension centre O may remain the same at all the temperature.

Explanation

First we must know what is a compensated pendulum. We've dicussed that due to change in temperature the time period of a pendulum clock changes. Due to this, in pendulum clocks , to make a pendulum some specific metals are used which have very low coefficient of expansion so that the error intriduced in their time is very small. The other alternative of minimizing the error in time measurement, is to use compensated pendulum. This is a pendulum made up of two or more metals as shown in Fig.
In this case the distance of centre of mass from suspension point O is
`h=sqrt(l_2^2-(l_1^2)/(4))`
When temperature is changed by `Deltat`, new value of h will become
`h'=sqrt(l_2^2(1+alpha_2Deltat)^2-(l_1^2)/(4)(1+alpha_1Deltat)^2)`
As we require `h=h'`, we have
`l_2^2-(l_1^2)/(4)=l_2^2(1+2alpha_2Deltat)-(l_1^2)/(4)(1+2alpha_1Deltat)`
or `2alpha_2l_2^2=(l_1^2)/(2)alpha_1`
or `l_2=(l_1)/(2)sqrt((alpha_1)/(alpha_2))`.