A brass rod of length is fixed to a vertical wall at one end, with the other end keeping free to expand. When the temperature of the rod is increased by , the length increases by . What is the strain?
To find the strain in the brass rod, we can follow these steps:
Step 1: Understand the given information
- The original length of the brass rod (L) = 1 meter = 100 cm (since we will convert everything to centimeters for consistency).
- The change in length (ΔL) = 3 cm.
- The change in temperature (ΔT) = 120 °C (not directly needed for strain calculation but relevant for understanding thermal expansion).
Step 2: Recall the formula for strain
Strain (ε) is defined as the ratio of the change in length (ΔL) to the original length (L):
Strain(ϵ)=ΔLL
Step 3: Substitute the values into the formula
Now, substituting the values we have:
ϵ=3 cm100 cm=0.03
Step 4: Conclusion
The strain in the brass rod is 0.03.
Final Answer
The strain in the brass rod is 0.03.
---
- The original length of the brass rod (L) = 1 meter = 100 cm (since we will convert everything to centimeters for consistency).
- The change in length (ΔL) = 3 cm.
- The change in temperature (ΔT) = 120 °C (not directly needed for strain calculation but relevant for understanding thermal expansion).
Strain (ε) is defined as the ratio of the change in length (ΔL) to the original length (L):
Strain(ϵ)=ΔLL
Now, substituting the values we have:
ϵ=3 cm100 cm=0.03
The strain in the brass rod is 0.03.
The strain in the brass rod is 0.03.
Topper's Solved these Questions
- PROPERTIES OF SOLIDS AND FLUIDSBook:CENGAGE PHYSICS ENGLISHChapter:PROPERTIES OF SOLIDS AND FLUIDSExercise:Solved Examples
Explore 11 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBook:CENGAGE PHYSICS ENGLISHChapter:PROPERTIES OF SOLIDS AND FLUIDSExercise:Exercise 5.1
Explore 12 Videos
- NEWTON'S LAWS OF MOTION 2Book:CENGAGE PHYSICS ENGLISHChapter:NEWTON'S LAWS OF MOTION 2Exercise:Integer type
Explore 1 Video
- RIGID BODY DYNAMICS 1Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 1Exercise:Integer
Explore 11 Videos
Similar Questions
Two rods of equal cross sections, one of copper and the other of steel, are joined to form a composite rod of length 2.0 m at 20∘C, the length of the copper rod is 0.5 m. When the temperature is raised to 120∘C, the length of composite rod increases to 2.002m. If the composite rod is fixed between two rigid walls and thus not allowed to expand, it is found that the lengths of the component rods also do not change with increase in temperature. Calculate Young's moulus of steel. (The coefficient of linear expansion of copper, αc=1.6×10−5∘C and Young's modulus of copper is 1.3×1013N/m2).
View SolutionA rod of length / and radius r is held between two rigid walls so that it is not allowed to expand. If its temperature is increased, then the force developed in it is proportional to
View Solution
In the given figure, a rod is free at one end and other end is fixed. When we change the temperature of rod by Δθ, then strain produced in the rod will be
View Solution
In the given figure, a rod is free at one end and other end is fixed. When we change the temperature of rod by Δθ, then strain produced in the rod will be
View SolutionIf rod is initially compressed by Δl length then what is the strain on the rod when the temperature
(a) is increased by Δθ (b) is decreased by Δθ
View SolutionA thin rod of negligible mass and area of cross section S is suspended vertically from one end. Length of the rod is L0 at T∘C . A mass m is attached to the lower end of the rod so that when temperature of the rod is reduced to 0∘C its length remains L0 Y is the Young’s modulus of the rod and α is coefficient of linear expansion of rod. Value of m is :
View SolutionThe ends of a rod of length l and mass m are attached to two identical springs as shown in the figure. The rod is free to rotate about its centre O . The rod is depressed slightly at end A and released . The time period of the oscillation is
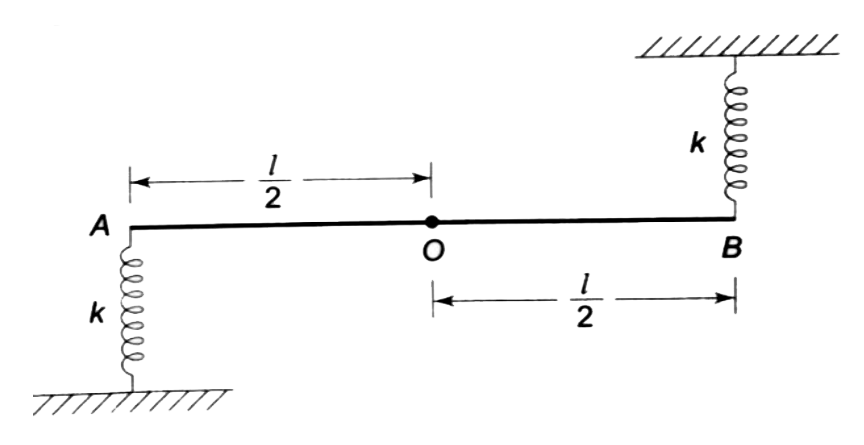
View SolutionA rod of length l(<2R) is kept inside a smooth sperical shell as shwon in fifure .mass of the rod is m
Keeping mass to be constant I flength of the rod is increased (but always <2R) the normal reaction at two ends of the rod

View SolutionA uniform rod of length L and mass M is pivoted freely at one end and placed in vertical position.
a. What is angular acceleration of the rod when it is at ann angle θ with the vertical?
b. What is the tangential linear acceleration of the free end when the rod is horizontal?
View SolutionA uniform rod of mass m and length l is fixed from Point A, which is at a distance l/4 from one end as shown in the figure. The rod is free to rotate in a vertical plane. The rod is released from the horizontal position.

What is the reaction at the hinge, when kinetic energy of the rod is maximum?
View Solution
