A body is hanging from a rigid support. by an inextensible string of length '1' . It is struck inelastically by an identical body of mass in with horizontal velocity v=√2gl the tension in the string increases just after the striking by
mg
3mg
2mg
none of these
The correct Answer is:C
To find the increase in tension in the string just after the inelastic collision, we can follow these steps:
Step 1: Understand the initial conditions
Initially, we have one body of mass m hanging from a rigid support by an inextensible string of length L. This body is at rest, so the tension in the string T0 is equal to the weight of the body:
T0=mg
Step 2: Analyze the collision
An identical body of mass m strikes the hanging body with a horizontal velocity v=√2gl. Since the collision is inelastic, the two bodies will stick together after the collision.
Step 3: Apply conservation of momentum
Before the collision, the momentum of the system is:
pinitial=mv
After the collision, the two bodies move together with a combined mass of 2m. Let v′ be their final velocity. By conservation of momentum:
mv=(2m)v′
Solving for v′:
v′=v2=√2gl2=√2gl2=√gl2
Step 4: Determine the forces acting on the combined mass
After the collision, the combined mass 2m will experience two forces:
1. The gravitational force acting downward: Fg=2mg
2. The tension T in the string acting upward.
Step 5: Apply centripetal force requirement
The tension in the string must provide the centripetal force required for the circular motion of the combined mass. The net force acting towards the center of the circular path is given by:
T−2mg=(2m)(v′)2L
Substituting v′=√gl2:
T−2mg=(2m)(gl2)L
This simplifies to:
T−2mg=mglL
Step 6: Solve for the tension T
Rearranging the equation gives:
T=2mg+mglL
Since L is the length of the string, we can replace glL with g (as l is equal to L):
T=2mg+mg=3mg
Step 7: Calculate the increase in tension
The initial tension was T0=mg. The increase in tension ΔT is:
ΔT=T−T0=3mg−mg=2mg
Final Answer
The increase in tension in the string just after the collision is:
ΔT=2mg
---
Initially, we have one body of mass m hanging from a rigid support by an inextensible string of length L. This body is at rest, so the tension in the string T0 is equal to the weight of the body:
T0=mg
An identical body of mass m strikes the hanging body with a horizontal velocity v=√2gl. Since the collision is inelastic, the two bodies will stick together after the collision.
Before the collision, the momentum of the system is:
pinitial=mv
After the collision, the two bodies move together with a combined mass of 2m. Let v′ be their final velocity. By conservation of momentum:
mv=(2m)v′
Solving for v′:
v′=v2=√2gl2=√2gl2=√gl2
After the collision, the combined mass 2m will experience two forces:
1. The gravitational force acting downward: Fg=2mg
2. The tension T in the string acting upward.
The tension in the string must provide the centripetal force required for the circular motion of the combined mass. The net force acting towards the center of the circular path is given by:
T−2mg=(2m)(v′)2L
Substituting v′=√gl2:
T−2mg=(2m)(gl2)L
This simplifies to:
T−2mg=mglL
Rearranging the equation gives:
T=2mg+mglL
Since L is the length of the string, we can replace glL with g (as l is equal to L):
T=2mg+mg=3mg
The initial tension was T0=mg. The increase in tension ΔT is:
ΔT=T−T0=3mg−mg=2mg
The increase in tension in the string just after the collision is:
ΔT=2mg
Topper's Solved these Questions
- CENTRE OF MASSBook:CENGAGE PHYSICS ENGLISHChapter:CENTRE OF MASSExercise:Multiple Correct
Explore 25 Videos
- CENTRE OF MASSBook:CENGAGE PHYSICS ENGLISHChapter:CENTRE OF MASSExercise:Assertion - Reasoning
Explore 2 Videos
- CENTRE OF MASSBook:CENGAGE PHYSICS ENGLISHChapter:CENTRE OF MASSExercise:Subjective
Explore 23 Videos
- CALORIMETRYBook:CENGAGE PHYSICS ENGLISHChapter:CALORIMETRYExercise:Solved Example
Explore 13 Videos
- DIMENSIONS & MEASUREMENTBook:CENGAGE PHYSICS ENGLISHChapter:DIMENSIONS & MEASUREMENTExercise:Integer
Explore 2 Videos
Similar Questions
A bob hangs from a rigid support by an inextensible string of length l. It is released from rest when string makes an agngle 60∘ with vertical . The speed of the bob at the lowest position is
View SolutionA bob hangs from a rigid support by an inextensible string of length l. If it is displaced through a distance l (from the lowest position) keeping the string straight and then released. The speed of the bob at the lowest position is
View SolutionA heavy particle hanging from a fixed point by a light inextensible string of length l is projected horizontally with speed (gl) . Then the speed of the particle and the inclination of the string to the vertical at the instant of the motion when the tension in the string equal the weight of the particle-
View SolutionTwo block of masses m1 and m2 are in equilibrium. The block m2 hangs from a fixed smooth pulley by an inextensible string that is fitted with a light spring of stiffness k as shown in fig. Neglecting friction and mass of the string, find the acceleration of the bodies just after the string S is cut.

View SolutionA heavy particle hanging from a fixed point by a light inextensible string of length l is projected horizonally with speed √gl . Find the speed of the particle and the inclination of the string to the vertical at the instant of the motion when the tension in the string is equal to the weight of the particle.
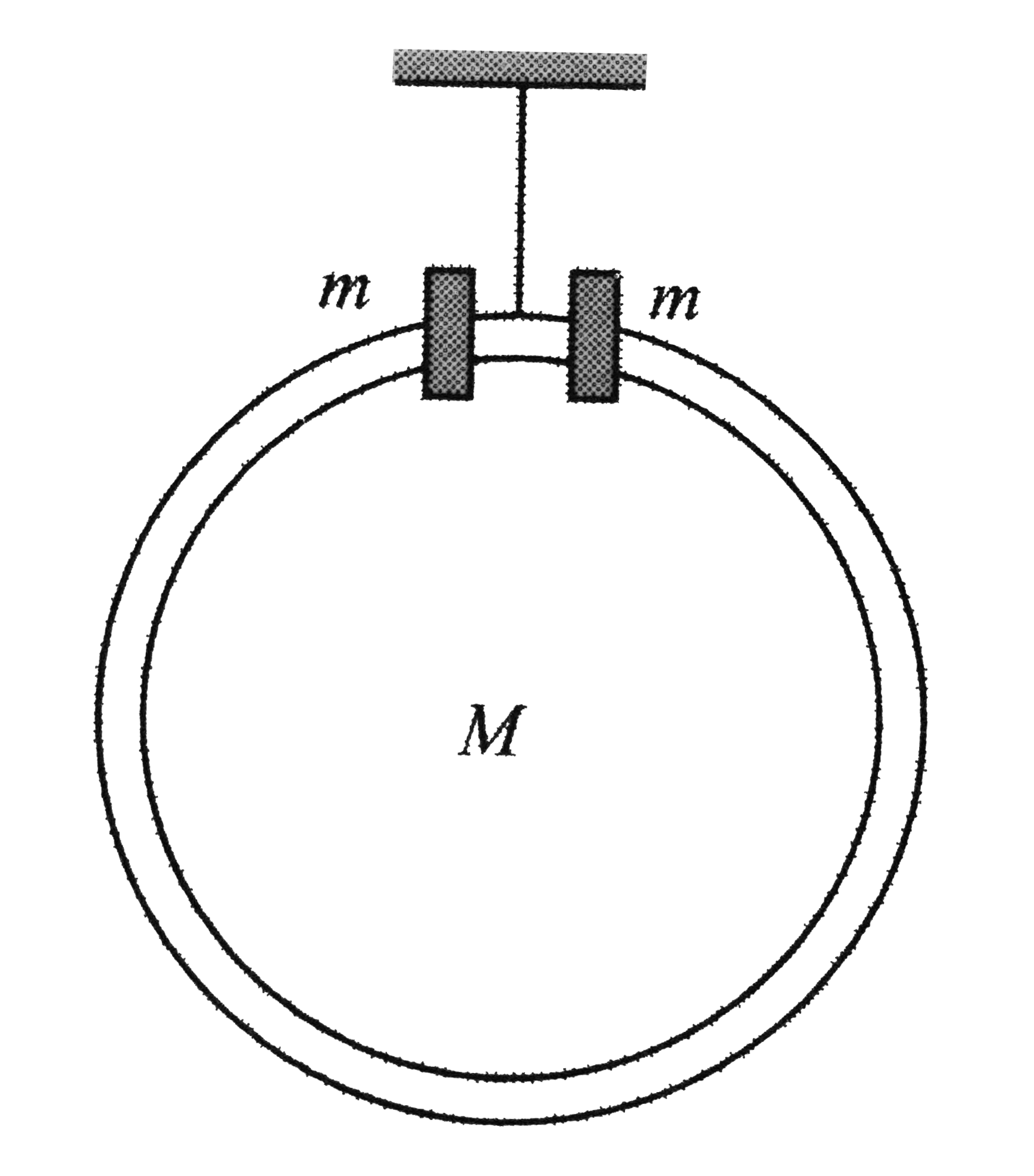
View SolutionA loop of mass M with two identical rings of mass m at its top hangs from a ceiling by an inextensible string. If the rings gently pushed horizontally in opposite directions, find the angular distance covered by each ring when the tension in the string vanishes for once during their motion.
View SolutionTwo particles A and B of equal masses lie close together on a horizontal table and are connected by a light inextensible string of length l. A is projected vertically upwards with a velocity √10gl. Find the velocity with which it reaches the table again.
View SolutionA particle suspended by a light inextensible thread of length l is projected horizontally from its lowest position with velocity √7gl/2. The string will slack after swinging through an angle equal to
View SolutionA bob of mass m suspended by a light inextensible string of length 'l' from a fixed point. The bob is given a speed of √6gl. Find the tension in the string when string deflects through an angle 120∘ from the vertical.
View SolutionA particle of mass m is attached to one end of a light inextensible string and the other end of the string is fixed in vertical plane as shown. Particle is given a horizontal velocity u=√52gl

The maximum angle made by the string with downward vertical is
View Solution