A particle oscillates simple harmonic motion with a period of 16s. Two second after crossing the equilibrium position its velocity becomes 1m/s. The amplitude is
π4m
8√2πm
8πm
4√2πm
The correct Answer is:B
ω=2πT=2π16=π8rad/s
At t=0, particle crosses the means position. Hence its velocity is maximum. So, velocity as a function of time can be written as
v=vmaxcosωt
or v=ωAcosωt
∴1=(π8)Acos(π8)(2)
=(π8√2)A
∴A=8√2πm
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBook:DC PANDEY ENGLISHChapter:SIMPLE HARMONIC MOTIONExercise:Level 2 More Than One Correct
Explore 8 Videos
- SIMPLE HARMONIC MOTIONBook:DC PANDEY ENGLISHChapter:SIMPLE HARMONIC MOTIONExercise:Level 2 Comprehension
Explore 2 Videos
- SIMPLE HARMONIC MOTIONBook:DC PANDEY ENGLISHChapter:SIMPLE HARMONIC MOTIONExercise:Level 1 Subjective
Explore 39 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBook:DC PANDEY ENGLISHChapter:SEMICONDUCTORS AND ELECTRONIC DEVICESExercise:More than One Option is Correct
Explore 3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBook:DC PANDEY ENGLISHChapter:SOLVD PAPERS 2017 NEET, AIIMS & JIPMERExercise:Solved paper 2018(JIPMER)
Explore 38 Videos
Similar Questions
A simple pendulum with a bob of mass m is suspended from the roof of a car moving with horizontal acceleration a
View SolutionA 2kg block hangs without vibrating at the bottom end of a spring with a force constant of 400N/m. The top end of the spring is attached to the ceiling of an elevator car. The car is rising with an upward acceleration of 5m/s2 when the acceleration suddenly ceases at time t=0 and the car moves upward with constant speed (g=10m/s2)
What is the angular frequencyof the block after the acceleration ceases ?
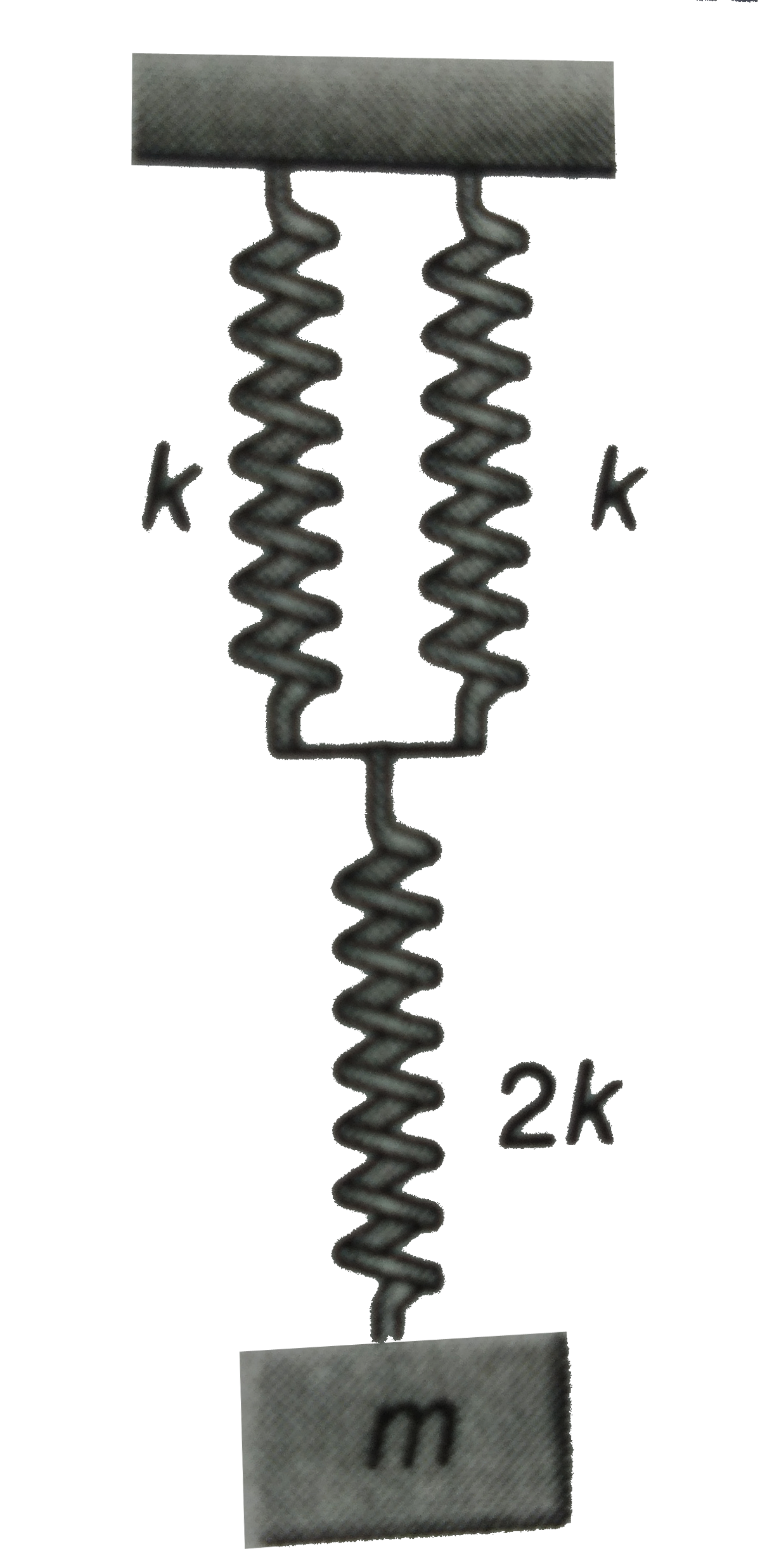
View SolutionFind the period of oscillation of the system shown in figure.
View SolutionWhen an electric field is applied across a semiconductor,
View SolutionIf a machine perform 4000 J output work and 1000 J is inside loss due to friction , then the find efficiency?
View SolutionThe maximum velocity a particle, executing simple harmonic motion with an amplitude 7 mm, 4.4 m//s. The period of oscillation is.
View SolutionA particle executes simple harmonic motion of period 16 s . Two seconds later after it passes through the center of oscillation its velocity is found to be 2 m//s . Find the amplitude.
View SolutionA particle executes simple harmonic motion with frequqncy 2.5Hz and amplitude 2m. The speed of the particle 0.3s after crossing, the equilibrium position is
View SolutionA particle oscillates simple harmonic motion with a period of 16 s . Two second after crossing the equilibrium position its velocity becomes 1 m//s . The amplitude is
View SolutionA particle performing SHM starts equilibrium position and its time period is 16 seconds. After 2 seconds its velocity is πm/s. Amplitude of oscillation is
(cos45∘=1√2)
View SolutionThe maximum velocity of a particle executing simple harmonic motion with an amplitude 7 mm is 4.4 m/s. The period of oscillation is :
View SolutionA simple harmonic oscillator has a period of 0.01 s and an amplitude of 0.2 m. The magnitude of the velocity in m/s at the centre of oscillation is……………….
View Solutionएक कण 16 सेकण्ड आवर्तकाल के साथ सरल आवर्त गति कर रहा है। t=2 सेकण्ड पर कण माध्य स्थिति से गुजरता है तथा t=1 सेकण्ड पर इसका वेग 2 मी/से हो जाता है। इस गति में आयाम मीटर में है
View SolutionA particle performing SHM starts equilibrium position and its time period is 16 seconds. After 2 seconds its velocity is pi m//s . Amplitude of oscillation is (cos 45^(@) = 1/(sqrt(2)))
View SolutionA particle executes simple harmonic motion of period 16 s . Two seconds later after it passes through the center of oscillation its velocity is found to be 2 m//s . Find the amplitude.
View Solution