In the above problem, find total mechanical energy of the system .
The correct Answer is:A, B, C
To find the total mechanical energy of the system consisting of three particles each of mass m at the vertices of an equilateral triangle of side a, we will calculate both the potential energy and kinetic energy of the system.
Step 1: Understanding the System
We have three particles, each of mass m, positioned at the vertices of an equilateral triangle with side length a. The particles are moving with a speed v=√GMa.
Step 2: Calculate the Potential Energy
The gravitational potential energy U between two masses m1 and m2 separated by a distance r is given by:
U=−Gm1m2r
For our system, we need to calculate the potential energy for each pair of particles.
1. There are three pairs of particles: (1,2), (2,3), and (3,1).
2. The distance between each pair is a.
Thus, the potential energy for each pair is:
U12=U23=U31=−Gm2a
The total potential energy Utotal of the system is:
Utotal=U12+U23+U31=3(−Gm2a)=−3Gm2a
Step 3: Calculate the Kinetic Energy
The kinetic energy K of a single particle moving with speed v is given by:
K=12mv2
Substituting v=√Gma:
K=12m(Gma)=Gm22a
Since there are three particles, the total kinetic energy Ktotal is:
Ktotal=3(Gm22a)=3Gm22a
Step 4: Calculate Total Mechanical Energy
The total mechanical energy E of the system is the sum of the total kinetic energy and the total potential energy:
E=Ktotal+Utotal
Substituting the values we calculated:
E=3Gm22a−3Gm2a
Combining the terms:
E=3Gm22a−6Gm22a=−3Gm22a
Final Answer
The total mechanical energy of the system is:
E=−3Gm22a
We have three particles, each of mass m, positioned at the vertices of an equilateral triangle with side length a. The particles are moving with a speed v=√GMa.
The gravitational potential energy U between two masses m1 and m2 separated by a distance r is given by:
U=−Gm1m2r
For our system, we need to calculate the potential energy for each pair of particles.
2. The distance between each pair is a.
U12=U23=U31=−Gm2a
Utotal=U12+U23+U31=3(−Gm2a)=−3Gm2a
The kinetic energy K of a single particle moving with speed v is given by:
K=12mv2
Substituting v=√Gma:
K=12m(Gma)=Gm22a
Ktotal=3(Gm22a)=3Gm22a
The total mechanical energy E of the system is the sum of the total kinetic energy and the total potential energy:
E=Ktotal+Utotal
Substituting the values we calculated:
E=3Gm22a−3Gm2a
Combining the terms:
E=3Gm22a−6Gm22a=−3Gm22a
The total mechanical energy of the system is:
E=−3Gm22a
Topper's Solved these Questions
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Miscellaneous Examples
8 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Exercise 13.1
5 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises
45 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
Similar Questions
A particle of mass 40 g executes a simple harmonic motion of amplitude 2.0 cm. If the time period is 0.20 s, find the total mechanical energy of the system.
View SolutionA particle of mass 40 g executes a simple harmonic motion of amplitude 2.0 cm. If the time period is 0.20 s, find the total mechanical energy of the system.
View Solution
Knowledge Check
The usable form of mechanical energy is:
AElastic potential energyBKinetic energyCGravitational potential energyDNone of the given options.
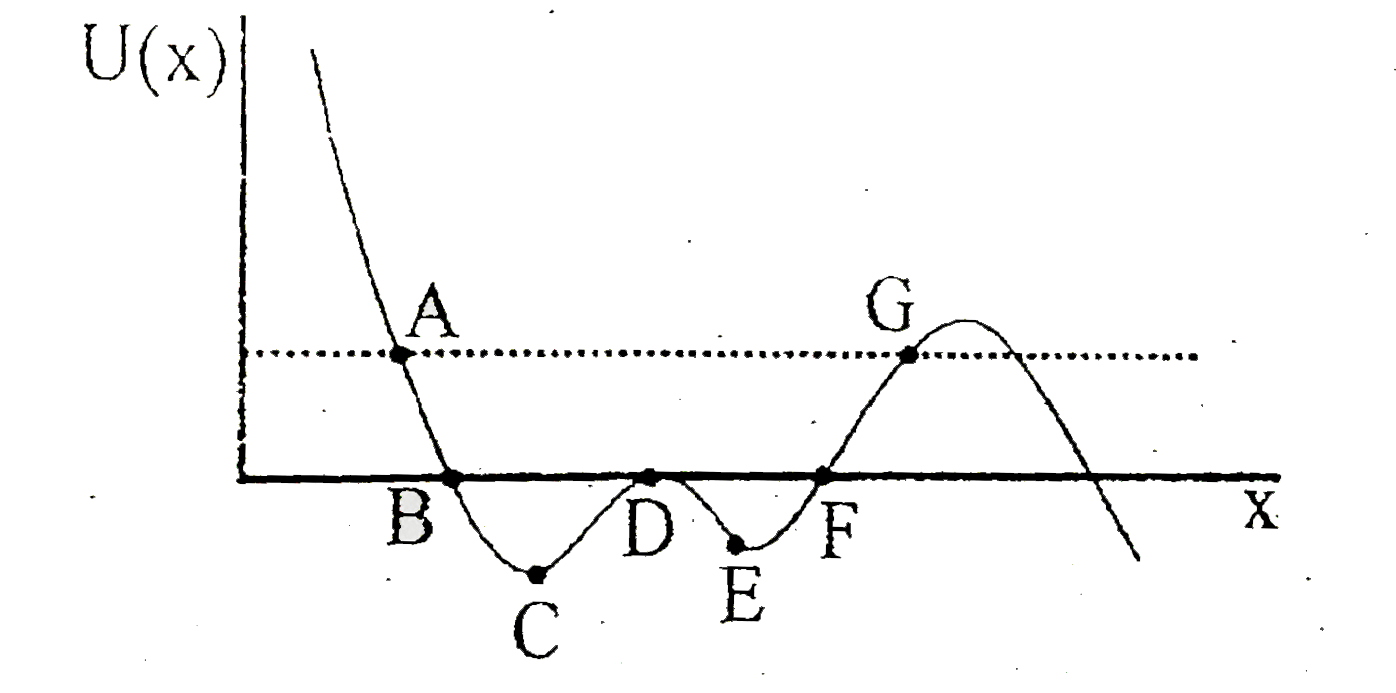
The figure below shows a graph of potential energy U(s) verses position x for a particle executing on edimensional motion along the x axis. The total mechanical energy of the system is indicated by the dashed line. At t=0 the particle is somewhere between points A and G. For later times choose the correct statement:-
View SolutionThe value of total mechanical energy of a particle is SHM is
View SolutionA thin hollow sphere of mass m=2kg, radius=(1/2)m is sliding on a horizontal surface with a constant frequency n = 60 rpm. Find the total mechanical energy of the hollow sphere.
View SolutionIn the above problem the angular velocity of the system after the particle sticks to it will be?
View SolutionIf particle is excuting simple harmonic motion with time period T, then the time period of its total mechanical energy is :-
View SolutionA ball of volume V and density ρ1 is moved downwards by a distance 'd' in liquid of density ρ2. Find total change in potential energy of the system.

View SolutionA lift is going up. The total mass of the lift and the passenger is 1500 kg. The variation in the speed of the lift is as shown in the figure. In the above problem, find the height to which the lift takes the passenger.
View Solution
