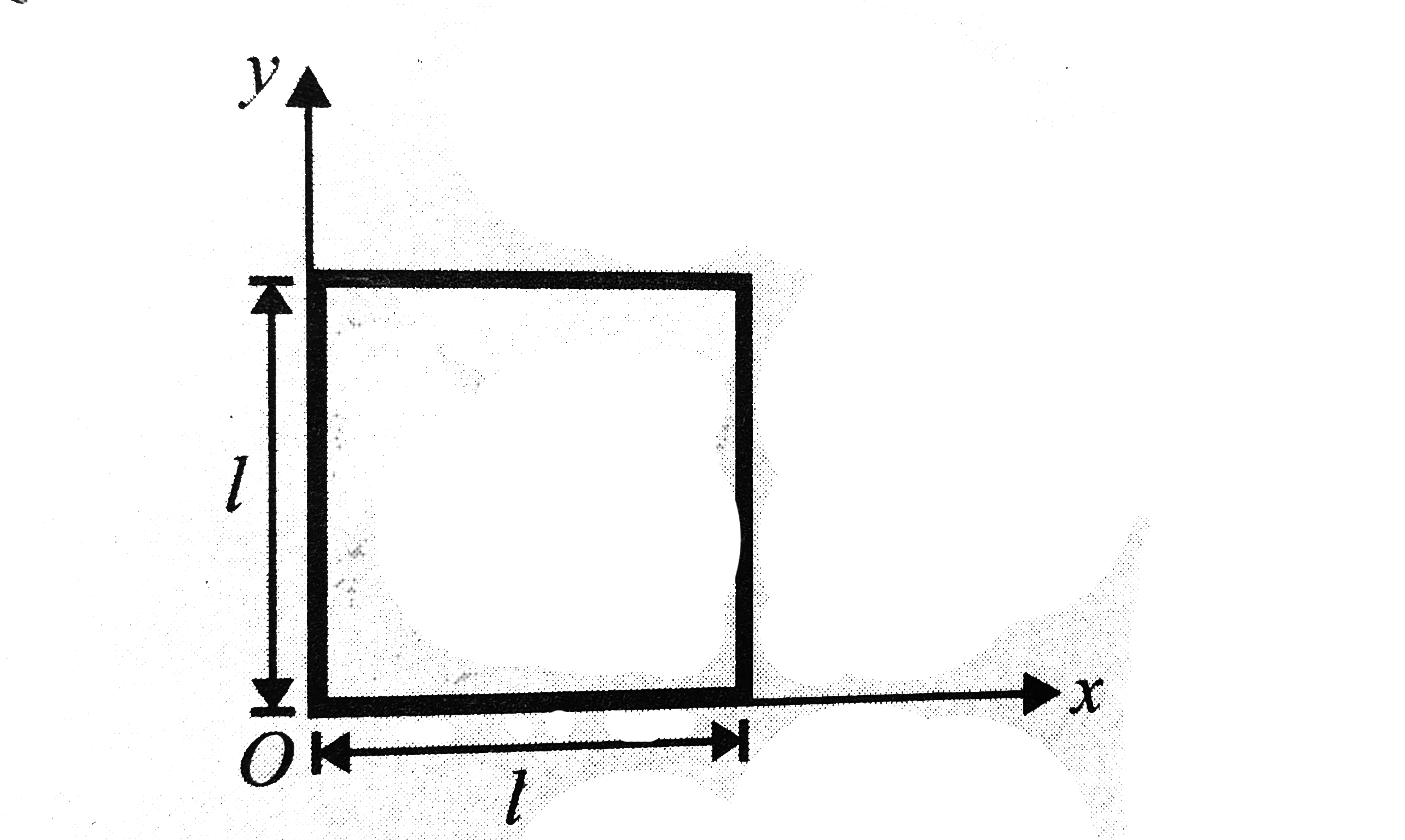
Four identical rods each of mass m and length l are joined to form a rigid square frame. The frame lies in the xy plane, with its centre at the origin and the sides parallel to the x and y axes. Its moment of inertia about
the x-axis is 23ml2
the z-axis is 43ml2
an axis parallel to the z-axis and passing through a corner is 103ml2
one side is 53ml2
To find the moment of inertia of the square frame about various axes, we will analyze each option step by step.
Step 1: Moment of Inertia about the X-axis (Ix)
1. Identify the rods: The square frame consists of four rods, each of mass m and length l. The frame is symmetric, so we can analyze two pairs of rods.
2. Calculate the moment of inertia for one rod about its center:
Icenter=ml212
3. Use the Parallel Axis Theorem for the vertical rods: For the vertical rods (let's say Rod 2 and Rod 4), the distance from the center of the frame to the x-axis is l2. Thus,
Ivertical=Icenter+m(l2)2=ml212+ml24=ml212+3ml212=4ml212=ml23
4. Calculate the total moment of inertia about the x-axis: Since there are two vertical rods,
Ix=2⋅Ivertical=2⋅ml23=2ml23
Step 2: Moment of Inertia about the Z-axis (Iz)
1. Use the Perpendicular Axis Theorem: For a planar object,
Iz=Ix+Iy
Since the frame is symmetric, Ix=Iy.
2. Calculate Iz:
Iz=Ix+Ix=2Ix=2⋅2ml23=4ml23
Step 3: Moment of Inertia about an axis parallel to the Z-axis and passing through a corner (I_corner)
1. Identify the distance to the center: The distance from the corner to the center of the square is l√22.
2. Use the Parallel Axis Theorem:
Icorner=Iz+m(l√22)2
Here, m is the total mass of the frame, which is 4m:
Icorner=4ml23+4m⋅l22=4ml23+2ml2=4ml23+6ml23=10ml23
Step 4: Moment of Inertia about one side (I_side)
1. Use the Parallel Axis Theorem: For the side (let's say Rod 1 and Rod 2),
Iside=Ix+md2
where d=l2 (the distance from the center to the side):
Iside=2ml23+4m⋅(l2)2=2ml23+4m⋅l24=2ml23+ml2=2ml23+3ml23=5ml23
Summary of Results:
- Moment of inertia about the x-axis: Ix=2ml23
- Moment of inertia about the z-axis: Iz=4ml23
- Moment of inertia about an axis through a corner: Icorner=10ml23
- Moment of inertia about one side: Iside=5ml23
Icenter=ml212
Ivertical=Icenter+m(l2)2=ml212+ml24=ml212+3ml212=4ml212=ml23
Ix=2⋅Ivertical=2⋅ml23=2ml23
Iz=Ix+Iy
Since the frame is symmetric, Ix=Iy.
Iz=Ix+Ix=2Ix=2⋅2ml23=4ml23
Icorner=Iz+m(l√22)2
Here, m is the total mass of the frame, which is 4m:
Icorner=4ml23+4m⋅l22=4ml23+2ml2=4ml23+6ml23=10ml23
Iside=Ix+md2
where d=l2 (the distance from the center to the side):
Iside=2ml23+4m⋅(l2)2=2ml23+4m⋅l24=2ml23+ml2=2ml23+3ml23=5ml23
- Moment of inertia about the x-axis: Ix=2ml23
- Moment of inertia about the z-axis: Iz=4ml23
- Moment of inertia about an axis through a corner: Icorner=10ml23
- Moment of inertia about one side: Iside=5ml23
Topper's Solved these Questions
- ROTATIONAL MECHANICSBook:DC PANDEY ENGLISHChapter:ROTATIONAL MECHANICSExercise:Level 2
Explore 1 Video
- ROTATIONAL MECHANICSBook:DC PANDEY ENGLISHChapter:ROTATIONAL MECHANICSExercise:Level 2 Subjective
Explore 36 Videos
- ROTATIONAL MECHANICSBook:DC PANDEY ENGLISHChapter:ROTATIONAL MECHANICSExercise:Level 2 Single Correct
Explore 26 Videos
- ROTATIONBook:DC PANDEY ENGLISHChapter:ROTATIONExercise:(C) Chapter Exercises
Explore 39 Videos
- ROTATIONAL MOTIONBook:DC PANDEY ENGLISHChapter:ROTATIONAL MOTIONExercise:Integer Type Questions
Explore 17 Videos
Similar Questions
Four identical rods, each of mass m and length l, make a square frame in the xy plane as shown in Fig.
a. Calculate its moment of inertia about the x-and y-axes.
b. Also, calculate its moment of inertia about the z-axis.
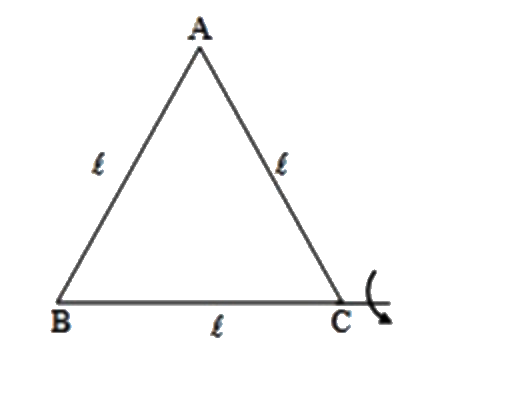
View SolutionThree identical rods each of mass M, length l are joined to form an equilateral ΔABC . Find the Moment of Inertia about BC as shown.
View SolutionFour identical thin rods each of mass M and length l, from a square frame. Moment of inertia of this frame about an axis through the centre of the square and perpendicular to its plane is
View SolutionThree identical rods, each of mass m and length l, form an equaliteral triangle. Moment of inertia about one of the sides is

View SolutionThree identical thin rods, each of mass m and length l, are joined to form an equilateral triangular frame. Find the moment of inertia of the frame about an axis parallel to tis one side and passing through the opposite vertex. Also find its radius of gyration about the given axis.
View SolutionFour thin rods each of mass m and length l are joined to make a square. Find moment of inertia of all the four rods about any side of the square.
View SolutionFour identical solid spheres each of mass M and radius R are fixed at four corners of a light square frame of side length 4R such that centres of spheres coincides with corners of square, moment of inertia of 4 spheres about an axis passing through any side of square is
View SolutionThree identical rods, each of length L, are joined to form a rigid equilateral triangle. Its radius of gyration about an axis passing thorugh a corner and perpendicular to plane of triangle is
View SolutionFour identical uniform thin rods, each of mass m and length l are joined to a common point O as shown in the figure. Moment of inertia of the system about an axis passing through O and lying in the plane of rods is
View SolutionTwo uniform, thin identical rods each of mass M and length l are joined together to form a cross. What will be the moment of inertia of the cross about an axis passing through the point at which the two rods are joined and perpendicular to the plane of the cross ?
View Solution