Four thin rods each of mass and length are joined to make a square. Find moment of inertia of all the four rods about any side of the square.
To find the moment of inertia of four thin rods joined to form a square about any side of the square, we will follow these steps:
Step 1: Understand the Configuration
We have a square made up of four thin rods, each of mass m and length l. The moment of inertia we need to calculate is about one of the sides of the square.
Step 2: Moment of Inertia of a Rod
The moment of inertia I of a thin rod of mass m and length l about an axis through one end and perpendicular to its length is given by the formula:
I=13ml2
Step 3: Identify the Contribution of Each Rod
When calculating the moment of inertia about one side of the square, we need to consider the contributions from all four rods:
- Rod along the chosen side: This rod's moment of inertia about the axis through its end is I1=13ml2.
- Rod opposite to the chosen side: This rod is parallel to the chosen side and is at a distance l from the axis. Its moment of inertia can be calculated using the parallel axis theorem:
I2=Icm+md2=13ml2+ml2=13ml2+ml2=43ml2
- Rods on the adjacent sides: Each of these rods is at a distance l2 from the axis. Their moment of inertia is also calculated using the parallel axis theorem:
I3=Icm+md2=13ml2+m(l2)2=13ml2+14ml2=712ml2
Since there are two such rods, their combined contribution is:
I4=2×712ml2=76ml2
Step 4: Total Moment of Inertia
Now, we can sum up the contributions from all four rods:
Itotal=I1+I2+I3+I4=13ml2+43ml2+76ml2
To add these fractions, we need a common denominator. The least common multiple of 3 and 6 is 6:
Itotal=26ml2+86ml2+76ml2=2+8+76ml2=176ml2
Final Answer
Thus, the moment of inertia of all four rods about any side of the square is:
I=176ml2
---
We have a square made up of four thin rods, each of mass m and length l. The moment of inertia we need to calculate is about one of the sides of the square.
The moment of inertia I of a thin rod of mass m and length l about an axis through one end and perpendicular to its length is given by the formula:
I=13ml2
When calculating the moment of inertia about one side of the square, we need to consider the contributions from all four rods:
- Rod along the chosen side: This rod's moment of inertia about the axis through its end is I1=13ml2.
- Rod opposite to the chosen side: This rod is parallel to the chosen side and is at a distance l from the axis. Its moment of inertia can be calculated using the parallel axis theorem:
I2=Icm+md2=13ml2+ml2=13ml2+ml2=43ml2
- Rods on the adjacent sides: Each of these rods is at a distance l2 from the axis. Their moment of inertia is also calculated using the parallel axis theorem:
I3=Icm+md2=13ml2+m(l2)2=13ml2+14ml2=712ml2
Since there are two such rods, their combined contribution is:
I4=2×712ml2=76ml2
Now, we can sum up the contributions from all four rods:
Itotal=I1+I2+I3+I4=13ml2+43ml2+76ml2
Itotal=26ml2+86ml2+76ml2=2+8+76ml2=176ml2
Thus, the moment of inertia of all four rods about any side of the square is:
I=176ml2
Topper's Solved these Questions
- ROTATIONAL MECHANICSBook:DC PANDEY ENGLISHChapter:ROTATIONAL MECHANICSExercise:Exercise 12.2
Explore 4 Videos
- ROTATIONAL MECHANICSBook:DC PANDEY ENGLISHChapter:ROTATIONAL MECHANICSExercise:Exercise 12.3
Explore 4 Videos
- ROTATIONAL MECHANICSBook:DC PANDEY ENGLISHChapter:ROTATIONAL MECHANICSExercise:Solved Example
Explore 1 Video
- ROTATIONBook:DC PANDEY ENGLISHChapter:ROTATIONExercise:(C) Chapter Exercises
Explore 39 Videos
- ROTATIONAL MOTIONBook:DC PANDEY ENGLISHChapter:ROTATIONAL MOTIONExercise:Integer Type Questions
Explore 17 Videos
Similar Questions
Three identical thin rods, each of mass m and length l, are joined to form an equilateral triangular frame. Find the moment of inertia of the frame about an axis parallel to tis one side and passing through the opposite vertex. Also find its radius of gyration about the given axis.
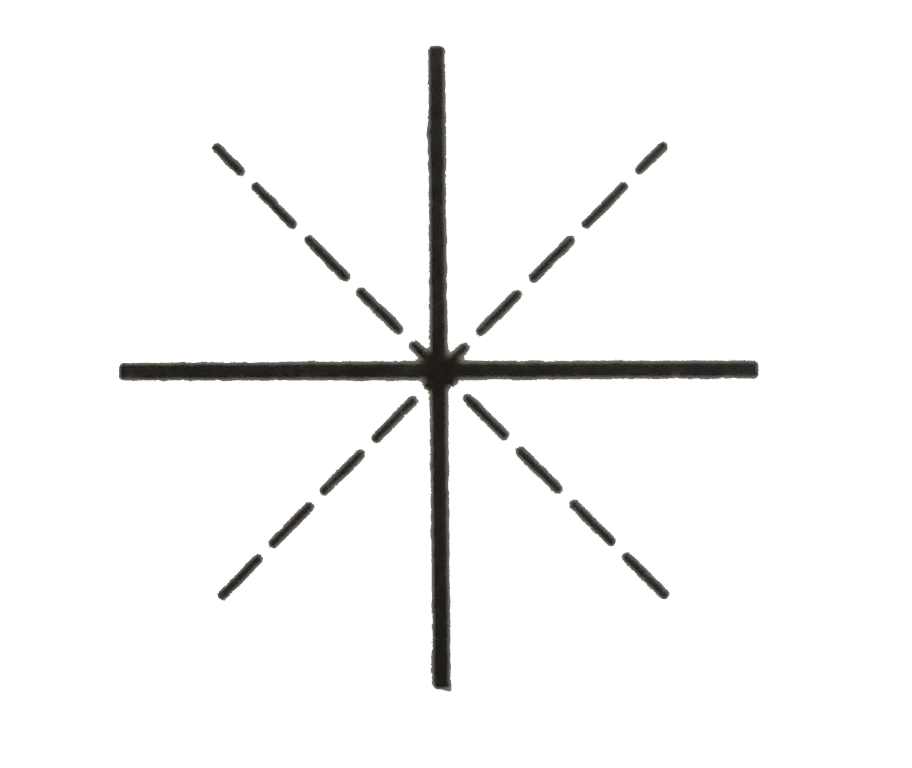
View SolutionTwo uniform identicla rods each of mass M and length l are joined to form a cross as shown in figure. Find the momet of inertia of the cross about a bisector as shown doted in the figure
View SolutionFour identical thin rods each of mass M and length l, from a square frame. Moment of inertia of this frame about an axis through the centre of the square and perpendicular to its plane is
View SolutionFour identical uniform thin rods, each of mass m and length l are joined to a common point O as shown in the figure. Moment of inertia of the system about an axis passing through O and lying in the plane of rods is
View SolutionTwo uniform, thin identical rods each of mass M and length l are joined together to form a cross. What will be the moment of inertia of the cross about an axis passing through the point at which the two rods are joined and perpendicular to the plane of the cross ?
View SolutionTwo rods each of mass m and length 1 are joined at the centre to form a cross. The moment of inertia of this cross about an axis passing through the common centre of the rods and perpendicular to the plane formed by them, is :
View SolutionTwo identical rods each of mass M and length L are kept according to figure. Find the moment of inertia of rods about an axis passing through O and perpendicular to the plane of rods.

View SolutionTwo uniform rods each of mass M and length L are joined as shown in the figure. The moment of inertia of the system about line AB is kML2.Find the value of k.
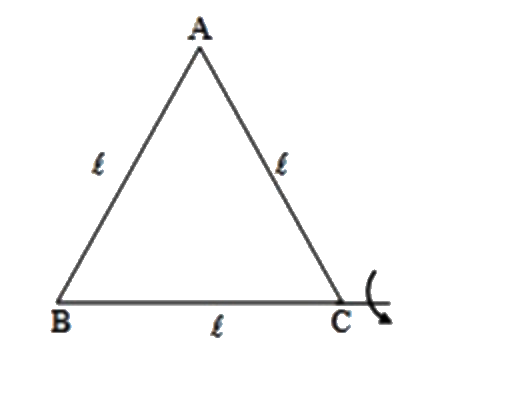
View SolutionThree identical rods each of mass M, length l are joined to form an equilateral ΔABC . Find the Moment of Inertia about BC as shown.
View Solution
Three rods each of mass m and length l are joined together to form an equilateral triangle as shown in figure. Find the moment of inertial of the system about an axis passing through its centre of mass and perpendicular to the plane of the particle.
View Solution