Question

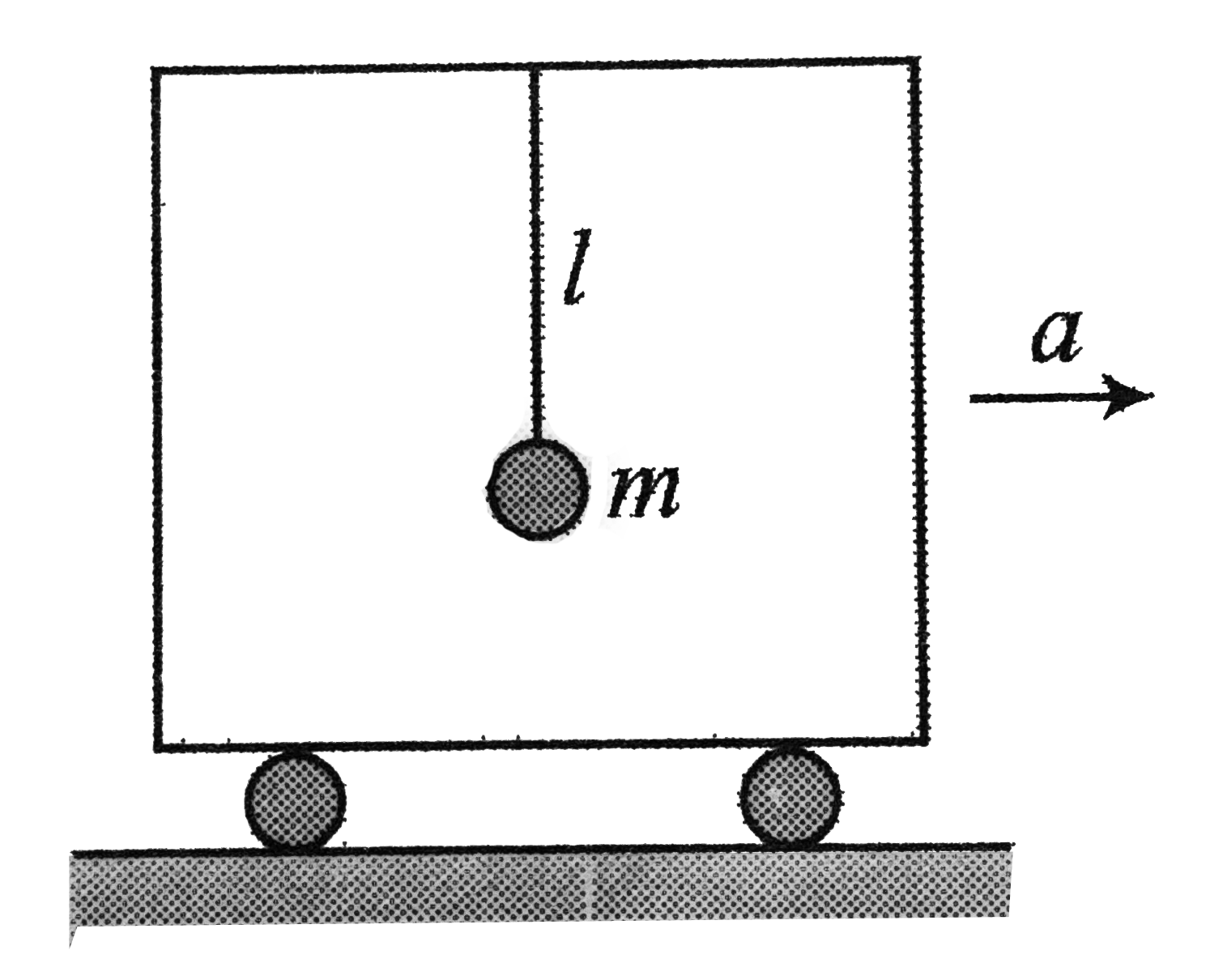
A wagon of mass ${\displaystyle M}$ can move without friction along horizontal rails. A simple pendulum consisting of a sphere of mass ${\displaystyle m}$ is suspended from the ceiling of the wagon by a string of length l. At the initial moment the wagon and the pendulum are at rest and the string is deflected through an angle ${\displaystyle \alpha }$ from the vertical. Find the velocity of the wagon when the pendulum passes through its mean position.

Answer

A::B

Explanation

To solve the problem, we will use the principles of conservation of momentum and the work-energy theorem. Let's break down the solution step by step.

Step 1: Understand the Initial and Final States
Initially, both the wagon and the pendulum are at rest. The pendulum is deflected at an angle \( \alpha \) from the vertical. When the pendulum passes through its mean position, it will have a certain velocity \( u \), and the wagon will have a velocity \( V \).

Step 2: Apply Conservation of Momentum
Since there are no external horizontal forces acting on the system, the horizontal momentum is conserved. Thus, we can write the conservation of momentum equation as:

\( 0 = m(-u) + M(V) \)

Where:
- \( m \) is the mass of the pendulum bob,
- \( u \) is the velocity of the pendulum bob when it reaches the mean position,
- \( M \) is the mass of the wagon,
- \( V \) is the velocity of the wagon.

Rearranging gives us:

\( m u = M V \quad \text{(1)} \)

Step 3: Calculate the Height Change
As the pendulum swings from the angle \( \alpha \) to the mean position, it descends a height \( h \). The height \( h \) can be calculated using:

\( h = l(1 - \cos \alpha) \)

Where \( l \) is the length of the string.

Step 4: Apply the Work-Energy Theorem
The work-energy theorem states that the work done on the system is equal to the change in kinetic energy. The initial kinetic energy is zero since both the wagon and the pendulum are at rest. Thus, we have:

\( \text{Final Kinetic Energy} = \text{Potential Energy lost} \)

The potential energy lost by the pendulum as it descends is:

\( mgh = mg(l(1 - \cos \alpha)) \)

The final kinetic energy of the system when the pendulum is at the mean position is:

\( \frac{1}{2} m u^2 + \frac{1}{2} M V^2 \quad \text{(2)} \)

Setting the two equal gives us:

\( \frac{1}{2} m u^2 + \frac{1}{2} M V^2 = mg l(1 - \cos \alpha \)

Step 5: Substitute \( u \) from Equation (1) into Equation (2)
From equation (1), we can express \( u \) in terms of \( V \):

\( u = \frac{M}{m} V \)

Substituting this into equation (2):

\( \frac{1}{2} m \left(\frac{M}{m} V\right)^2 + \frac{1}{2} M V^2 = mg l(1 - \cos \alpha \)

This simplifies to:

\( \frac{1}{2} \frac{M^2}{m} V^2 + \frac{1}{2} M V^2 = mg l(1 - \cos \alpha \)

Step 6: Factor Out \( V^2 \)
Combining the terms gives:

\( \frac{1}{2} V^2 \left(\frac{M^2}{m} + M\right) = mg l(1 - \cos \alpha \)

Step 7: Solve for \( V^2 \)
Rearranging gives:

\( V^2 = \frac{2mg l(1 - \cos \alpha)}{\frac{M^2}{m} + M} \)

Step 8: Take the Square Root to Find \( V \)
Finally, we take the square root to find the velocity of the wagon:

\( V = \sqrt{\frac{2mg l(1 - \cos \alpha)}{\frac{M^2}{m} + M}} \)

Summary
The velocity of the wagon when the pendulum passes through its mean position is given by:

\( V = \sqrt{\frac{2mg l(1 - \cos \alpha)}{\frac{M^2}{m} + M}} \)