A bullet of mass 0.25kg is fired with velocity 302m/s into a block of wood of mass m1=37.5kg. It gets embedded into it. The block m1 is resting on a long block m2 and the horizontal surface on which it is placed is smooth. The coefficient of friction between m1 and m2 is 0.5. Find the displacement of m1 on m2 and the common velocity of m1 and m2. Mass m2=1.25kg.
The correct Answer is:A, D
To solve the problem, we will follow these steps:
Step 1: Apply Conservation of Momentum
The conservation of momentum states that the total momentum before the collision is equal to the total momentum after the collision.
Let:
- Mass of the bullet, mb=0.25kg
- Velocity of the bullet, vb=302m/s
- Mass of the block, m1=37.5kg
- Mass of the second block, m2=1.25kg
Before the collision, the momentum of the system is:
Initial momentum=mb⋅vb+m1⋅0=0.25⋅302+0=75.5kg m/s
After the bullet embeds into the block, the total mass is mb+m1 and let V be the common velocity after the collision:
Final momentum=(mb+m1)⋅V
Setting initial momentum equal to final momentum:
75.5=(0.25+37.5)⋅V
75.5=37.75⋅V
V=75.537.75≈2.0m/s
Step 2: Calculate the Force of Friction
The force of friction Ff acting on block m1 is given by:
Ff=μ⋅N
where μ=0.5 (coefficient of friction) and N is the normal force. The normal force N is equal to the weight of the block m1 plus the bullet:
N=(m1+mb)⋅g=(37.5+0.25)⋅9.81≈37.75⋅9.81≈370.5N
Thus, the force of friction is:
Ff=0.5⋅370.5≈185.25N
Step 3: Calculate the Displacement
The kinetic energy KE of the block m1 after the collision is:
KE=12(m1+mb)V2=12(37.75)(2.0)2=12⋅37.75⋅4=75.5J
The work done by the friction force to stop the block is equal to the kinetic energy:
Ff⋅y=KE
185.25⋅y=75.5
y=75.5185.25≈0.407m
Final Results
- Common velocity V≈2.0m/s
- Displacement y≈0.407m
The conservation of momentum states that the total momentum before the collision is equal to the total momentum after the collision.
- Mass of the bullet, mb=0.25kg
- Velocity of the bullet, vb=302m/s
- Mass of the block, m1=37.5kg
- Mass of the second block, m2=1.25kg
Initial momentum=mb⋅vb+m1⋅0=0.25⋅302+0=75.5kg m/s
Final momentum=(mb+m1)⋅V
75.5=(0.25+37.5)⋅V
75.5=37.75⋅V
V=75.537.75≈2.0m/s
The force of friction Ff acting on block m1 is given by:
Ff=μ⋅N
where μ=0.5 (coefficient of friction) and N is the normal force. The normal force N is equal to the weight of the block m1 plus the bullet:
N=(m1+mb)⋅g=(37.5+0.25)⋅9.81≈37.75⋅9.81≈370.5N
Thus, the force of friction is:
Ff=0.5⋅370.5≈185.25N
The kinetic energy KE of the block m1 after the collision is:
KE=12(m1+mb)V2=12(37.75)(2.0)2=12⋅37.75⋅4=75.5J
Ff⋅y=KE
185.25⋅y=75.5
y=75.5185.25≈0.407m
- Common velocity V≈2.0m/s
- Displacement y≈0.407m
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Single Correct
23 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Single Correct Option
1 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 1 Subjective
32 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery
19 Videos
Similar Questions
A bullet of mass m moving with velocity v strikes a block of mass M at rest and gets embedded into it. The kinetic energy of the composite block will be
View SolutionA bullet of mass 'm' moving with velocity 'u' passes through a wooden block of mass M=nm as shown in figure. The block is resting on a smooth horizontal floor. After passing through the block, velocity relative to the block is
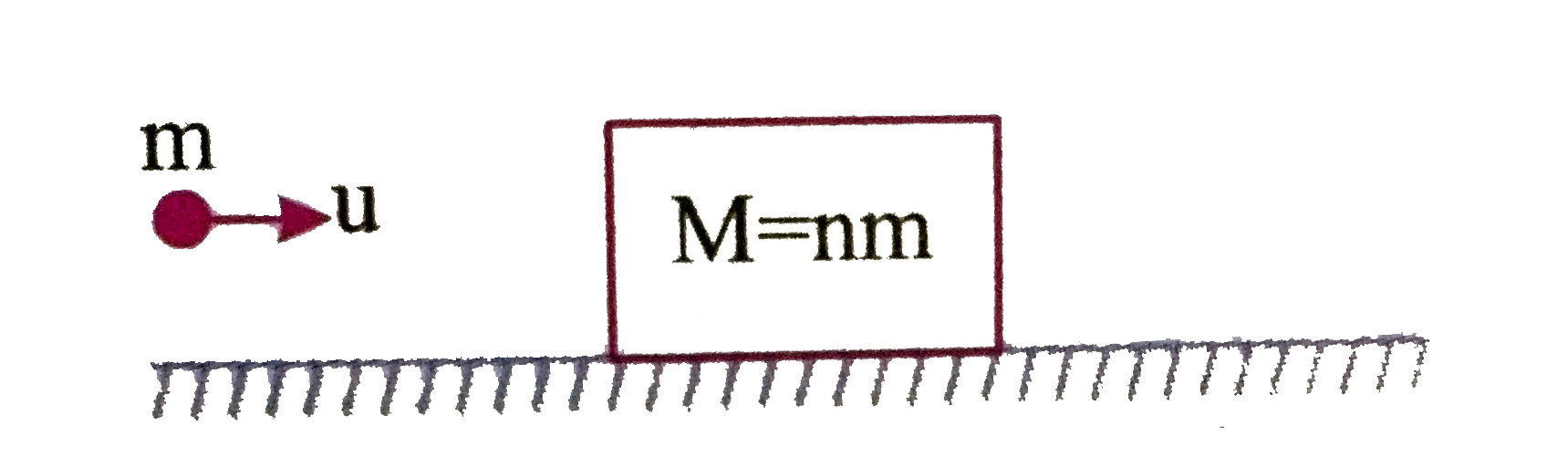 .
.
View Solution
Knowledge Check
A bullet of mass m moving horizontally with a velocity v strikes a block of wood of mass M and gets embedded in the block. The block is suspended from the ceiling by a massless string. The height to which block rises is
Av22g(mM+m)2Bv22g(M+mm)2Cv22g(mM)2Dv22g(Mm)2A block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
A10NB5NC2.5ND20N
A bullet of mass m is fired into a block of wood of mass M which hangs on the end of pendulum and gets embedded into it. When the bullet strikes with maximum rise R. Then, the velocity of the bullet is given by
View SolutionA block of mass m=5kg is resting on a rough horizontal surface for which the coefficient of friction is 0.2 . When a force F=40N is applied, the acceleration of the block will be (g=10m/s2) .

View SolutionA block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
View SolutionA bullet of mass 5gm is fired horizontally into block of mass 1kg at rest on a horizontal surface and gets embedded in it. The combination moves 5m before coming to rest. If μk=0.40, what is the speed with which the bullet strikes the block ?
View SolutionA bullet of mass m and velocity v is fired into a large block of mass M. The final velocity of the system is
View SolutionA block of mass m is placed on another block of mass M lying on a smooth horizontal surface as shown in the figure. The co-efficient of friction between the blocks is μ. Find the maximum horizontal force F (in Newton) that can be applied to the block M so that the blocks together with same acceleration? (M=3kg,m=2kg,μ=0.1,g=10m/s2)

View Solution
