A ball of mass 50 g moving at a speed of 2.0 m/s strikes a plane surface at an angle of incidence 450. The ball is reflected by the plane at an equal angle of reflection with the same speed. Calculate (a). the magnitude of the change in momentum of the ball (b). the change in the magnitude of the mometum of the ball.
The correct Answer is:A, B, D
To solve the problem step by step, we will calculate the change in momentum of the ball before and after it strikes the plane surface.
Given Data:
- Mass of the ball, m=50g=0.05kg
- Initial speed of the ball, v=2.0m/s
- Angle of incidence (and reflection), θ=45∘
Step 1: Calculate the initial momentum of the ball
The initial velocity can be broken down into its components:
- vix=v⋅cos(45∘)=2⋅1√2=√2m/s
- viy=−v⋅sin(45∘)=−2⋅1√2=−√2m/s
Thus, the initial momentum →pi can be calculated as:
→pi=m⋅→vi=0.05⋅(√2^i−√2^j)=0.05√2^i−0.05√2^j
Step 2: Calculate the final momentum of the ball
After striking the plane surface, the ball is reflected with the same speed but the direction of the y-component of the velocity changes. Therefore:
- vfx=−vix=−√2m/s
- vfy=viy=−√2m/s
Thus, the final momentum →pf can be calculated as:
→pf=m⋅→vf=0.05⋅(−√2^i−√2^j)=−0.05√2^i−0.05√2^j
Step 3: Calculate the change in momentum
The change in momentum Δ→p is given by:
Δ→p=→pf−→pi
Substituting the values:
Δ→p=(−0.05√2^i−0.05√2^j)−(0.05√2^i−0.05√2^j)
=−0.05√2^i−0.05√2^j−0.05√2^i+0.05√2^j
=−0.1√2^i
Step 4: Calculate the magnitude of the change in momentum
The magnitude of the change in momentum is:
|Δ→p|=|−0.1√2|=0.1√2≈0.1414kg m/s
Step 5: Change in the magnitude of the momentum
The magnitude of the initial momentum |→pi| is:
|→pi|=√(0.05√2)2+(−0.05√2)2=√0.0025+0.0025=√0.005=0.0707kg m/s
The magnitude of the final momentum |→pf| is the same:
|→pf|=√(−0.05√2)2+(−0.05√2)2=0.0707kg m/s
Thus, the change in the magnitude of the momentum is:
Δ|→p|=|→pf|−|→pi|=0.0707−0.0707=0
Final Answers:
(a) The magnitude of the change in momentum of the ball is approximately 0.1414kg m/s.
(b) The change in the magnitude of the momentum of the ball is 0.
- Mass of the ball, m=50g=0.05kg
- Initial speed of the ball, v=2.0m/s
- Angle of incidence (and reflection), θ=45∘
The initial velocity can be broken down into its components:
- vix=v⋅cos(45∘)=2⋅1√2=√2m/s
- viy=−v⋅sin(45∘)=−2⋅1√2=−√2m/s
→pi=m⋅→vi=0.05⋅(√2^i−√2^j)=0.05√2^i−0.05√2^j
After striking the plane surface, the ball is reflected with the same speed but the direction of the y-component of the velocity changes. Therefore:
- vfx=−vix=−√2m/s
- vfy=viy=−√2m/s
→pf=m⋅→vf=0.05⋅(−√2^i−√2^j)=−0.05√2^i−0.05√2^j
The change in momentum Δ→p is given by:
Δ→p=→pf−→pi
Substituting the values:
Δ→p=(−0.05√2^i−0.05√2^j)−(0.05√2^i−0.05√2^j)
=−0.05√2^i−0.05√2^j−0.05√2^i+0.05√2^j
=−0.1√2^i
The magnitude of the change in momentum is:
|Δ→p|=|−0.1√2|=0.1√2≈0.1414kg m/s
The magnitude of the initial momentum |→pi| is:
|→pi|=√(0.05√2)2+(−0.05√2)2=√0.0025+0.0025=√0.005=0.0707kg m/s
|→pf|=√(−0.05√2)2+(−0.05√2)2=0.0707kg m/s
Δ|→p|=|→pf|−|→pi|=0.0707−0.0707=0
(a) The magnitude of the change in momentum of the ball is approximately 0.1414kg m/s.
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 1 Subjective Questions
1 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Single Correct
23 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 1 Objective
42 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery
19 Videos
Similar Questions
A ball of mass 50 g moving at a speed of 2.0 m/s strikes a plane surface at an angle of incidence 450. The ball is reflected by the plane at equal angle of reflection with the same speed. Calculate a. the magnitude of the change in momentum of the ball b. the change in the magnitude of the momentum of the wall.
View SolutionA 0.5kg ball moving with a speed of 12m/s strikes a hard wall at an angle of 30∘ with the wall. It is reflected with the same speed and at the same angle . If the ball is in contact with the wall for 0.25s, the average force acting on the wall is

View SolutionA ball of mass 3kg moving with a speed of 100 m/s, strikes a wall at an angle 60∘ (as shown in figure). The ball rebounds at the same speed and remains in contact with the wall for 0.2 s, the force exerted by the ball on the wall is

View SolutionA ball moving with a momentum of 5kgms−1 strikes against a wall at an angle of 45∘ and is reflected at the same angle calculate the change in momentum
View SolutionA ball of mass 0⋅2 kg moving with a speed of 30 m/s strikes a bat returns in opposite direction with speed of 90m/s . Magnitude of change in momentu,m of ball will be
View SolutionAn object of m kg with speed of v ms−1 strikes a wall at an angle θ and rebounds at the same speed and same angle. Find the magnitude of change in the momentum of object.
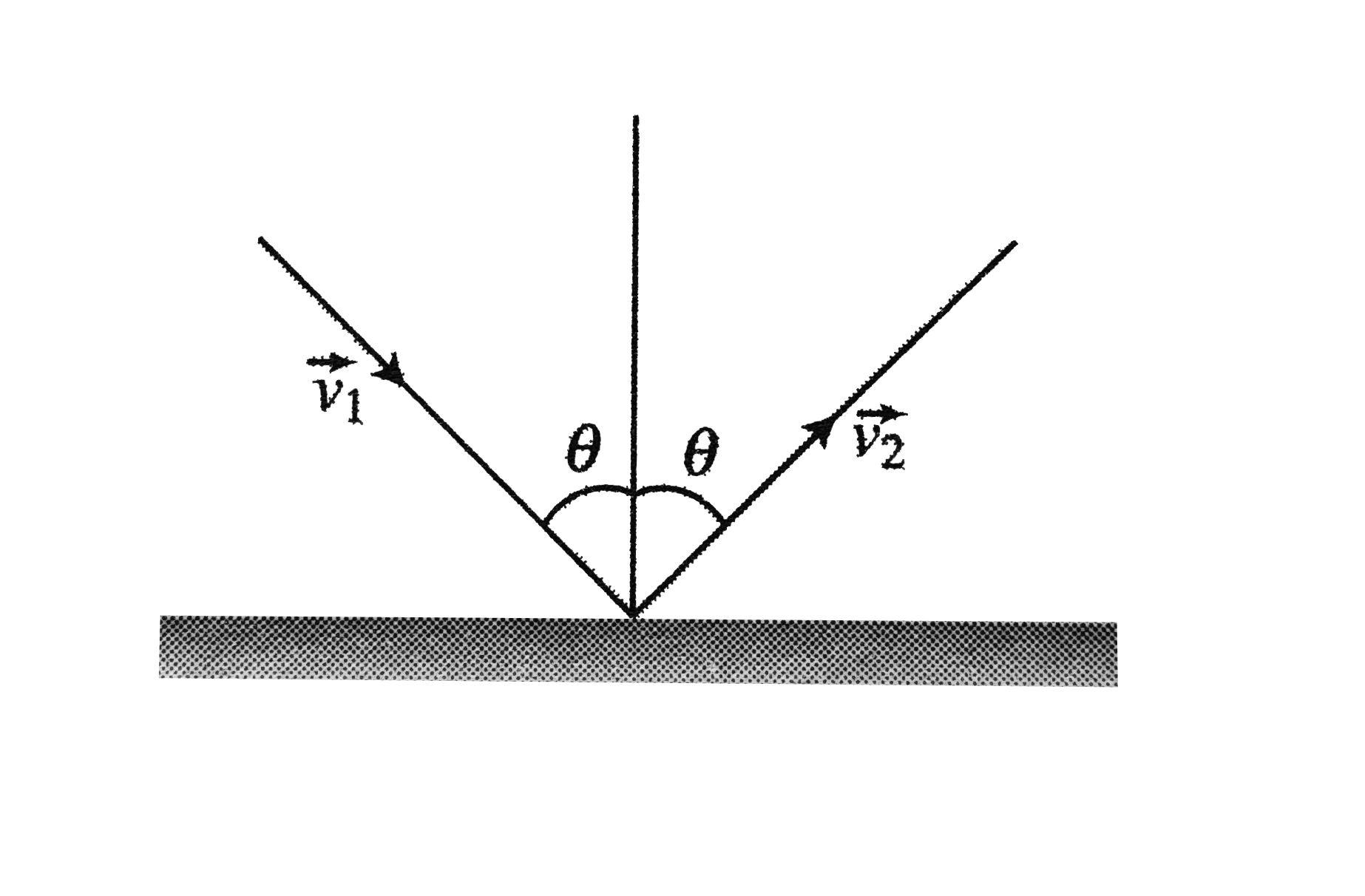
View SolutionAn object of m kg with speed of v ms−1 strikes a wall at an angle θ and rebounds at the same speed and same angle. Find the magnitude of change in the momentum of object.

View SolutionA body of mass m hits a wall with speed v at an angle of 30degree with normal and reflects at same angle from normal on other side of normal. The magnitude of change in linear momentum of the body is (Consider elastic collision)
View SolutionA ball of mass 600 gm strikes a wall with a velocity of 5ms−1 at an angle 30∘ with the wall and rebounds with the same speed at the same angle with the wall. The change in momentum of the ball is, (in kg ms−1)
View SolutionA particle of mass m strikes elastically on a wall with velocity v, at an angle of 60∘ from the wall then magnitude of change in momentum of ball along the wall is
View Solution
