Assume that a tunnel is dug along a chord of the earth, at a perpendicular distance (R/2) from the earth's centre, where 'R' is the radius of the Earth. The wall of the tunnel is frictionless. If a particle is released in this tunnel, it will execute a simple harmonic motion with a time period :
2πRg
g2πr
12π√gr
2π√Rg
The correct Answer is:D
To solve the problem, we need to determine the time period of a particle executing simple harmonic motion (SHM) when released in a tunnel dug along a chord of the Earth at a perpendicular distance of R2 from the Earth's center, where R is the radius of the Earth.
1. Understanding the Setup:
- The tunnel is dug at a distance of R2 from the center of the Earth.
- When a particle is released in this tunnel, it will experience gravitational force directed towards the center of the Earth.
2. Gravitational Force Inside the Earth:
- The gravitational force acting on the particle at a distance r from the center of the Earth is given by:
Fg=−GMemr2
- However, since the particle is at a distance of R2, we need to find the gravitational field at that point.
3. Gravitational Field Inside the Earth:
- The gravitational field E at a distance r from the center of the Earth is given by:
E=GMer2
- For r=R2:
E=GMe(R2)2=4GMeR2
4. Restoring Force:
- The restoring force F acting on the particle can be expressed as:
F=mE=m⋅4GMeR2
- This force acts towards the center of the Earth, which is characteristic of SHM.
5. Relating to SHM:
- In SHM, the restoring force is proportional to the displacement x from the equilibrium position:
F=−kx
- Here, we can identify k as:
k=4GMeR2
6. Finding the Time Period:
- The time period T of SHM is given by:
T=2π√mk
- Substituting for k:
T=2π
⎷m4GMeR2=2π√mR24GMe
- Since g=GMeR2, we can express Me in terms of g:
T=2π√Rg
7. Final Result:
- The time period of the particle executing SHM in the tunnel is:
T=2π√Rg
Conclusion:
Thus, the time period of the particle executing simple harmonic motion in the tunnel is T=2π√Rg.
- The tunnel is dug at a distance of R2 from the center of the Earth.
- When a particle is released in this tunnel, it will experience gravitational force directed towards the center of the Earth.
- The gravitational force acting on the particle at a distance r from the center of the Earth is given by:
Fg=−GMemr2
- However, since the particle is at a distance of R2, we need to find the gravitational field at that point.
- The gravitational field E at a distance r from the center of the Earth is given by:
E=GMer2
- For r=R2:
E=GMe(R2)2=4GMeR2
- The restoring force F acting on the particle can be expressed as:
F=mE=m⋅4GMeR2
- This force acts towards the center of the Earth, which is characteristic of SHM.
- In SHM, the restoring force is proportional to the displacement x from the equilibrium position:
F=−kx
- Here, we can identify k as:
k=4GMeR2
- The time period T of SHM is given by:
T=2π√mk
- Substituting for k:
T=2π
⎷m4GMeR2=2π√mR24GMe
- Since g=GMeR2, we can express Me in terms of g:
T=2π√Rg
- The time period of the particle executing SHM in the tunnel is:
T=2π√Rg
Thus, the time period of the particle executing simple harmonic motion in the tunnel is T=2π√Rg.
Topper's Solved these Questions
- JEE MAIN 2021Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2021Exercise:SECTION-B
Explore 40 Videos
- JEE MAIN 2021Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2021Exercise:PHYSICS (SECTION-A)
Explore 20 Videos
- JEE MAIN 2021Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2021Exercise:PHYSICS SECTION B
Explore 30 Videos
- JEE MAINBook:JEE MAINS PREVIOUS YEARChapter:JEE MAINExercise:All Questions
Explore 473 Videos
- JEE MAIN 2022Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2022Exercise:Question
Explore 492 Videos
Similar Questions
Assume that a tunnel is dug along a cord of earth at a perpendicular distance R2 from earth's centre where R is the radius of earth. The wall of tunnel is frictionless. Find the time period of particle excuting SHM in tunnel
View SolutionAssume that a tunnel ils dug along a chord of the earth, at a perpendicular distance R/2 from the earth's centre where R is the radius of the earth. The wall of the tunnel is frictionless. a. Find the gravitational force exerted by the earth on a particle of mass m placed in the tunnel at a distance x from the centre of the tunnel. b. Find the component of this fore along the tunnel and perpendicular to the tunnel. c. Find the normal force exerted by the wall on the particle. d. Find the resultant force on the particle. e.Show that the motion of the particle in the tunnel is simple harmonic and find the time period.
View Solution
Knowledge Check
A tunnel is dug along a chord of the earth at a perpendicular distance R/2 from the earth's centre. The wall of the tunnel may be assumed to be frictionless. A particle is released from one end of the tunnel. The pressing force by the particle on the wall, and the acceleration of the particle vary with x (distance of the particle from the centre) according to
A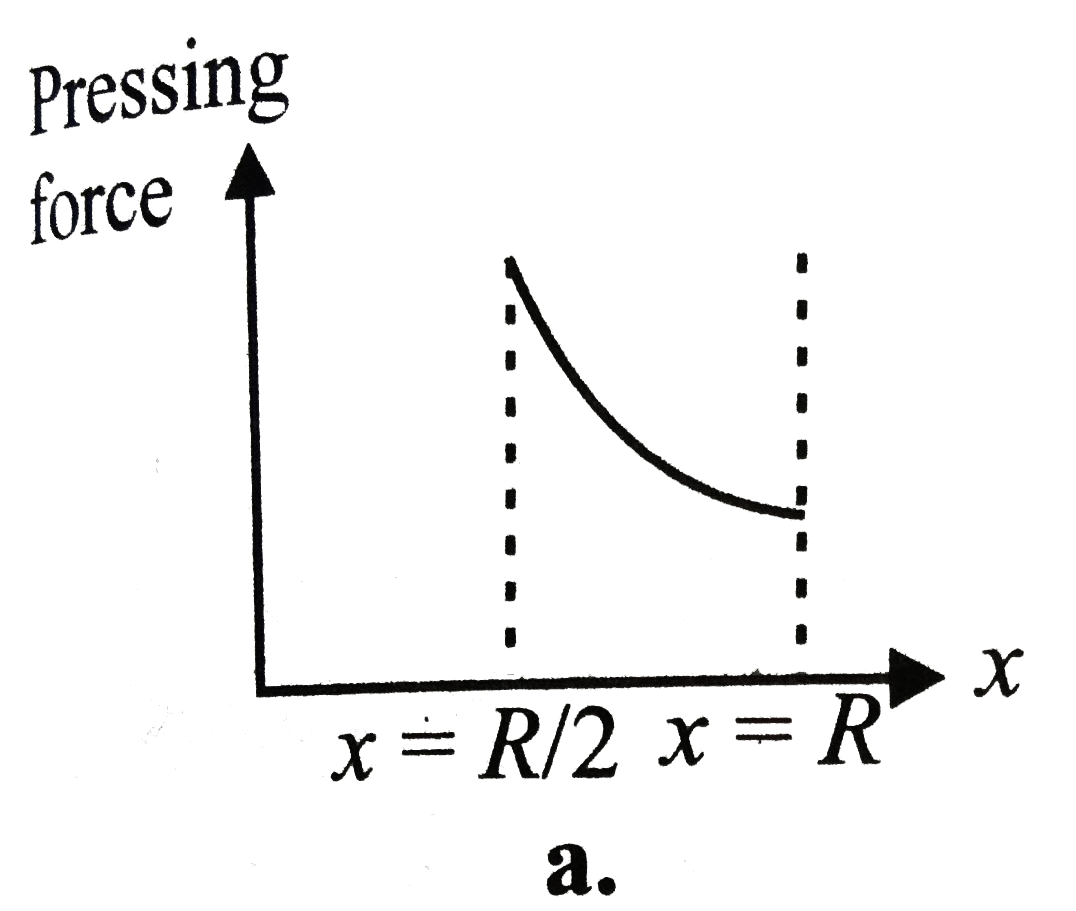 B
B C
C D
D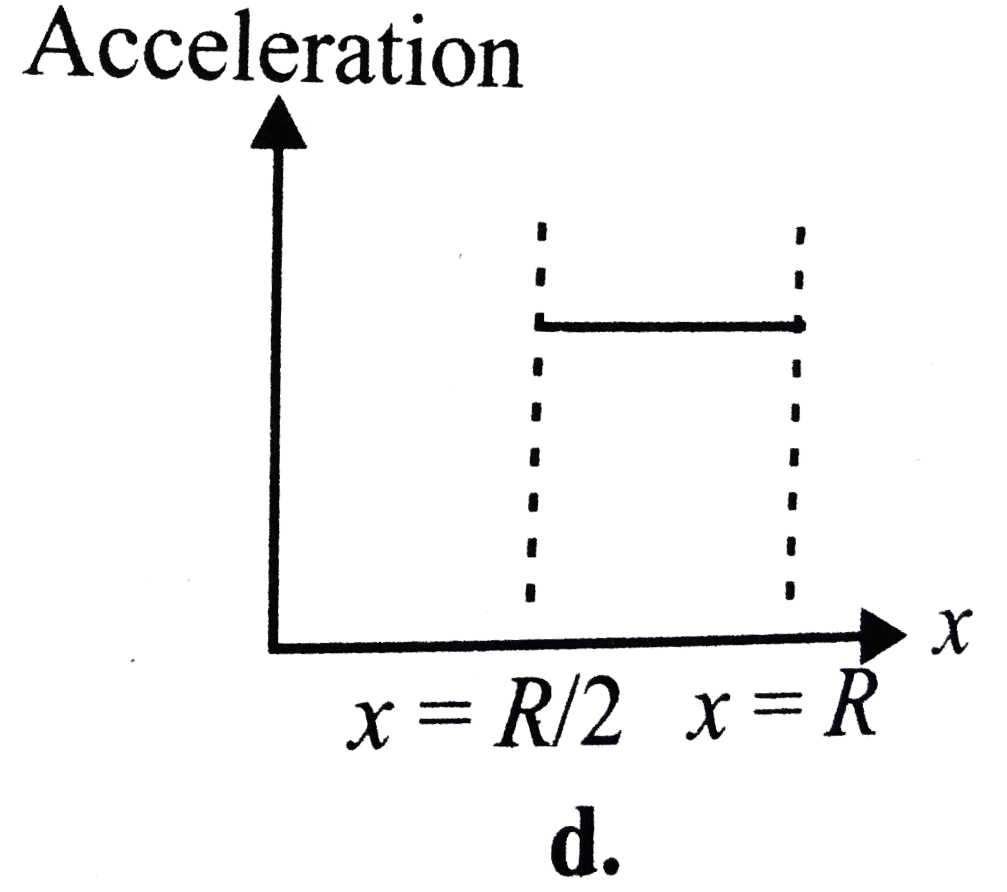
if a boby is released into a tunal dug across the diameter of earth, it executes simple harmonic motoin with time period
AT=2π√RegBT=2π√2RegCT=2π√Re2gDT=2 seconds
Assume that a tunnel is dug along a chord of the earth, at a perpendicular distance R/2 from the earth's centre where R is the radius of the earth. The wall of the tunnel is frictionless.
(a) Find the gravitational force exerted by the earth on a particle of mass m placed in the tunnel at a distance x from the centre of the tunnel.
(b) Find the component of this force along the tunnel and perpendicular to the tunnel.
(c) Find the normal force exerted by the wall on the particle.
(d) Find the resultant force on the particles.
(e) Show that the motion of the particle in the tunnel is simple harmonic and find the time period.
View SolutionA tunnel ois dug along a chord of the earth a perpendicular distance R2 from the earth's centre. The wall of the tunnel may be assumed to be frictionless. Find the force exerted by the eall on a particle of mass m when it is at a distance x from the centre of the tunnel.
View Solution(a) A tunnel is dug along a diameter of the earth. Find the force on the a particle of mass m placed in the tunnel at a distance x from the centre.
(b) A tunnel is dug along a chord of the earth at a perpendicular distance R/2 from the earth's centre. the wall of the tunnel may be assumed to be frictionless. Find the force exerted by the wall on a particle of mass m when it is at a distance x from the centre of the tunnel.
View SolutionAsume that as narrow tunnel is dug between two diametricaly opposite points of the earth. Treat the earth as a solid sphere of uniform density. Show that ilf a particle is relesed in this tunel, ilt wil execute a simple harmonic motion. Calculate the time period of this motion.
View SolutionA tunnel is dug through the centre of the earth. Shwo that a body of mass m when dropped form rest from one end of the tunnel will execute simple harmonic motion.
View SolutionImagine a smooth tunnel along a chord of nonrotating earth at a distance R2 from the centre. R is the radius of the earth. A projectile is fired along the tunnel from the centre of the tunnel at a speed V∘=√gR [g is acceleration due to gravity at the surface of the earth]. (a) Is the angular momentum [about the centre of the earth] of the projectile onserved as it moves along the tunnel? (b) Calculate the maximum distance of the projectile from the centre of the earth during its course of motion.
View Solution