A parallel beam of light of wavelength 560 nm falls on a thin film of oil (refractive index = 1.4). What should be the minimum thickness of the film so that it strongly transmit the light ?
The correct Answer is:A
To find the minimum thickness of the oil film that allows strong transmission of light, we can use the following steps:
Step 1: Understand the Condition for Strong Transmission
For a thin film to strongly transmit light, we need to consider the interference of light waves. The condition for constructive interference (strong transmission) in a thin film with a refractive index greater than that of the surrounding medium is given by:
2nt=(m+12)λ
where:
- n is the refractive index of the film,
- t is the thickness of the film,
- λ is the wavelength of light in vacuum,
- m is an integer (0, 1, 2,...).
Step 2: Rearranging the Formula
To find the minimum thickness t, we can rearrange the formula:
t=(m+12)λ2n
For the minimum thickness, we set m=0:
t=12λ2n=λ4n
Step 3: Substitute the Given Values
We are given:
- Wavelength λ=560nm=560×10−9m
- Refractive index n=1.4
Now substituting these values into the equation:
t=560×10−9m4×1.4
Step 4: Calculate the Thickness
Calculating the denominator:
4×1.4=5.6
Now substituting back:
t=560×10−95.6
Calculating the thickness:
t=100×10−9m=100nm
Final Answer
The minimum thickness of the oil film for strong transmission of light is:
t=100nm
---
For a thin film to strongly transmit light, we need to consider the interference of light waves. The condition for constructive interference (strong transmission) in a thin film with a refractive index greater than that of the surrounding medium is given by:
2nt=(m+12)λ
where:
- n is the refractive index of the film,
- t is the thickness of the film,
- λ is the wavelength of light in vacuum,
- m is an integer (0, 1, 2,...).
To find the minimum thickness t, we can rearrange the formula:
t=(m+12)λ2n
For the minimum thickness, we set m=0:
t=12λ2n=λ4n
We are given:
- Wavelength λ=560nm=560×10−9m
- Refractive index n=1.4
t=560×10−9m4×1.4
Calculating the denominator:
4×1.4=5.6
t=560×10−95.6
t=100×10−9m=100nm
The minimum thickness of the oil film for strong transmission of light is:
t=100nm
Topper's Solved these Questions
- LIGHT WAVESBOOK - HC VERMA ENGLISHCHAPTER - LIGHT WAVESEXERCISE - Question for short Answer
11 Videos
- LIGHT WAVESBOOK - HC VERMA ENGLISHCHAPTER - LIGHT WAVESEXERCISE - Objective -2
10 Videos
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Question For short Answer
18 Videos
- MAGNETIC FIELDBOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC FIELDEXERCISE - objective2
9 Videos
Similar Questions
A film with index of refraction 1.50 coats a glass lens with index of refraction 1.80. What is the minimum thickness of the thin film that will strongly reflect light with wavelength 600 nm?
View SolutionA light of wavelength 5890Å falls normally on a thin air film. The minimum thickness of the film such that the film appears dark in reflected light is
View SolutionA parallel beam of white light falls on a thin film whose refractive index is equal to 4/3. The angle of incidence i=53∘. What must be the minimum film thickness if the reflected light is to be coloured yellow most intensively? (tan53∘=4/3)
View SolutionA parallel beam of white light falls on a thin film whose refractive index is equal to 4/3. The angle of incidence i=53∘. What must be the minimum film thickness if the reflected light is to be coloured yellow most intensively? (tan53∘=4/3)
View SolutionFind the speed of light of wavelength λ=780nm (in air) in a medium of refractive index μ=1.55.
(b) What is the wavelength of this light in the given medium ?
View Solution_S01_025_Q01.png)
In Young's double slit experiment the slits, S1&S2 are illuminated by a parallel beam of light of wavelength 4000 Å from the medium of refractive index n1=1.2 A thin film of thickness 1.2 μm and refractive index n=1.5 is placed infrom of S1 perpedicular to path of light. the refractive index of medium between plane of slits & screen is n2=1.4 if the light coming from the film and S1&S2 have equal intensities I then intensity at geometrical centre of the screen O is
View SolutionA material having an index of refraction of 1.30 is used as an antireflective coating on a piece of glass (n = 1.50). What should be the minimum thickness of this film in order to minimize reflection of 500 nm light?
View SolutionA parallel beam of light falls on a solid tranparent sphere.
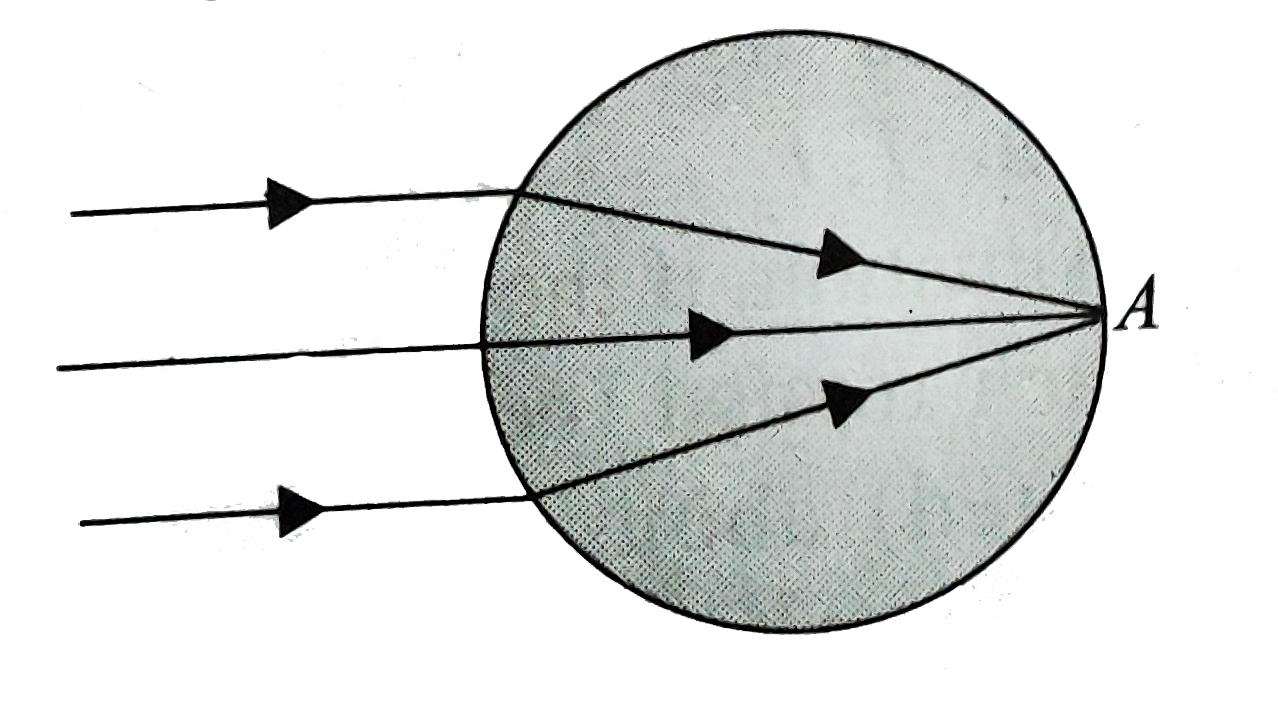
Q. For what value of refractive index μ, the thin beam can be focussed at centre of sphere.
View SolutionA double slit experiment is performed with light of wavelength 500nm. A thin film of thickness 2μm and refractive index 1.5 is introduced in the path of the upper beam. The location of the central maximum will
View SolutionOil floating on water looks cloured due to interference of light. What should be the approximate thickness of the film for such effects to be visible ?
View Solution
