A parallel beam of white light falls on a thin film whose refractive index is equal to 4/3. The angle of incidence i=53∘. What must be the minimum film thickness if the reflected light is to be coloured yellow most intensively? (tan53∘=4/3)
The correct Answer is:A, D
To find the minimum film thickness required for the reflected light to be colored yellow most intensively, we can follow these steps:
Step 1: Understand the Setup
We have a thin film with a refractive index μ=43 and a beam of white light incident at an angle i=53∘. We need to find the minimum thickness t of the film for constructive interference of yellow light.
Step 2: Determine the Angle of Refraction
Using Snell's law:
μ1sini=μ2sinr
where μ1=1 (air), μ2=43, and i=53∘.
Substituting the values:
1⋅sin(53∘)=43sin(r)
sin(r)=34sin(53∘)
Calculating sin(53∘):
sin(53∘)≈0.7986(using a calculator)
Thus,
sin(r)=34⋅0.7986≈0.59895
Now, we find r using the inverse sine function:
r≈sin−1(0.59895)≈37∘
Step 3: Calculate the Path Difference
The path difference Δx between the two rays (one reflected and one refracted) can be calculated as:
Δx=2tμcos(r)−2tsin(i)
Step 4: Express the Path Difference
Using the values:
- μ=43
- cos(37∘)≈0.7986
- sin(53∘)≈0.7986
We can express the path difference:
Δx=2t(43⋅0.7986)−2t⋅0.7986
Δx=2t(43⋅0.7986−0.7986)
Δx=2t⋅0.7986(43−1)
Δx=2t⋅0.7986⋅13=2t⋅0.79863
Step 5: Condition for Constructive Interference
For constructive interference, the path difference must equal nλ (where n is an integer and λ is the wavelength of yellow light). The minimum thickness corresponds to n=1:
Δx=λ
Step 6: Substitute Wavelength for Yellow Light
Using λ=0.6μm=0.6×10−6m:
2t⋅0.79863=0.6×10−6
Solving for t:
t=3⋅0.6×10−62⋅0.7986≈1.8×10−61.5972≈1.125×10−6m
Step 7: Final Calculation
Converting to micrometers:
t≈1.125μm
Conclusion
The minimum film thickness t required for the reflected light to be colored yellow most intensively is approximately 1.125μm.
We have a thin film with a refractive index μ=43 and a beam of white light incident at an angle i=53∘. We need to find the minimum thickness t of the film for constructive interference of yellow light.
Using Snell's law:
μ1sini=μ2sinr
where μ1=1 (air), μ2=43, and i=53∘.
1⋅sin(53∘)=43sin(r)
sin(r)=34sin(53∘)
Calculating sin(53∘):
sin(53∘)≈0.7986(using a calculator)
Thus,
sin(r)=34⋅0.7986≈0.59895
Now, we find r using the inverse sine function:
r≈sin−1(0.59895)≈37∘
The path difference Δx between the two rays (one reflected and one refracted) can be calculated as:
Δx=2tμcos(r)−2tsin(i)
Using the values:
- μ=43
- cos(37∘)≈0.7986
- sin(53∘)≈0.7986
Δx=2t(43⋅0.7986)−2t⋅0.7986
Δx=2t(43⋅0.7986−0.7986)
Δx=2t⋅0.7986(43−1)
Δx=2t⋅0.7986⋅13=2t⋅0.79863
For constructive interference, the path difference must equal nλ (where n is an integer and λ is the wavelength of yellow light). The minimum thickness corresponds to n=1:
Δx=λ
Using λ=0.6μm=0.6×10−6m:
2t⋅0.79863=0.6×10−6
Solving for t:
t=3⋅0.6×10−62⋅0.7986≈1.8×10−61.5972≈1.125×10−6m
Converting to micrometers:
t≈1.125μm
The minimum film thickness t required for the reflected light to be colored yellow most intensively is approximately 1.125μm.
Topper's Solved these Questions
- INTERFERENCE AND DIFFRACTION OF LIGHTBook:DC PANDEY ENGLISHChapter:INTERFERENCE AND DIFFRACTION OF LIGHTExercise:Level 2 Single Correct
Explore 11 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBook:DC PANDEY ENGLISHChapter:INTERFERENCE AND DIFFRACTION OF LIGHTExercise:LEVEL 2 (single correct option)
Explore 1 Video
- INTERFERENCE AND DIFFRACTION OF LIGHTBook:DC PANDEY ENGLISHChapter:INTERFERENCE AND DIFFRACTION OF LIGHTExercise:Level 1Subjective
Explore 22 Videos
- GRAVITATIONBook:DC PANDEY ENGLISHChapter:GRAVITATIONExercise:All Questions
Explore 135 Videos
- MAGNETIC FIELD AND FORCESBook:DC PANDEY ENGLISHChapter:MAGNETIC FIELD AND FORCESExercise:Medical entrance s gallery
Explore 59 Videos
Similar Questions
A parallel beam of white light falls on a thin film whose refractive index is equal to 4/3. The angle of incidence i=53∘. What must be the minimum film thickness if the reflected light is to be coloured yellow most intensively? (tan53∘=4/3)
View SolutionA parallel beam of light of wavelength 560 nm falls on a thin film of oil (refractive index = 1.4). What should be the minimum thickness of the film so that it strongly transmit the light ?
View SolutionA ray of light falls on a glass plate of refractive index μ=1.5.
What is the angle of incidence of the ray if the angle between the reflected and
refracted rays is 90∘?
View SolutionA ray of light falls on a glass plate of refractive index μ=1.5.
What is the angle of incidence of the ray if the angle between the reflected and
refracted rays is 90∘?
View SolutionA light of wavelength fall on a plane surface at an angle of incidence 53. The refractive index of surface material, if the reflected light is completely plane polarised is
View SolutionWhite light reflected from a soap film (Refractive index =1.5 ) has a maxima at 600 nm and a minima at 450 nm at with no minimum in between. Then, the thickness of the film is
View SolutionA parallel beam of light falls on a solid tranparent sphere.
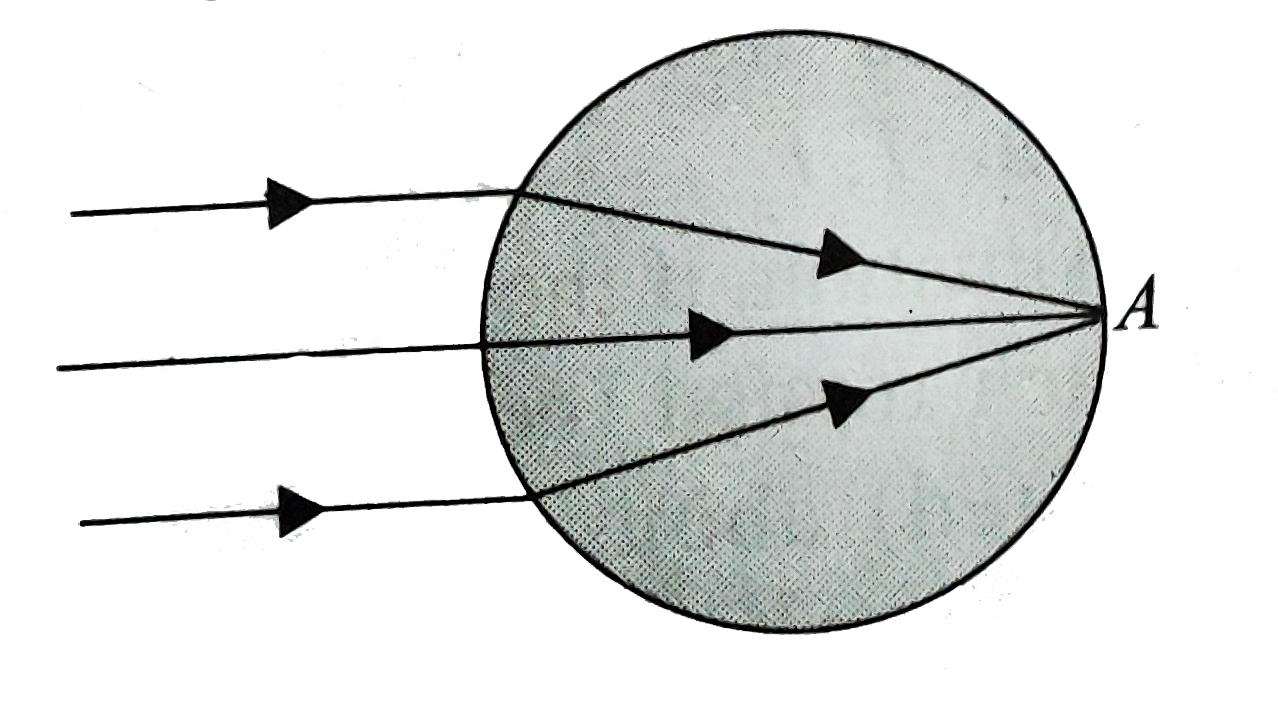
Q. For what value of refractive index μ, the thin beam can be focussed at centre of sphere.
View SolutionA circular beam of light of diameter d=2cm falls on a plane refractive of glass. The angle of incidence is 60∘ and refractive index of glass is μ=3/2. The diameter of the refracted beam is
View SolutionWhen white light is incident normally an oil film of thickness 10−4cm and refractive index. 1.4 then the wavelength which will not be seen in the reflected system of light is
View SolutionA parallel beam of white light is incident on a thin film of air of uniform thickness. Wavlength 7200Å and 5400Å are observed to be missing from the spectrum of reflected light viewed normally. The other wavelength in the visible region missing in the reflected spectrum is
View Solution