Consider an ideal gas with following distribution of spedds.
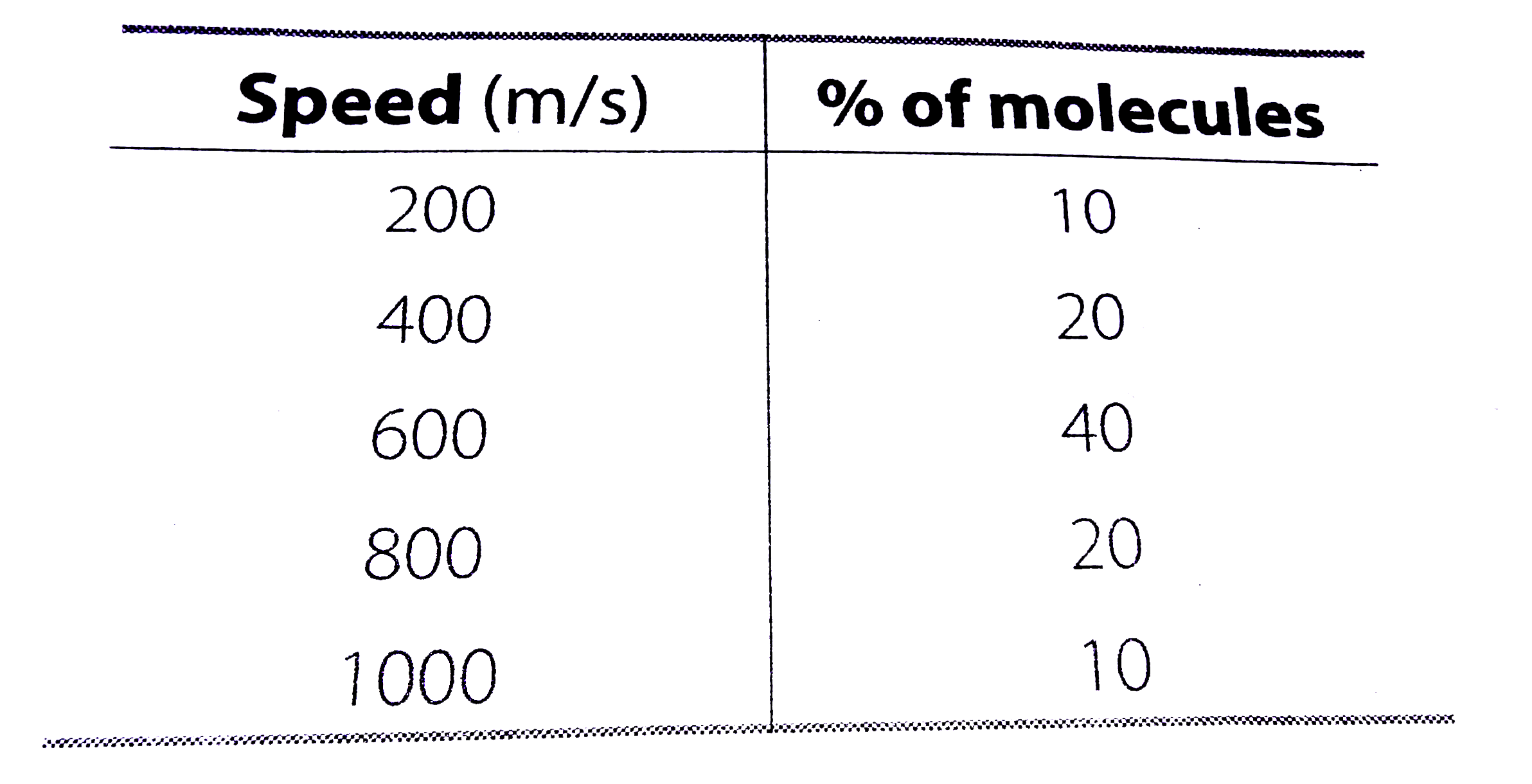
(a) Calculate Vrms and henceT. (m=3.0×10−26kg)
(b) If all the molecules with speed 1000m/s escape from the system, calculate new Vrms and hence T.
(a) We know that V2rms=ΣiniV2iΣni
This is the rms speed for all molecules collectively.
Now , Vrms=⎛⎜⎝Σiniv2iΣni⎞⎟⎠12
=√n1v21+n2v22+n3v23+.........+nnv2nn1+n2+n3+............+nn
=√n1v21+n2v22+n3v23+n4v24+n5v25n1+n2+n3+n4+n5
=
⎷10×(200)2+20×(400)2+40×(600)2+20×(800)2+10×(1000)2100
=√(1000×(4+32+144+128+100))
=√408×1000≈639m/s.
Now, according to kinetic theory of gasses
12mv2rms=32kgT [kB=Boltzmann constantm=mass of gaseous molecules]
T=13mv2rmsKB=13×30×10−26×4.08×1051.38×10−23
=2.96×102K=296K
(b) If all the molecules with speed 1000m/s escape ,then
V2rms=10×(200)2+20×(400)2+40×(600)2+20×(800)290
=10×1002×(1×4+2×16+4×36+2×64)90
=10000×3089=342×1000m2/s2
Vrms=584m/s
Again T=13mv2rmsK
=13×3×10−26×3.42×1051.38×10−23
=2.478×102
=247.8−248K
Note After escaping of molecules with speed of 1000 m/s the temperature in part (b) is 248 K whereas in part (a) before escaping of molecules the temperature was 297 K. thus evaprations facilitates cooling.
Topper's Solved these Questions
Similar Questions
For an ideal gas, the graph representing the variation of the speed of sound (v) in a gas, with the r.m.s. speed (vrms) of its gas molecules with be
View SolutionThe uncertainty in the momentum of a particle is 6.0×10−2kgms−1 .Calculate the uncertainty in the position
View SolutionConsider an 1100 particels gas system with speeds distribution as follows :
1000 particles each with speed 100m/s
2000 particles each wityh speed 200m/s
4000 particles each with speed 300m/s
3000 particles each with speed 400m/s and 1000 particles each with speed 500m/s
Find the average speed, and rms speed.
View SolutionThe root mean square speed of gas molecules at a temperature 27K and pressure 1.5 bar is 1×104cm/sec If both temperature and pressure are raised three times calculate the new rms speed of gas molecules .
View SolutionTwo moles of an ideal gas undergoes the following process. Given that (∂P∂T)Vis x×10+y, then calculate the value of (x + Y)
View SolutionThe velocities of sound in an ideal gas at temperature T1andT2 K are found to be V1andV2 respectively. If ther.m.s velocities of the molecules of the same gas at the same temperatures T1andT2 are v1andv2 respectively then
View SolutionThe velocities of sound in an ideal gas at temperature T1andT2 K are found to be V1andV2 respectively. If ther.m.s velocities of the molecules of the same gas at the same temperatures T1andT2 are v1andv2 respectively then
View SolutionRms speed of O2 molecule is 200m/s at T=300K and P=3atm. If diameter of molecule is 0.3nm then collision frequency is :
View SolutionAt 127∘C and 1.00×10−2 atm pressure, the density of a gas is 1.24×10−2kgm−3.
a. Find vrms for the gas molecules.
b. Find the molecular weight of the gas and identify it.
View SolutionFive molecules of a gas have speeds 1, 1, 3, 3, 2 km/s the value of the r.m.s spreed of the gas molecules is
View Solution
