In may experimental set-ups the source and screen are fixed at a distance say and the lens is movable. Show that there are two positions for the lens for which an image is formed on the screen. Find the distance between these points and the ratio of the image sizes for these two points.
To solve the problem, we will follow these steps:
Step 1: Understanding the Setup
In the given experimental setup, we have a fixed distance D between the source and the screen. The lens can be moved, and we need to find two positions of the lens where an image is formed on the screen.
Step 2: Defining Variables
Let:
- u = object distance (distance from the lens to the object)
- v = image distance (distance from the lens to the image on the screen)
- D = distance between the object and the screen (fixed)
From the geometry, we can express the relationship between u and v as:
u+v=D
This means v=D−u.
Step 3: Applying the Lens Formula
The lens formula is given by:
1f=1v−1u
Substituting v from the previous step:
1f=1D−u−1u
To simplify, we can find a common denominator:
1f=u−(D−u)u(D−u)=2u−Du(D−u)
This leads to:
u(D−u)f=2u−D
Step 4: Rearranging the Equation
Rearranging gives:
u(D−u)=f(2u−D)
Expanding and rearranging:
Du−u2=2fu−fD
u2−(D+2f)u+fD=0
Step 5: Solving the Quadratic Equation
This is a quadratic equation in u:
u2−(D+2f)u+fD=0
Using the quadratic formula u=−b±√b2−4ac2a:
u=D+2f±√(D+2f)2−4fD2
Step 6: Finding the Two Positions
The two positions u1 and u2 can be expressed as:
u1=D+2f+√(D+2f)2−4fD2
u2=D+2f−√(D+2f)2−4fD2
Step 7: Finding the Distance Between the Two Positions
The distance Δu between the two positions is:
Δu=u1−u2=√(D+2f)2−4fD
Step 8: Finding the Ratio of Image Sizes
The magnification m is given by:
m=vu
For the two positions:
m1=D−u1u1,m2=D−u2u2
Calculating the ratio of the magnifications:
Ratio=m1m2=(D−u1)u2(D−u2)u1
Summary
1. The two positions of the lens are given by the quadratic solutions for u.
2. The distance between these two positions is Δu=√(D+2f)2−4fD.
3. The ratio of the image sizes (magnifications) can be calculated using the derived expressions for m1 and m2.
In the given experimental setup, we have a fixed distance D between the source and the screen. The lens can be moved, and we need to find two positions of the lens where an image is formed on the screen.
Let:
- u = object distance (distance from the lens to the object)
- v = image distance (distance from the lens to the image on the screen)
- D = distance between the object and the screen (fixed)
u+v=D
This means v=D−u.
The lens formula is given by:
1f=1v−1u
Substituting v from the previous step:
1f=1D−u−1u
To simplify, we can find a common denominator:
1f=u−(D−u)u(D−u)=2u−Du(D−u)
This leads to:
u(D−u)f=2u−D
Rearranging gives:
u(D−u)=f(2u−D)
Expanding and rearranging:
Du−u2=2fu−fD
u2−(D+2f)u+fD=0
This is a quadratic equation in u:
u2−(D+2f)u+fD=0
Using the quadratic formula u=−b±√b2−4ac2a:
u=D+2f±√(D+2f)2−4fD2
The two positions u1 and u2 can be expressed as:
u1=D+2f+√(D+2f)2−4fD2
u2=D+2f−√(D+2f)2−4fD2
The distance Δu between the two positions is:
Δu=u1−u2=√(D+2f)2−4fD
The magnification m is given by:
m=vu
For the two positions:
m1=D−u1u1,m2=D−u2u2
Calculating the ratio of the magnifications:
Ratio=m1m2=(D−u1)u2(D−u2)u1
1. The two positions of the lens are given by the quadratic solutions for u.
2. The distance between these two positions is Δu=√(D+2f)2−4fD.
3. The ratio of the image sizes (magnifications) can be calculated using the derived expressions for m1 and m2.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTS Book:NCERT EXEMPLAR ENGLISHChapter:RAY OPTICS AND OPTICAL INSTRUMENTS Exercise:MULTIPLE CHOICE QUESTIONS (MORE THAN ONE OPTIONS )
Explore 6 Videos
- NUCLEIBook:NCERT EXEMPLAR ENGLISHChapter:NUCLEIExercise:Long answer type question
Explore 10 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIAL,DEVICES AND SIMPLE CIRCUITBook:NCERT EXEMPLAR ENGLISHChapter:SEMICONDUCTOR ELECTRONICS: MATERIAL,DEVICES AND SIMPLE CIRCUITExercise:Multiplel choice questions
Explore 26 Videos
Similar Questions
For the same statement as above, the ration of the two image sizes for these two positions of the lens is
View SolutionThe magnifications for two positions of image of the same lens are 3 and 2, of a fix point object on a fix screen when the distance between the two positions of lens is 30 cm .
The focal lengths of the lens is
View SolutionA lens of focal length 12cm forms an upright image three times the size of a real object. Find the distance in cm between the object and image.
View SolutionA convex lens is placed between an object and a screen which are at a fixed distance apart for one position of the lens. The magnification of the image obtained on the screen is m1. When the lens is moved by a distance d the magnification of the image obtained on the same screen m2, Find the focal length of the lens.
View SolutionAn object and a screen are fixed at a distance d apart. When a lens of focal length f is moved between the object and the screen, sharp images of the object are formed on the screen for two positions of the lens.
The magnifications produced at these two positions are M1andM2-
View SolutionAn object is placed 12cm in front of a lens to form an image on a screen. The size of the image is twice that of the object. What is the distance between the object and the image?
View SolutionThe filament of lamp is 80 cm from a screen and a converging lens forms an image of it on a screen, magnified three times. Find the distance of the lens from the filament and the focal length of the lens.
View SolutionA point object is placed as shown. The two pieces are of the same lens of focal length 10 cm. Find the distance (in cm) between the two images formed by two pieces of the lens.
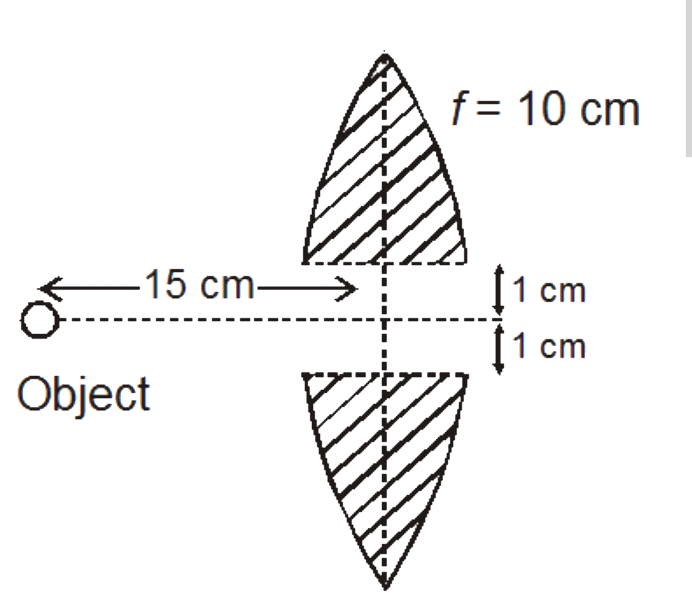
View SolutionIn a slide show programme, the image on the screen has an area 900times that of the slide. If the distance between the slide and the screen is x times the distance between the slide and the projector lens, then
View SolutionThe distance between object and the screen is D. Real images of an object are formed on the screen for two positions of a lens separated by a distance d. The ratio between the sizes of two images will be:
View Solution