For the same statement as above, the ration of the two image sizes for these two positions of the lens is
[D−dD+d]2
[D+dD−d]2
[D−2dD+2d]2
[D+2dD−2d]2
The correct Answer is:B
To solve the problem of finding the ratio of the sizes of two images formed by a convex lens at two different positions, we can follow these steps:
Step 1: Define the Variables
Let:
- D = initial distance from the object to the lens
- d = distance by which the lens is shifted
- u1 = object distance for the first position of the lens
- u2 = object distance for the second position of the lens
- v1 = image distance for the first position of the lens
- v2 = image distance for the second position of the lens
Step 2: Calculate Object Distances
For the first position of the lens:
u1=D−d2
For the second position of the lens:
u2=D+d2
Step 3: Calculate Image Distances
Using the lens formula, we can derive the image distances:
For the first position:
v1=D−u1=D−D−d2=D+d2
For the second position:
v2=D−u2=D−D+d2=D−d2
Step 4: Calculate Magnifications
The magnification m is given by the formula:
m=vu
For the first position:
m1=v1u1=D+d2D−d2=D+dD−d
For the second position:
m2=v2u2=D−d2D+d2=D−dD+d
Step 5: Calculate the Ratio of the Image Sizes
To find the ratio of the heights of the images formed at the two positions, we can use the magnification values:
Ratio=m1m2=D+dD−dD−dD+d=(D+d)2(D−d)2
Final Answer
The ratio of the two image sizes for these two positions of the lens is:
(D+d)2(D−d)2
Let:
- D = initial distance from the object to the lens
- d = distance by which the lens is shifted
- u1 = object distance for the first position of the lens
- u2 = object distance for the second position of the lens
- v1 = image distance for the first position of the lens
- v2 = image distance for the second position of the lens
For the first position of the lens:
u1=D−d2
u2=D+d2
Using the lens formula, we can derive the image distances:
v1=D−u1=D−D−d2=D+d2
v2=D−u2=D−D+d2=D−d2
The magnification m is given by the formula:
m=vu
m1=v1u1=D+d2D−d2=D+dD−d
m2=v2u2=D−d2D+d2=D−dD+d
To find the ratio of the heights of the images formed at the two positions, we can use the magnification values:
Ratio=m1m2=D+dD−dD−dD+d=(D+d)2(D−d)2
The ratio of the two image sizes for these two positions of the lens is:
(D+d)2(D−d)2
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig
2 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Linked Comprehension
51 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Subjective
26 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
In may experimental set-ups the source and screen are fixed at a distance say D and the lens is movable. Show that there are two positions for the lens for which an image is formed on the screen. Find the distance between these points and the ratio of the image sizes for these two points.
View SolutionWhen the distance between the object and the screen is more than 4f. We can obtain image of the object on the screen for the two positions of the lens. If is called displacement method. In one case, the image is magnified and in the other case, it is diminished. If I1 and I2 be the sized of the two images, then the size of the object is
View SolutionIf I1 and I2 be the size of the images respectively for the two positions of lens in the displacement method, then the size of the object is given by
View SolutionAn object and a screen are fixed at a distance d apart. When a lens of focal length f is moved between the object and the screen, sharp images of the object are formed on the screen for two positions of the lens.
The magnifications produced at these two positions are M1andM2-
View Solution(a) A screen is kept at a distance of 1m from the object. A converging lens between the object and the screen, when placed at any of the two positions which are 60cm apart, forms a sharp image of the object on the screen. Find the focal length of the lens.
(b) In the two positions of the lens, lateral size of the image is 4cm and 9cm. Find the size of the object.
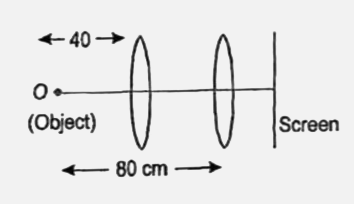
View SolutionIn displacement method, there are two position of a lens for which we get clear image. First position of the lens is at 40 cm from object and second is at 80 cm, the focal length of lens is
View SolutionA linear object is placed on the axis of a lens. An image is formed by refraction in the lens. For all positions of the object on the axis of the lens, the positions of the image are always between the lens and the object.
(i) Name the lens.
(ii) Draw a ray diagram to show the formation of the image of an object placed in front of the lens at any position of your choice except infinity.
View SolutionThe distance between an object and a screen is 100 cm. A lens can produce real image of the object on the screen for two different position between the screen and the object. The distance between these two positions is 40 cm. If the power of the lens is close to (N100)D where N is integer, the value of N is ___________.
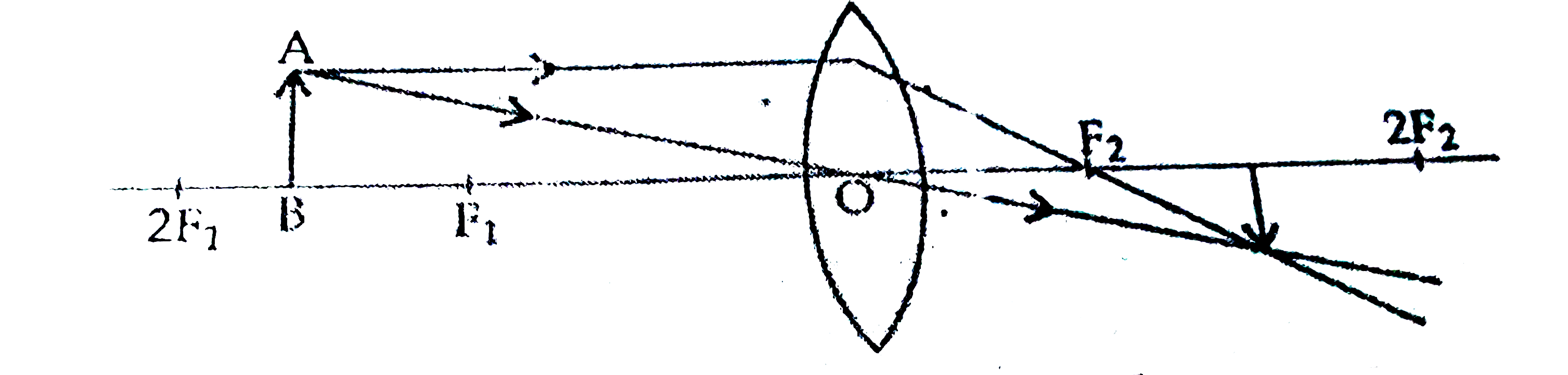
View SolutionStudy the following ray diagram and list any two mistakes committed by the student while tracing it. Rectify these mistakes by drawing the correct ray diagram to show the real position and size of the image corresponding to the position of the object AB.
View SolutionWhen an object is placed on the principal axis of a convex lens at two different positins, it produces the images with magnifications +2 and −4 respectively. How many times more away from the lens the image will be formed in the second position as compared to the first postion?
View Solution
