Three immiscible liquids of densities d1>d2>d3 and refractive indices μ1>μ2>μ3 are put in a beaker. The height of each liquid column is h3. A dot is made at the bottom of the beaker. For near normal vision, find the apparent depth of the dot.
To find the apparent depth of the dot at the bottom of the beaker containing three immiscible liquids with different densities and refractive indices, we can follow these steps:
1. Understanding the Setup:
- We have three immiscible liquids with densities d1>d2>d3 and refractive indices μ1>μ2>μ3.
- Each liquid has a height of h3.
2. Using the Formula for Apparent Depth:
- The relationship between real depth and apparent depth in a medium is given by:
μ=Real DepthApparent Depth
- Rearranging this gives:
Apparent Depth=Real Depthμ
3. Calculating Apparent Depth for Each Liquid:
- For the first liquid (with refractive index μ1):
x1=h3μ1=h3μ1
- For the second liquid (with refractive index μ2):
x2=h3μ2=h3μ2
- For the third liquid (with refractive index μ3):
x3=h3μ3=h3μ3
4. Finding the Total Apparent Depth:
- The total apparent depth x is the sum of the apparent depths from all three liquids:
x=x1+x2+x3
- Substituting the values we found:
x=h3μ1+h3μ2+h3μ3
5. Simplifying the Expression:
- Factor out h3:
x=h3(1μ1+1μ2+1μ3)
Final Answer:
The apparent depth of the dot at the bottom of the beaker is:
x=h3(1μ1+1μ2+1μ3)
- We have three immiscible liquids with densities d1>d2>d3 and refractive indices μ1>μ2>μ3.
- Each liquid has a height of h3.
- The relationship between real depth and apparent depth in a medium is given by:
μ=Real DepthApparent Depth
- Rearranging this gives:
Apparent Depth=Real Depthμ
- For the first liquid (with refractive index μ1):
x1=h3μ1=h3μ1
- For the second liquid (with refractive index μ2):
x2=h3μ2=h3μ2
- For the third liquid (with refractive index μ3):
x3=h3μ3=h3μ3
- The total apparent depth x is the sum of the apparent depths from all three liquids:
x=x1+x2+x3
- Substituting the values we found:
x=h3μ1+h3μ2+h3μ3
- Factor out h3:
x=h3(1μ1+1μ2+1μ3)
The apparent depth of the dot at the bottom of the beaker is:
x=h3(1μ1+1μ2+1μ3)
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTS BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTS EXERCISE - MULTIPLE CHOICE QUESTIONS (MORE THAN ONE OPTIONS )
6 Videos
- NUCLEIBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - NUCLEIEXERCISE - Long answer type question
10 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIAL,DEVICES AND SIMPLE CIRCUITBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIAL,DEVICES AND SIMPLE CIRCUITEXERCISE - Multiplel choice questions
26 Videos
Similar Questions
There are three optical media 1,2 and 3 with the refractive indices μ1>μ2>μ3.
(TIR → total internal reflection)
View SolutionTwo immiscible liquid of densities rho and 3ρ are put in a container. The height of each liquid is h. A solid cylinder of length L and density d is put in this container. The cylinder floats completely inside liquids with its axis vertical and length L3 in the denser liquid. The density d is equal to
View SolutionTwo non-mixing liquids of densities ρ and nρ (n>1) are put in a container. The height of each liquid is h. A solid cylinder of length L and density d is put in this container. The cylinder floats with its axis vertical and length pL(p<1) in the denser liquid. The density d is equal to :
View SolutionThree immiscibles transparent liquids with erefractive indices 3/2,4/3 and 6/5 are arranged one on top of another. The depth of the liquid are 3 cm, 4 cm and 6 cm respectively. The apparent depth of the vessel is
View SolutionA solid sphere of radius r is floating at the interface of two immiscible liquids of densities ρ1 and ρ2(ρ2>ρ1), half of its volume lying in each. The height of the upper liquid column from the interface of the two liquids is h. The force exerted on the sphere by the upper liquid is (atmospheric pressure =p0 and acceleration due to gravity is g):
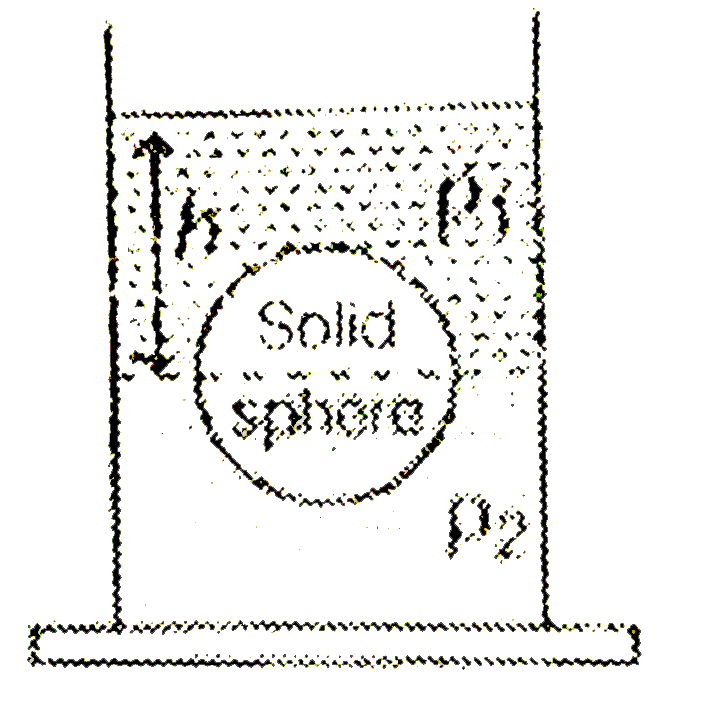
View SolutionA beaker is filled with two immiscible transparent liquids of refractive indices μ1 and μ2 having thickness of layers d1 and d2 respectively. The apparent depth of the bottom of the beaker is
View SolutionIn the figure shown, μ1>μ2>μ3. What are the limits of angle i so that it is neither get total internal reflection at AB nor at CD ?

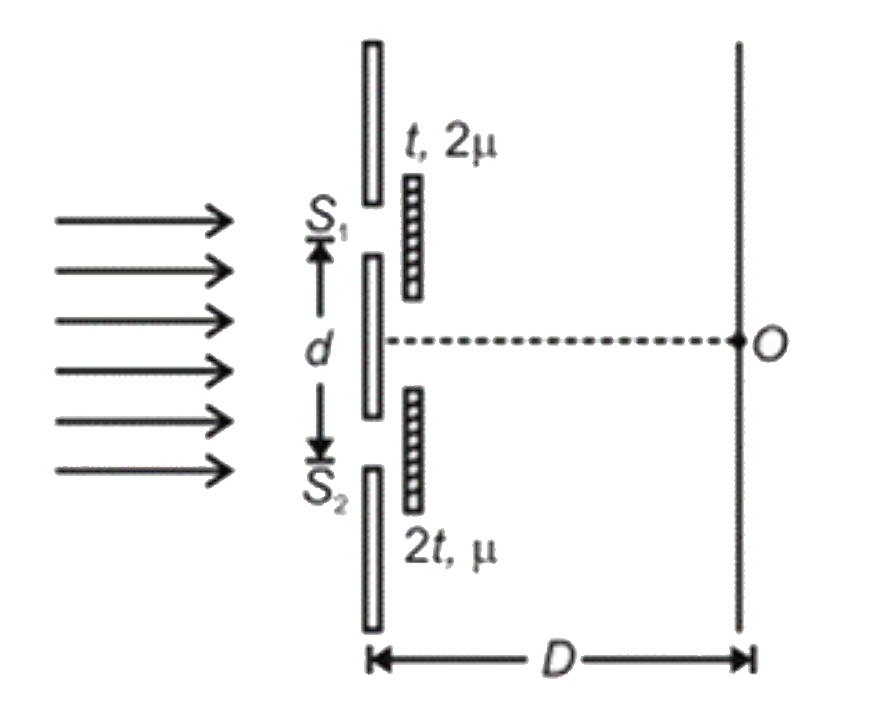
View SolutionIn the YDSE shown below, two slits are covered with thin sheets having a thickness t and 2t and refractive indices 2μ and μ respectively. The position of central maxima is shifted by a distance of N (in cm) from the central position of the screen. Find the value of N.
[d=1mm,D=1m,t=2×10−2mm,μ=32]
View SolutionA beaker contains water up to a height h1 and K oil above water up to another height h2. Find the apparent shift in the position of the bottom of the beaker when viewed from above. Refractive index of water is μ1 and that of K.oil is mu_2.
View Solution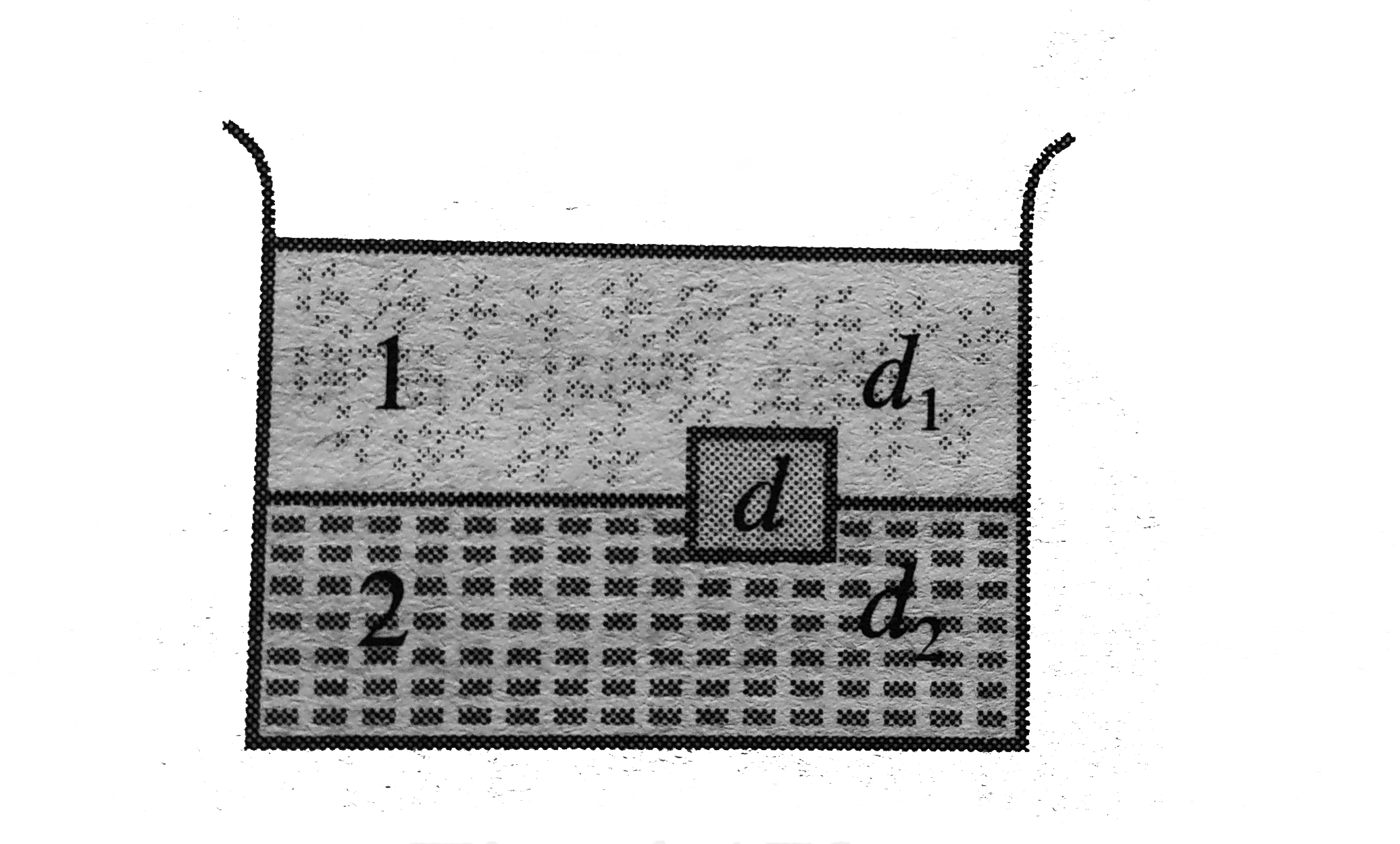
A cylinderical block of density d stays fully immersed in a beaker filled with two immiscible liquids of different densities d1 and d2 The block is in equilibrium with half of it in liquid 1 and the other half in liquid 2 as shown in the Fig. If the block is given a displacement downwards released, then neglecting friction study the following statements.
View Solution

