The mixture of a pure liquid and a solution in a along vertical column (i.e., horizontal dimensions << vertical dimensions) produces diffusion of solute particles and hence a refractive index gradient along the vertical dimension. A ray of light entering the column at right angles to the vertical is deviated from its original path. Find the deviation in travelling a horizontal distance d<<h, the height of the column.
To solve the problem of finding the deviation of a ray of light traveling through a vertical column with a refractive index gradient, we can follow these steps:
1. Understanding the Setup:
- We have a vertical column filled with a mixture of a pure liquid and a solution. The horizontal dimensions are much smaller than the vertical dimensions, leading to a refractive index gradient along the vertical direction.
- A ray of light enters the column at a right angle to the vertical.
2. Identifying the Variables:
- Let h be the height of the column.
- Let d be the horizontal distance traveled by the light ray, where d≪h.
- The refractive index is a function of the vertical position y, denoted as μ(y).
3. Applying Snell's Law:
- When the light ray enters the column, we can apply Snell's law at the interface:
μ(y)sin(θ)=μ(y+dy)sin(θ+dθ)
- Here, θ is the angle of incidence, and dθ is the small deviation angle.
4. Using Small Angle Approximations:
- For small angles, we can use the approximations:
sin(θ)≈θandcos(θ)≈1
- Thus, we can rewrite the equation as:
μ(y)θ=μ(y+dy)(θ+dθ)
5. Expanding the Right Side:
- Using Taylor expansion for μ(y+dy):
μ(y+dy)≈μ(y)+dμdydy
- Substituting this back into the equation gives:
μ(y)θ=(μ(y)+dμdydy)(θ+dθ)
6. Simplifying the Equation:
- After expanding and simplifying, we can isolate dθ:
μ(y)θ=μ(y)θ+μ(y)dθ+dμdydyθ+dμdydydθ
- Neglecting the higher-order terms, we get:
0=μ(y)dθ+dμdydyθ
7. Finding the Relationship:
- Rearranging gives:
dθ=−1μ(y)dμdydyθ
8. Relating dx and dy:
- From geometry, we know that:
dx=dytan(θ)≈dyθ(for small angles)
- Substituting this into our equation gives:
dθ=−1μ(y)dμdy⋅dxθ
9. Integrating to Find Total Deviation:
- Integrating from 0 to d for dx and from 0 to θ for dθ:
∫θ0dθ=−1μ(y)∫d0dμdydx
- This gives us the total deviation θ in terms of the gradient of the refractive index.
10. Final Result:
- The horizontal deviation D is:
D=−1μ(y)(dμdy⋅d)
- We have a vertical column filled with a mixture of a pure liquid and a solution. The horizontal dimensions are much smaller than the vertical dimensions, leading to a refractive index gradient along the vertical direction.
- A ray of light enters the column at a right angle to the vertical.
- Let h be the height of the column.
- Let d be the horizontal distance traveled by the light ray, where d≪h.
- The refractive index is a function of the vertical position y, denoted as μ(y).
- When the light ray enters the column, we can apply Snell's law at the interface:
μ(y)sin(θ)=μ(y+dy)sin(θ+dθ)
- Here, θ is the angle of incidence, and dθ is the small deviation angle.
- For small angles, we can use the approximations:
sin(θ)≈θandcos(θ)≈1
- Thus, we can rewrite the equation as:
μ(y)θ=μ(y+dy)(θ+dθ)
- Using Taylor expansion for μ(y+dy):
μ(y+dy)≈μ(y)+dμdydy
- Substituting this back into the equation gives:
μ(y)θ=(μ(y)+dμdydy)(θ+dθ)
- After expanding and simplifying, we can isolate dθ:
μ(y)θ=μ(y)θ+μ(y)dθ+dμdydyθ+dμdydydθ
- Neglecting the higher-order terms, we get:
0=μ(y)dθ+dμdydyθ
- Rearranging gives:
dθ=−1μ(y)dμdydyθ
- From geometry, we know that:
dx=dytan(θ)≈dyθ(for small angles)
- Substituting this into our equation gives:
dθ=−1μ(y)dμdy⋅dxθ
- Integrating from 0 to d for dx and from 0 to θ for dθ:
∫θ0dθ=−1μ(y)∫d0dμdydx
- This gives us the total deviation θ in terms of the gradient of the refractive index.
- The horizontal deviation D is:
D=−1μ(y)(dμdy⋅d)
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTS Book:NCERT EXEMPLAR ENGLISHChapter:RAY OPTICS AND OPTICAL INSTRUMENTS Exercise:MULTIPLE CHOICE QUESTIONS (MORE THAN ONE OPTIONS )
Explore 6 Videos
- NUCLEIBook:NCERT EXEMPLAR ENGLISHChapter:NUCLEIExercise:Long answer type question
Explore 10 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIAL,DEVICES AND SIMPLE CIRCUITBook:NCERT EXEMPLAR ENGLISHChapter:SEMICONDUCTOR ELECTRONICS: MATERIAL,DEVICES AND SIMPLE CIRCUITExercise:Multiplel choice questions
Explore 26 Videos
Similar Questions
Vertical columns of periodic table are known as
View SolutionA prism having an apex angle 4∘ and refractive index 1.5 is located in front of a vertical plane mirror as shown in figure. Through what total angle is the ray is deviated after reflection from the mirror ?
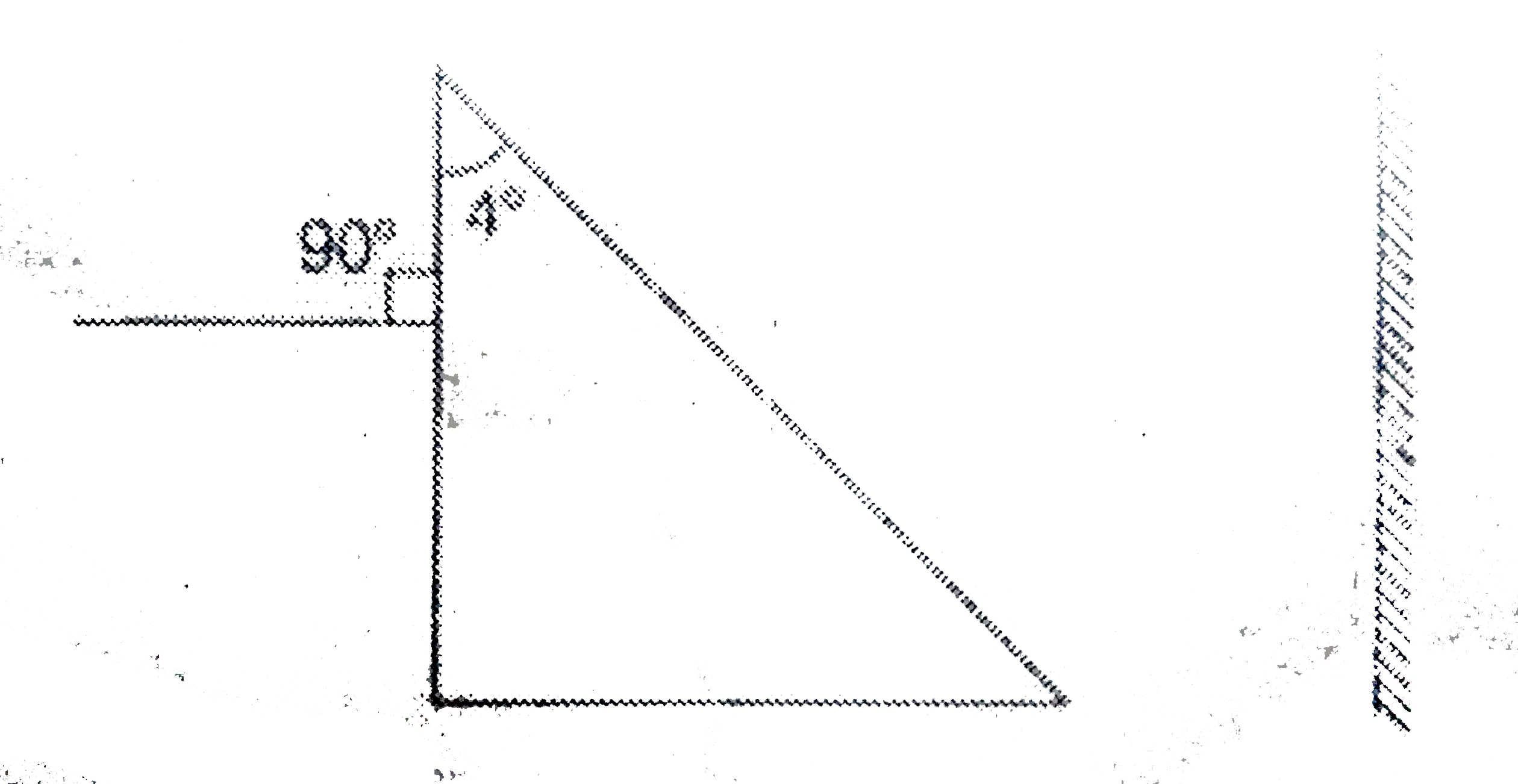
View SolutionA ray of light suffers a minimum deviation when incident on equilateral prism of refractive index √2 The angle of deviation is
View SolutionHow does the deviation produced by a prism depend on (i) the refractive index of its material, and (ii) the wavelength of incident light.
View SolutionA force of 4N is inclined at an angle of 60∘ from the vertical. Find out its components along horizontal and vertical directions.

View SolutionA force of 4N is inclined at an angle of 60∘ from the vertical. Find out its components along horizontal and vertical directions.

View SolutionThe tabular arrangment of elements in horizontal rows and vertical columns……………….
View SolutionThe angle of a prism is 60∘ and its refractive index is √2. The angle of minumum deviation sufferred by a ray of light in passing through it is
View SolutionA plane mirror lies face up, making an angle of 15∘ with the horizontal .A ray of light shines down vertically on the mirror .What is the angle of incidence? What will be the angle between the reflection ray and the horizontal?
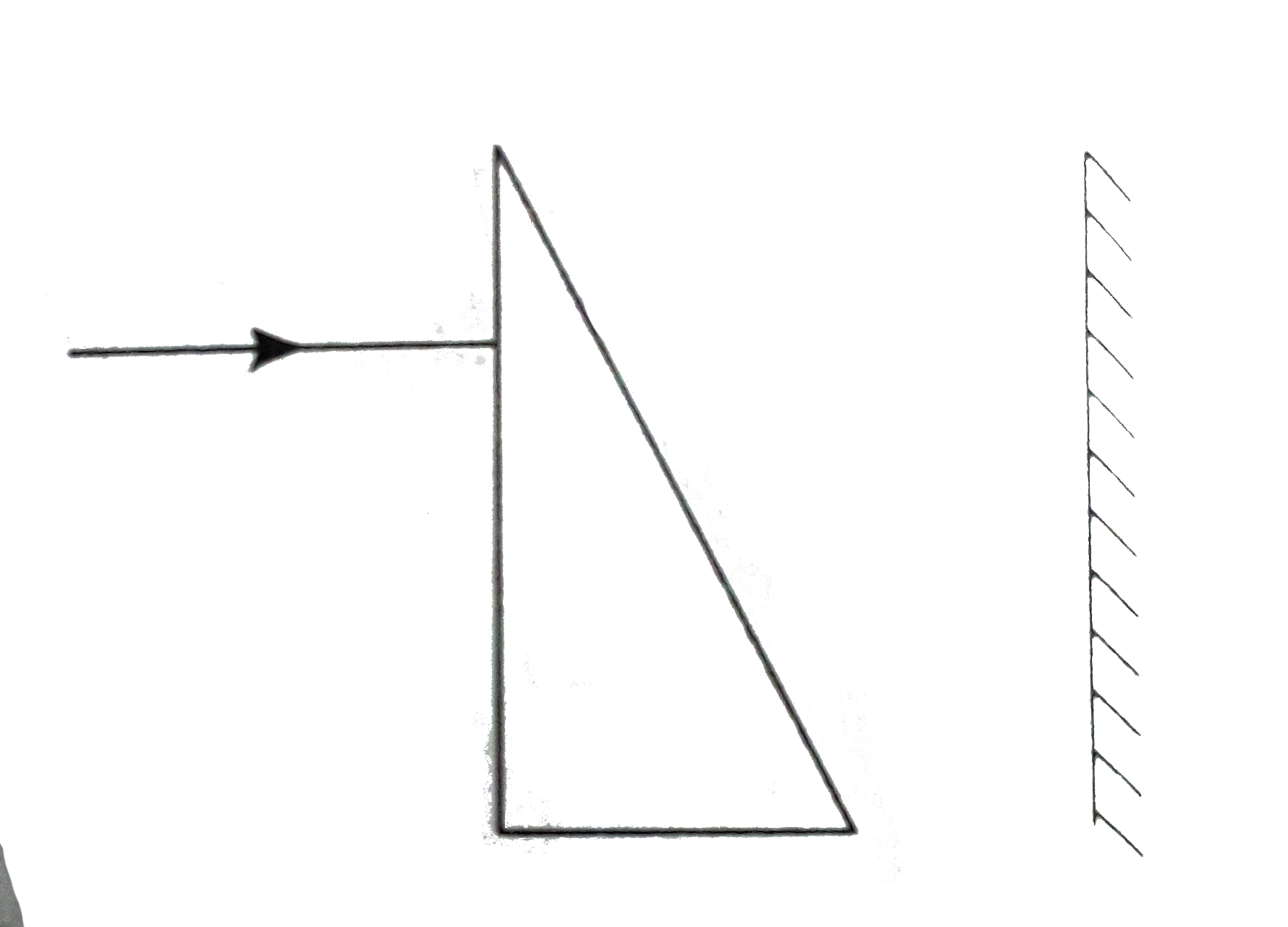
View SolutionA right-angled prism of apex angle 4∘ and refractive index 1.5 is located in front of a vertical plane mirror as shown in figure. A horizontal ray of light is falling on the prism. Find the total deviation produced in the light ray as it emerges 2nd time from the prism.
View Solution