An aeroplane when flying at a height of 4000 m from the ground passes vertically above another aeroplane at an instant when the angles of the elevation of the two planes from the same point on the ground are $60^{\circ}$ and $45^{\circ}$ respectively. Find the vertical distance between the aeroplanes at that instant.

Video Solution

Text Solution

Verified by Experts

Let O be the point of observation. Let A and B be the positions of the two planes at the given instant when A is vertically above B.
Let AB when produced meet the ground at C.
Then, $∠ C O A) = 60^{\circ}, ∠ C O B = 45^{\circ}, ∠ O C B = ∠ O C A = 90^{\circ}$ and $A C = 3000 m$ .
Let AB = x metres. Then, $B C = (3000 - x) m$ .

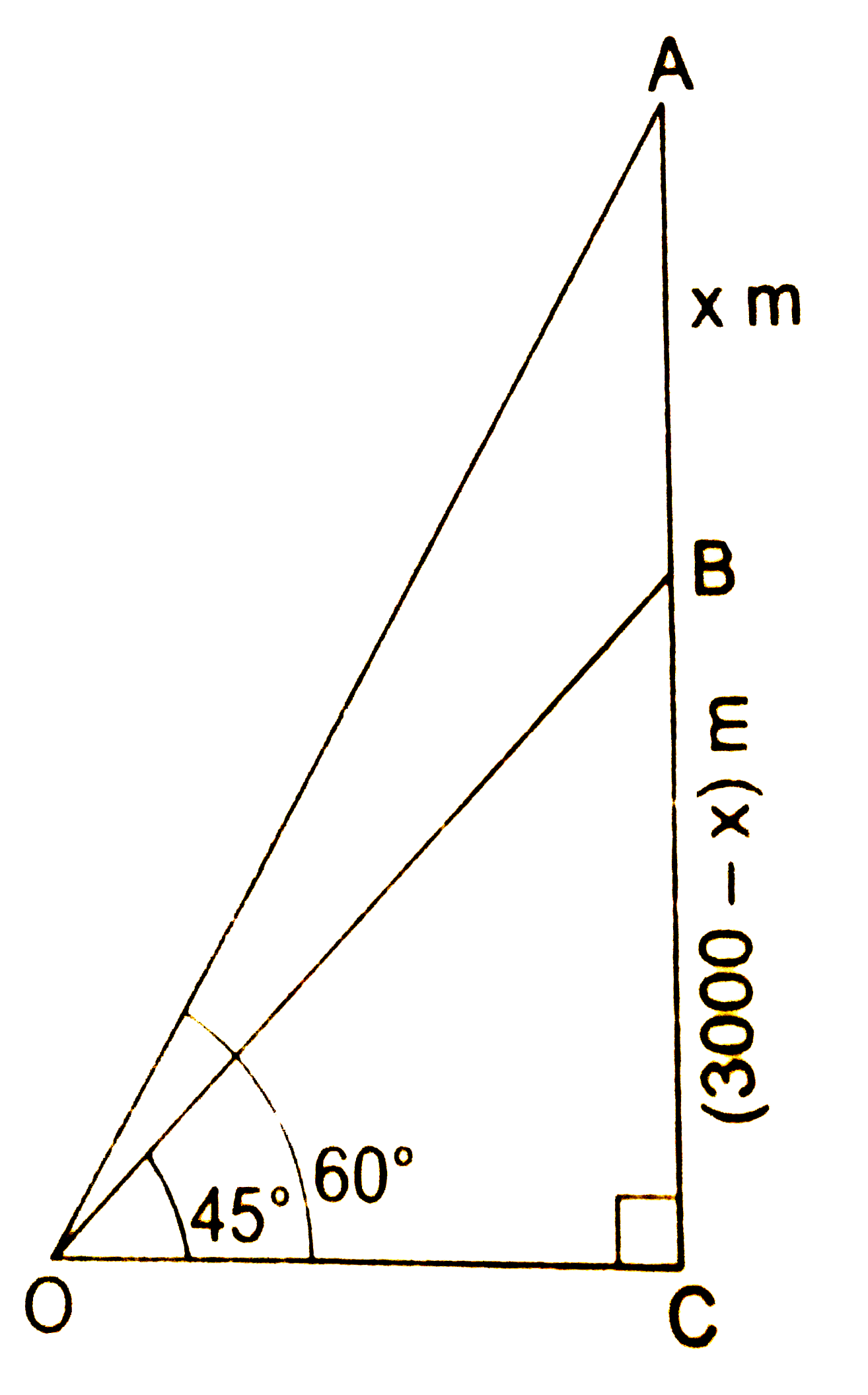
From right $Δ O C B$ , we have
$\frac{O C}{B C} = {cot 45}^{\circ} = 1 \Rightarrow \frac{O C}{(300 - x) m} = 1$
$\Rightarrow O C = (3000 - x) m . ...... (i)$
From right $Δ O C A)$ . we have
$\frac{O C}{A C} = {cot 60}^{2} = \frac{1}{\sqrt{3}} \Rightarrow \frac{O C}{3000 m} = \frac{1}{\sqrt{3}}$
$\Rightarrow O C = (\frac{3000}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}}) m = 1000 \sqrt{3} m ........ (i i)$
From (i) and (ii), we get
$(3000 - x) = 1000 \sqrt{3}$
$\Rightarrow x = (3000 - 1000 \sqrt{3}) = (3000 - 1000 \times 1.73)$
$= (3000 - 1730) = 1270$ .

Updated on:21/07/2023

An aeroplane flying at a height of 300 m above the ground passes vertically above another plane at an instant when the angles of elevation of two planes from the same point on the ground are $60^{\circ} and 45^{\circ},$ respectively. What is the height of the lower plane from the ground?
A50 m
B $\frac{100}{\sqrt{3}} m$
C $100 \sqrt{3} m$
D $150 (\sqrt{3} + 1) m$
An aeroplane flying at a height of 300 m above the ground passes vertically above another plane at an instant when the angles of elevation of two planes from the same point on the ground are $60^{\circ} and 45^{\circ},$ respectively. What is the height of the lower plane from the ground?
A $100 \sqrt{3}$
B $100 / \sqrt{3}$
C50
D $150 (\sqrt{3} + 1)$
An aeroplane at a height of 600 m passes vertically above another aeroplane at an instant when their angles of elevation at the same observing point are $60^{\circ} and 45^{\circ}$ respectively. How many metres higher is the one from the other?
A286.53 m
B274.53 m
C253.58 m
D263.83 m