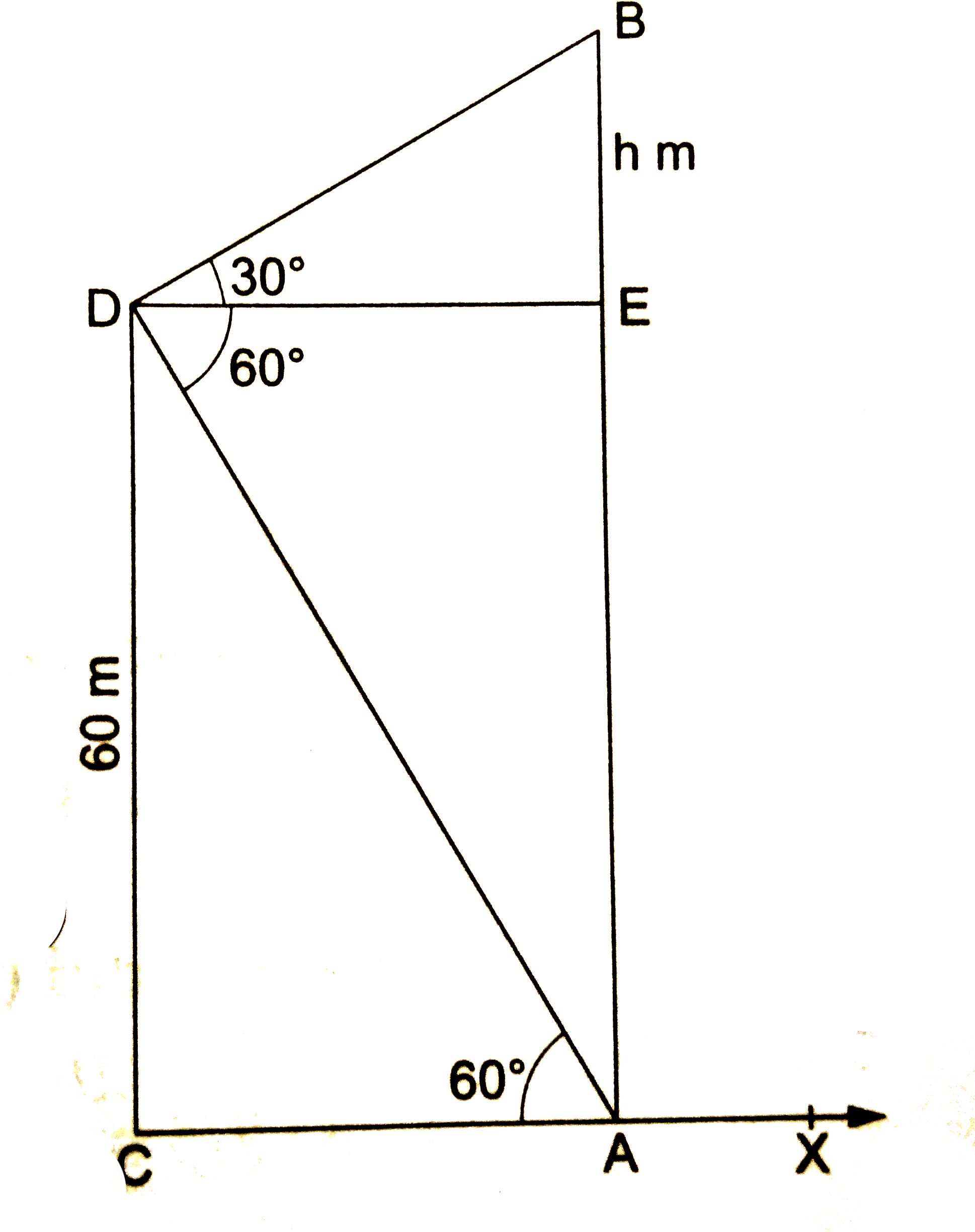
From the top of a building 60m high the angles of depression of the top and the bottom of a tower are observed to be $30^{0} a n d 60^{0}$ . Find the height of the tower.

Video Solution

Text Solution

Verified by Experts

Let AB be the tower and the CD be the building. Then, $C D = 60 m$ . Ket $C A X$ be the horizontal ground.
Draw $D E ⊥ A B$ . Now , $∠ E D B = 30^{\circ}$ and $∠ C A D = ∠ A D E = 60^{\circ}$ .
Also, $A E = C D = 60 m$
Let $B E = h$ metres.
From right $Δ A C D$ , we have
$\frac{C A}{C D} = {cot 60}^{\circ} = \frac{1}{\sqrt{3}}$ .
$\Rightarrow \frac{C A}{60 m} = \frac{1}{\sqrt{3}} \Rightarrow C A = \frac{60}{\sqrt{3}} ......... (i)$
From right $Δ E D B$ , we have
$\frac{D E}{B E} = {cot 30}^{\circ} = \sqrt{3} \Rightarrow \frac{D E}{h m} = \sqrt{3}$
$\Rightarrow D E = h \sqrt{3} m ...... (i i)$
But , $C A = D E$ .
$∴$ from (i) and (ii), we get
$\frac{60}{\sqrt{3}} = h \sqrt{3} \Leftrightarrow 3 h = 60 \Rightarrow h = 20$ .
Hence, the difference between the heights of the towards and building is $20$ metres.
From (i), we get $C A = (\frac{60}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}}) m = 20 \sqrt{3}$ metres.
Hence the distance between the tower and the building is $20 \sqrt{3} m$ .

Updated on:21/07/2023

From the top of a building 60 metre high ,the angles of depression of the top and bottom of a tower are observed to be $30^{\circ} and 60^{\circ}$ respectively.The height of the tower in metre is :
Aa.40
Bb.45
Cc.50
Dd.55
From the top of a cliff 90 m high, the angles of depression of the top and bottom of a tower are observed to be $30^{\circ}$ and $60^{\circ}$ respectively. The height of the tower is :
A45 m
B60 m
C75 m
D30 m
From the top of the cliff 150 m high, the angles of depression of the top and bottom of a tower are observed to be 30° and 60°, respectively. The height of the tower is
A100 m
B $50 \sqrt{3} m$
C $133 \frac{1}{3}$
D $100 (\sqrt{3} - 1)$