The shadow of a tower standing on a level plane is found to be 30 m longer when sun's elevation is 30∘ than when it is 60∘. Find the height of the tower.
28.98 m
25.98m
32.98 m
22.98 m
The correct Answer is:B
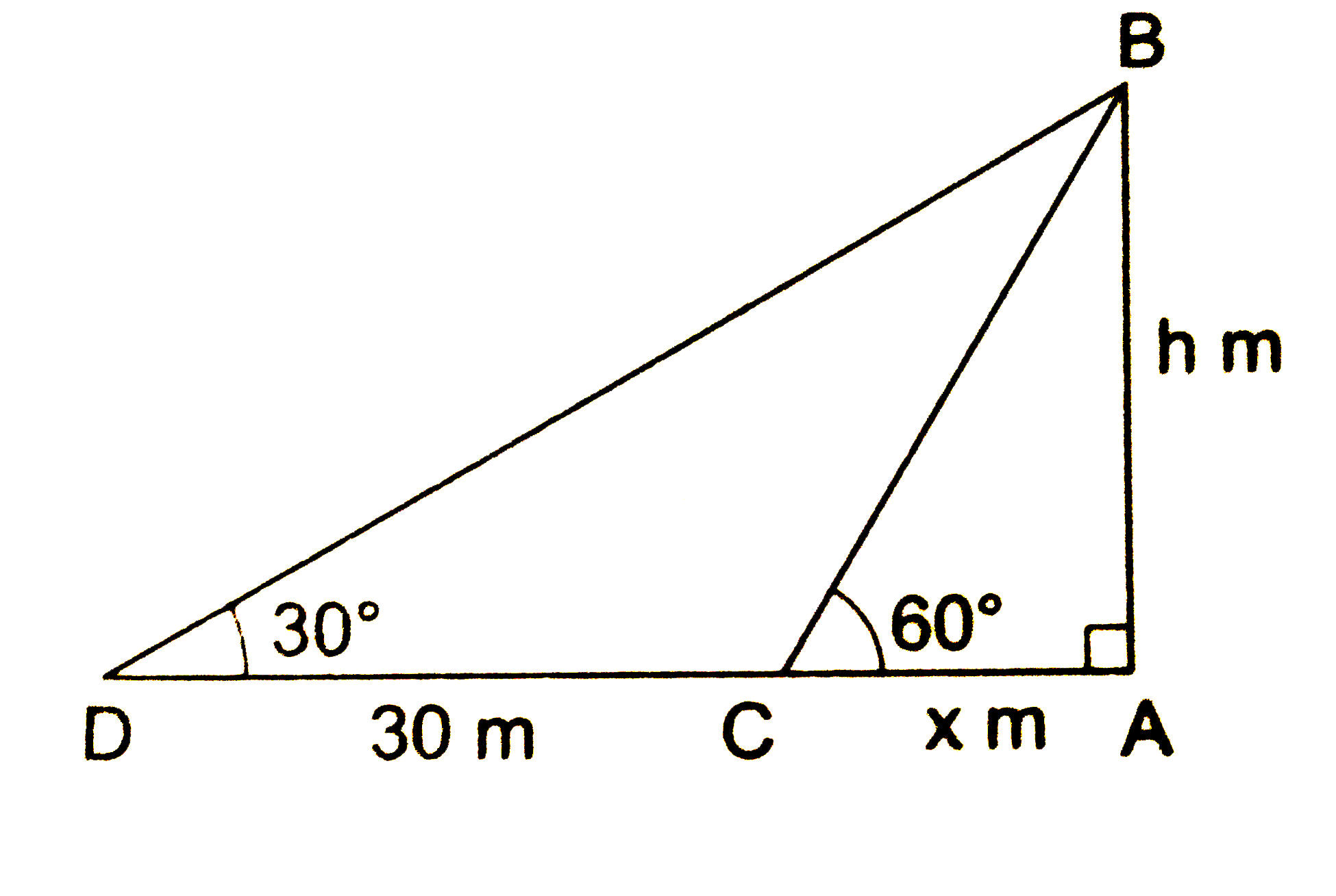
Let B be the tower and let AC and AD be the lengths of its shadows when ∠ACB)=60∘ and ∠ADB)=30∘
Let AB=h metres, and AC=x metres.
From right ΔCAB , we have
ACAB=cot60∘=1√3⇒xh=1√3.
⇒x=(h×1√3)=h√3......(i)
From right, ΔDAB , we have
ADAB=cot30∘=√3⇒x+30h=√3.
⇒x=(√3h−30)
Equating the values of x from (i) and (ii), we get
h√3=(√3h−30)⇒h=3h−30√3
⇒2h=30√30⇒h=15√3=(15×1.732)=25.98.
Hence, the height of the tower is 25.98m.
 .
.
Topper's Solved these Questions
- HEIGHTS AND DISTANCESBOOK - RS AGGARWALCHAPTER - HEIGHTS AND DISTANCESEXERCISE - Exercise 14
38 Videos
- HEIGHTS AND DISTANCESBOOK - RS AGGARWALCHAPTER - HEIGHTS AND DISTANCESEXERCISE - Multiple Choice Questions (Mcq)
25 Videos
- COORDINATE GEOMETRYBOOK - RS AGGARWALCHAPTER - COORDINATE GEOMETRYEXERCISE - Multiple Choice Questions (Mcq)
34 Videos
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - RS AGGARWALCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - QUESTION
2 Videos
Similar Questions
The shadow of a tower standing on a level plane is found to be 50 m longer when when sun's elevation is 30∘ than when it is 60∘. Find the height of the tower.
View SolutionThe shadow of a tower standing on a level plane is found to be 50 m longer when the Sun's elevation is 30∘ than when it is 60∘ What is the height of the tower ?
View Solution
Knowledge Check
The shadow of a tower standing on a level plane is found to be 50 m longer when the Sun's elevation is 30∘ than when it is 60∘. The height of the tower is
AA. 25 mBB. 25√3mCC. 50mDD. None of theseThe shadow of a tower standing on a level plane is found to be 50 m longer when the Sun's elevation is 30∘ than when it is 60∘ What is the height of the tower ?
A10 mB10√3mC20 mD20√3mThe shadow of a tower standing on a level plane is found to be 50m longer when sun's altitude is 30∘ then when it is 60∘. Find the height of tower.
A40.3mB43.3mC43.9mD46.3m
The shadow of a tower standing on a level plane is found to be 50 m longer when the Sun's elevation is 30∘ than when it is 60∘. The height of the tower is
View SolutionThe shadow of a tower standing on a level ground is found to be 20 m longer when the sun's altitude is 45∘ than when it is 60∘ .Find the height of the tower .
View SolutionThe shadow of a tower standing on a level ground is found to be 60 metres longer when the sun's altitude is 30∘ than when it is 45∘. The height of the tower is____
View SolutionThe shadow of a tower standing on a level plane is found to be 40 m longer when the sun 's altitude is 45∘ , than when it is 60∘ . The height of the tower is
View SolutionThe shadow of a tower standing on a level plane is found to be 50m longer when sun's altitude is 30∘ then when it is 60∘. Find the height of tower.
View Solution
