Find the area bounded by the curves x2+y2=25,4y=∣∣4−x2∣∣, and x=0 above the x-axis.
Video Solution
Text Solution
The correct Answer is:[4+25sin−1(45)] sq units
Given curves , x2+y2=25,4y=∣∣4−x2∣∣ could be sketched as below, whose points of intersection are
x2+(4−x2)216=25
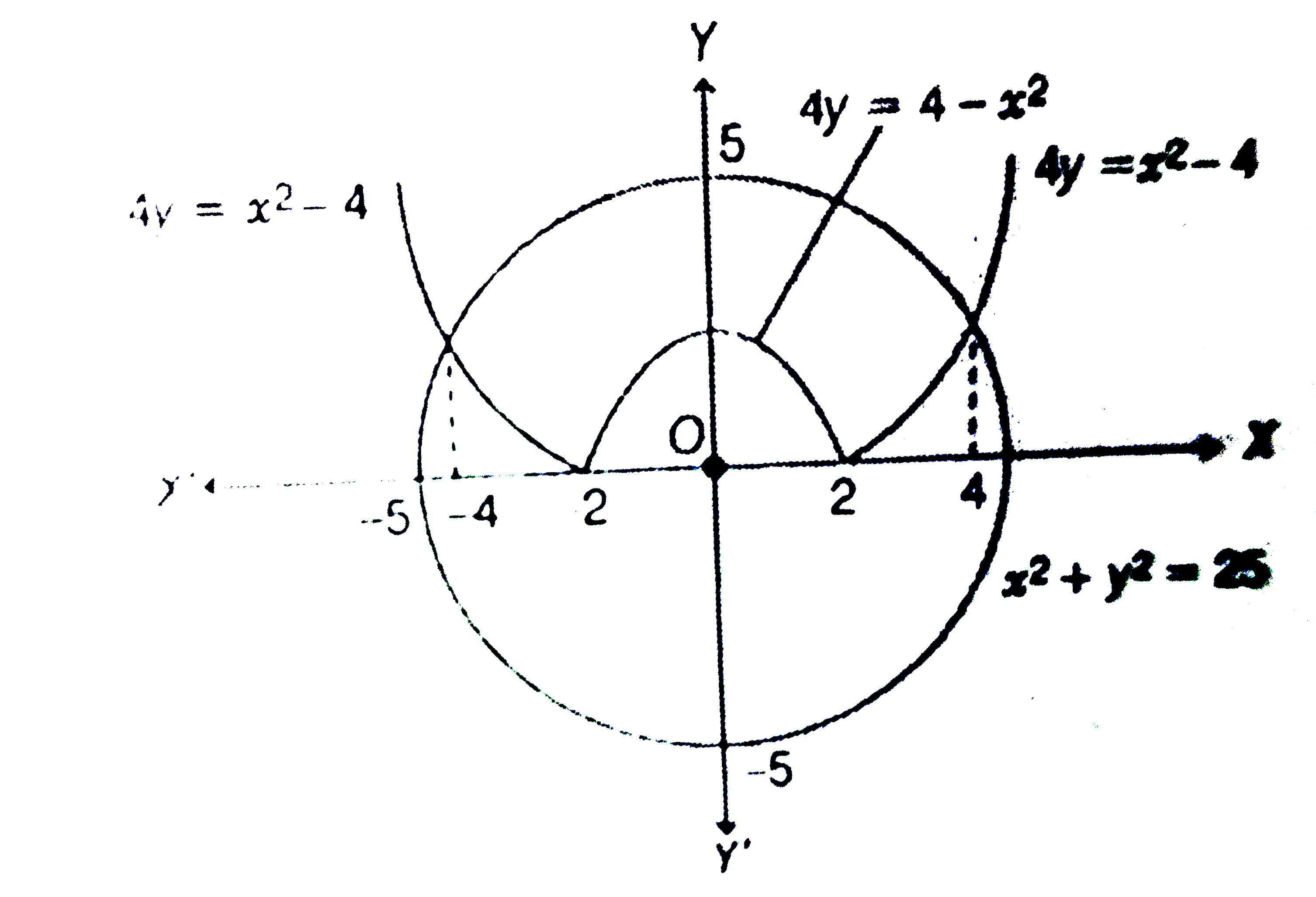
⇒(x2+24)(x2−16)=0
⇒x=±4
∴ Required area =2[∫40√25−x2dx−∫20(4−x24)dx−∫42(x2−44)dx]
=2[[x2√25−x2+252sin−1(x5)]40−14[4x−x33]20−14[x33−4x]42]
=2[[6+252sin−1(45)]14[8−83]−14[(643−16)−(83−8)]]
=2[6+252sin−1(45)−43−43−43]
=[4+25sin−1(45)] sq units
|
Topper's Solved these Questions
- AREABOOK - IIT JEE PREVIOUS YEARCHAPTER - AREAEXERCISE - AREA USING INTEGRATION
55 Videos
- APPLICATION OF DERIVATIVES BOOK - IIT JEE PREVIOUS YEARCHAPTER - APPLICATION OF DERIVATIVES EXERCISE - MAXIMA AND MINIMA ( INTEGER ANSWER TYPE QUESTIONS)
8 Videos
- BINOMIAL THEOREMBOOK - IIT JEE PREVIOUS YEARCHAPTER - BINOMIAL THEOREMEXERCISE - Topic 2 Properties of Binomial Coefficent Objective Questions I (Only one correct option) (Analytical & Descriptive Questions )
8 Videos
Similar Questions
Find the area bounded by the curves x2+y2=25,4y=∣∣4−x2∣∣, and x=0 above the x-axis.
View SolutionFind the area bounded by the curves x+2|y|=1 and x=0 .
View SolutionFind the area bounded by the curves 4y=∣∣4−x2∣∣,y=7−|x|
View SolutionFind the area bounded by the curves : y2=4x,y2=x,x=1andx=4
View SolutionFind the area bounded by the curve y=−3|x|+2 and x-axis
View Solution.Find the area bounded between the curves y2=4x,y2=4(4−x)
View SolutionFind the area bounded by the curve 4y2=9xand3x2=16y
View SolutionFind the area bounded by the curves x2=y, x2=−y and y2=4x−3
View SolutionFind the area bounded by the curve y2=4ax and x2+y2−2ax=0 above the x-axis.
View SolutionFind the area bounded by the curves x2+y2=4 and x2+y2=4x
View Solution