Question

Let $f\left(x\right)$
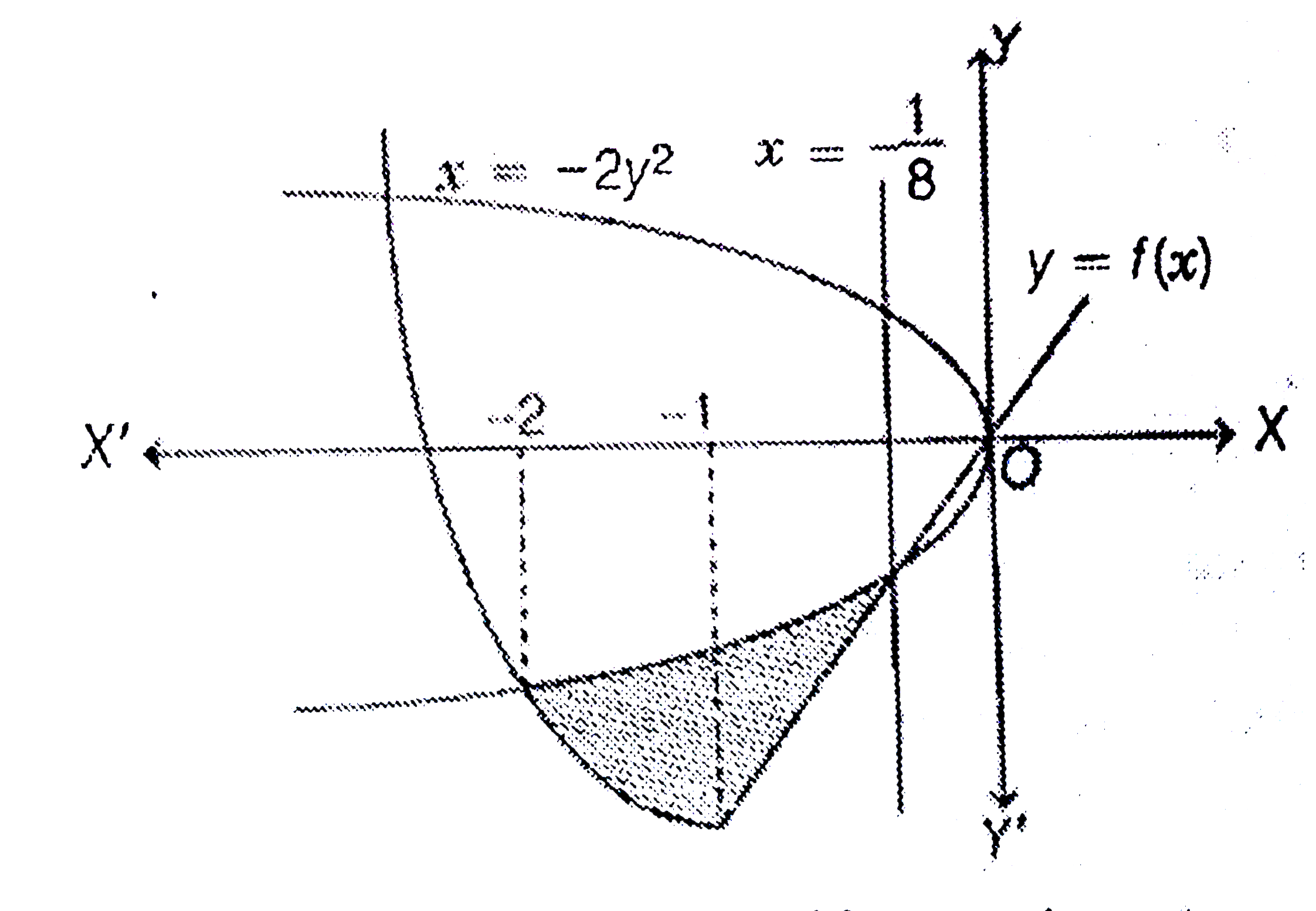
be continuous function given by
$f\left(x\right)=\{2x,\left|x\right|\le 1x^2+ax+b,\left|x\right|>1\}\stackrel{.}{}$

Find the area of the region in the third quadrant bounded by the curves
$x=-2y^2$ and $y=f\left(x\right)$
lying on the left of the line $8x+1=0.$

Answer

`((761)/(192))` sq units

Explanation

Given, `f(x)={(2x",",|x|le1),(x^(2)+ax+b",",|x| gt1):}`

`rArr f(x)={(x^(2)+ax+b",",if x lt -1),(2x",", if -1 le x lt 1),(x^(2)+ax+b",", if x ge 1):}`
f is continuous on R, so f is continuous at -1 and 1.
`lim_(xto -1^(-))f(x)=lim_(xto -1^(+))f(x) = f(-1)`
` and lim_(xto -1^(-)) f(x)=lim_(xto 1^(+))f(x)=f(1)`
`rArr 1-a + b = -2 and 2=1+a+b`
`rArr a-b= 3 and a+b=1`
`therefore a =2, b= -1`
Hence, `f(x)={(x^(2)+2x-1",",if x lt -1),(2x",", if -1 le x lt 1),(x^(2)+2x-1",", if x ge 1):}`
Next, we have to find the points `x= -2y^(2) and y=f(x).`
The point of intersection is (-2, -1).
`therefore " Required area " =int_(-2)^(-1//8)[sqrt((-x)/(2))-f(x)]dx`
`=int_(-2)^(-1//8) sqrt((-x)/(2))dx-int_(-2)^(-1)(x^(2)+2x-1)dx-int_(-1)^(-1//8) 2x dx`
`= - (2)/(3sqrt(2))[(-x)^(3//2)]_(-2)^(-1//8)-[((x^(3))/(3)+x^(2)-x)]_(-2)^(-1)-[x^(2)]_(-1)^(-1//8)`
`= -(2)/(3sqrt(2))[((1)/(8))^(3//2)-2^(3//2)] -(-(1)/(3)+1+1)+(-(8)/(3)+4+2)-[(1)/(64)-1]`
`=(sqrt(2))/(3) [2sqrt(2)-2^(-9//2)]+(5)/(3)+(63)/(64)`
`=(63)/(16xx3)+(509)/(64xx3)=(761)/(192)` sq units