The height of a right circular cylinder of maxium volume inscirbed in a sphere of radius 3 is

A

√6

B

2√3

C

√3

D

23√3

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:B

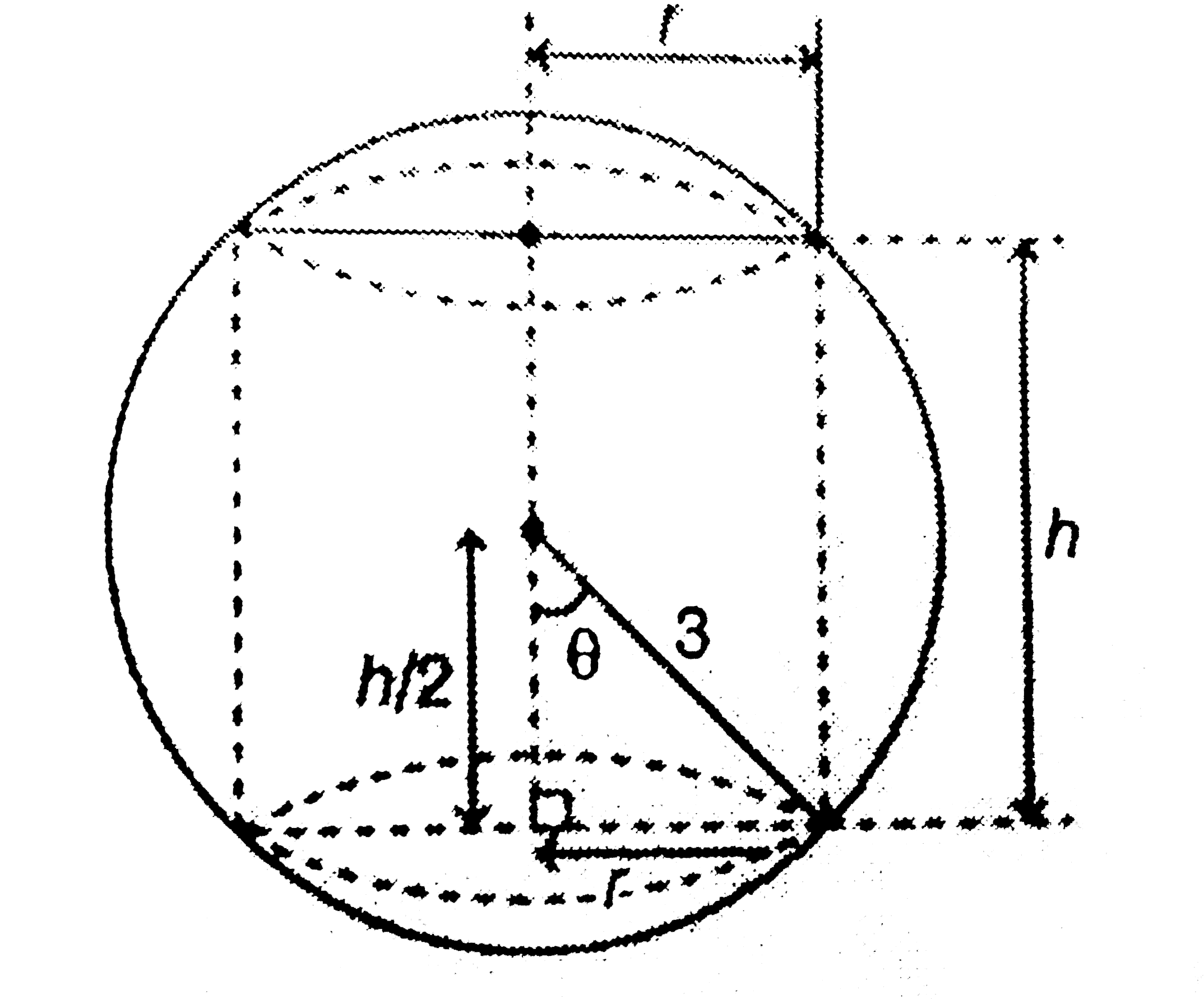
Let a sphere of radius, 3, which inscribed a right circular cylinder having radius r and height is h, so
From the figure, h2=3cosθ
⇒h=6cosθ
and r=3sinθ ...(i)
ocr_image
For maxima or minima, dVdthη=0
⇒54π[2sinθcos2θ−sin3θ]=0
⇒sinθ[2cos2θ−sin2θ]=0
⇒tan2θ=2[∴θ∈(0,π2)]
⇒tanθ=√2
⇒sinθ=√23andcosθ=1√3 ...(ii)
From Eqs.(i) and (ii), we get
h=61√3=2√3

Updated on:21/07/2023
|
Class 12MATHSAPPLICATION OF DERIVATIVES

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The height of the cylinder of max. volume that can be inscribed in a sphere of radius a is

    A2a√3
    Ba√2
    C5a4
    DNone

  • The height of the cylinder of the greatest volume that can be inscribed in a sphere of radius 3 is

    A3√3
    B2√3
    C√3
    D√2

  • Each of the radius of the base and the height of a right circular cylinder is increased by 10%. The volume of the cylinder is increased by

    A3.31%
    B14.5%
    C33.1%
    D19.5%