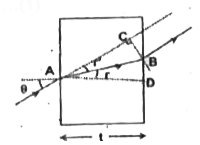
A ray of light strikes a glass slab of thickness t.
(i) Prove that it emerges on the opposite face, parallel to the initial ray.
(ii) (ii) Prove that the value of deflection of beam which passed through the plate is :
$t {\sin i}_{1} [1 - \sqrt{(\frac{1 - \sin^{2} i_{1}}{._{a} μ_{g}^{2} - \sin^{1} i_{1}})}]$
(iii) Prove that for a small angle of incidence $i_{1}$ , the internal shift x is given by
$x = t i_{1} (1 - \frac{1}{._{a} μ_{g}})$
where $._{a} μ_{g}$ is the refractive index of glass with respect to air.

Video Solution

Text Solution

Verified by Experts

Lateral shift, $y = B C = A B {\sin r}^{.} = A B \sin (θ - r)$
$\Rightarrow y = \frac{t}{\cos r} (\sin θ - \cos θ \sin r) = t (\sin θ - \cos θ \tan r)$
$t \sin θ (1 - \cot θ \tan r)$ …(2)
where AB= t/cosr and r= angle of refraction given by using snell.s law,
$\frac{\sin θ}{\sin r} = n$
$\Rightarrow \sin r = \frac{\sin θ}{n}$ & cosr in (1) we obtain
$y = t \sin θ [1 - \cot θ \frac{\frac{\sin θ}{n}}{\frac{\sqrt{n^{2} - \sin^{2} θ}}{n}}]$
$= t \sin θ [1 - \sqrt{\frac{1 - \sin^{2} θ}{n^{2} - \sin^{2} θ}}]$
(b) If $θ$ is small, $\sin^{2} θ$ can be ignored in comparison to 1, $n^{2}$
$\Rightarrow y ≅ t θ [1 - \sqrt{\frac{1}{n^{2}}}]$
$\Rightarrow y = t θ [1 - \frac{1}{n}]$

Updated on:21/07/2023

A ray of light strikes a glass slab of thickness t. It emergens on the opposite face, parallel to the incident ray but laterally displaced. The lateral displacement, $Δ x$ is
A $Δ x = 0$
B $Δ x = t sin ( I - r) cos r$
C $Δ x = \frac{t sin I}{cos r}$
D $Δ x = \frac{t sin ( I - r)}{cos r}$
A ray of light strikes a glass slab (R.l. = $μ$ ) of finite thickness t. The emerging light is
Aparallel to. the incident ray
Bperpendicular to the incident ray
Crotates by an angle $[{sin}^{- 1} (\frac{1}{μ})]$
Dtotally internally reflected
A ray of light is incident on a glass slab of thickness t, at an angle i,r is the angle of refraction in the glass slab. Distance travelled in the glass slab is
A $t cos r$
B $a n r$
C $\frac{t}{cos r}$
D $\frac{t}{sin r}$