Find the centre of mass of the shaded portion of a disc.
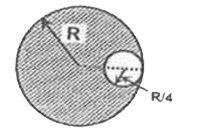
Xcm=m2(0)−m1(3R4)m2−m1=−3R4(m2m1−1)
where m2m1=(πR2)ρπ(R4)2ρ=16
⇒Xcm=−3R4(16−1)=−R20
Note : Negative mass is taken because the mass is removed.
Topper's Solved these Questions
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - SOLVED PROBLEMS (OBJECTIVE)
18 Videos
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - SOLVED PROBLEMS (OBJECTIVE) ASSERTION REASONING TYPE
1 Video
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - (NUMERICAL BASED QUESTIONS)
4 Videos
- AC CIRCUITSBOOK - FIITJEECHAPTER - AC CIRCUITSEXERCISE - ASSERTION REASONING TYPE
1 Video
- CURRENT ELECTRICITYBOOK - FIITJEECHAPTER - CURRENT ELECTRICITYEXERCISE - Comprehension -4
3 Videos
Similar Questions
Find the centre of mass xCM of the shaded portion of the disc of radius R=24cm.

View SolutionFrom a uniform circular disc of radius R, a circular disc of radius R/6 and having centre at a distance R/2 from the centre of the disc is removed. Determine the centre of mass of remaining portion of the disc.
View Solution
Knowledge Check
The centre of mass of the shaded portion of the disc is: ( The mass is uniformly distributed in the shaded portion).
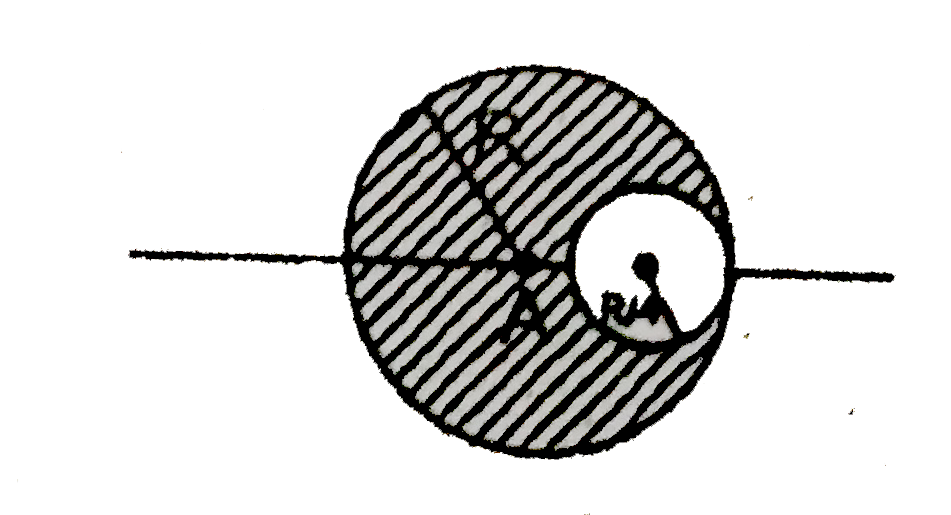 AR20 to the left of ABR12 to the left of ACR20 to the right of ADR12 to the right of A
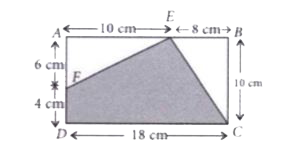
AR20 to the left of ABR12 to the left of ACR20 to the right of ADR12 to the right of AFind the perimeter and area of the shaded portion of the adjoining diagram:
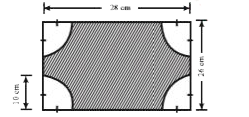 A90.8cm,414cm2B181.6cm,423.7cm2C90.8cm,827.4cm2D181.6cm,827.4cm2
A90.8cm,414cm2B181.6cm,423.7cm2C90.8cm,827.4cm2D181.6cm,827.4cm2
Find the area of the shaded portion.
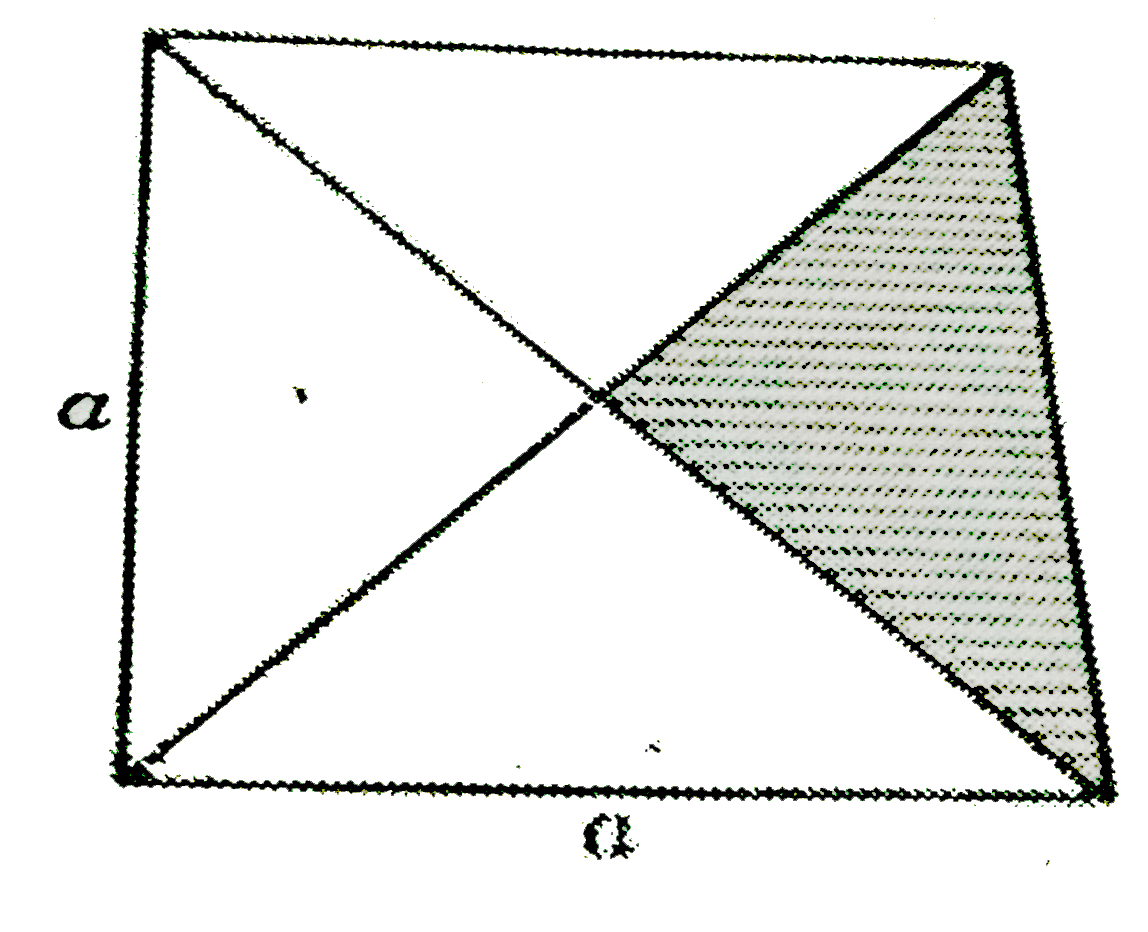
View SolutionFrom a square sheet of uniform density , a portion a removed shown shaded in fig. Find the centre of msas of the remaining portion is the side of the square is α
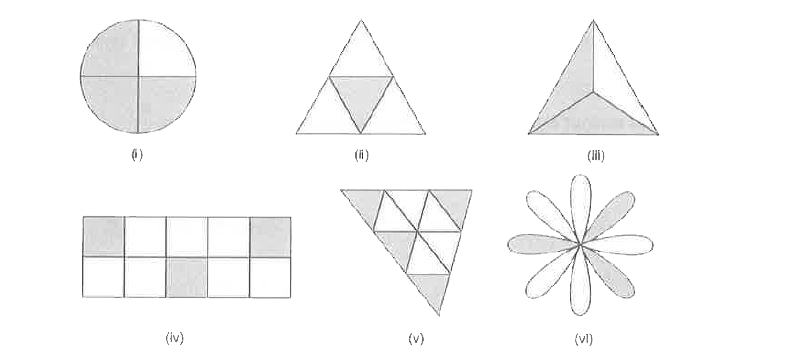
View Solution(i) Write the fraction of the shaded portion:
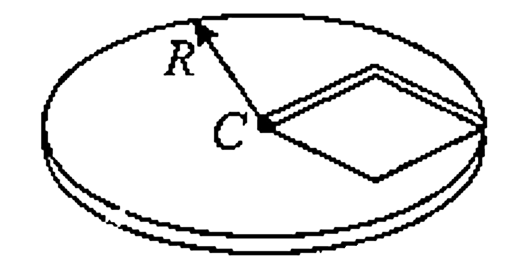
View SolutionA circular disc of radius R4 is cut from a uniform circular disc of radius R.
The centre of cut portion is at a distance of R2 from the centre of the disc from which it is removed. Calculate the centre of mass of remaining portion of the disc.
View SolutionFind the centre of mass of the disc shown in figure. . A square is removed from the disc as shown
View SolutionFind the centre of mass of a thin, uniform disc of radius R from which a small concentric disc of radius r is cut.
View Solution
